Sangaku with Versines
The following Sangaku has been written in 1825 in the Tokyo prefecture but has since disappeared. It relates the rarely used nowadays versine quantities and the distance from a vertex to the inclircle.
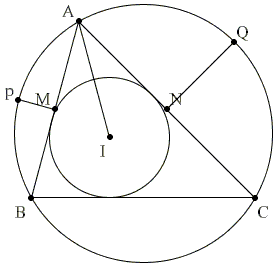
Assuming that MP and NQ are the perpendicular bisectors of AB and AC, respectively, we have
| (1) | 4·MP·NQ = AI2. |
It sheds some light on the appearance of the inradius in a different formula from a related problem.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny

Assuming that MP and NQ are the perpendicular bisectors of AB and AC, respectively, we have
| (1) | 4·MP·NQ = AI2. |
Solution

Let A', B', C' be the points of tangency of the incircle with BC, AC, and AB, respectively. Introduce
a' = AB' = AC' = p - a,
b' = BA' = BC' = p - b,
c' = CA' = CB' = p - c,
where p is the semiperimeter p = (a + b + c)/2. Note that
Lemma 1
Proof
By Heron's formula, area S can be obtained from
S2 = pa'b'c',
but also S = rp, which gives (2).
Lemma 2
Proof
We use a known relation between the angles in a triangle
| (4) | cot(A/2) + cot(B/2) + cot(C/2) = cot(A/2)·cot(B/2)·cot(C/2). |
Observe that ![]() AQN = (π - B)/2, so that
AQN = (π - B)/2, so that
a'/r + (a' + c')/(2d1) + c'/r = (a'/r)((a' + c')/(2d1))(c'/r),
which leads to
1/r + 1/(2d1) = a'c'/(2d1r2).
So that we get a'c' = r2 + 2rd1, the first equation in (3). The second one is obtained similarly.
Proof of (1)
Add the equations in (3):
a'(b' + c') = 2r2 + 2r(d1 + d2),
and multiplying the equations in (3) we get
a'b'c' = 2r2(r + 2d1)(r + 2d2) / a'.
Lemma 1 in the form r(a' + b' + c') = a'b'c' gives
r2(a' + (2r2 + 2r(2d1 + 2d2) / a')) = r2(r + 2d1)(r + 2d2)/ a',
which leads to (a')2 + r2 = 4d1d2, but
Remark
If we use k, l, m (as in a related problem) for MP, NQ and the altitude of the circular segment cut off by BC, then the product of the three identities implied by (1) shows that
klm = 8·AI·BI·CI.
References
H. Fukagawa, D. Pedoe, Japanese Temple Geometry Problems, The Charles Babbage Research Center, Winnipeg, 1989, pp. 101-102
Write to:
Charles Babbage Research Center
P.O. Box 272, St. Norbert Postal Station
Winnipeg, MB
Canada R3V 1L6
Sangaku
- Sangaku: Reflections on the Phenomenon
- Critique of My View and a Response
- 1 + 27 = 12 + 16 Sangaku
- 3-4-5 Triangle by a Kid
- 7 = 2 + 5 Sangaku
- A 49th Degree Challenge
- A Geometric Mean Sangaku
- A Hard but Important Sangaku
- A Restored Sangaku Problem
- A Sangaku: Two Unrelated Circles
- A Sangaku by a Teen
- A Sangaku Follow-Up on an Archimedes' Lemma
- A Sangaku with an Egyptian Attachment
- A Sangaku with Many Circles and Some
- A Sushi Morsel
- An Old Japanese Theorem
- Archimedes Twins in the Edo Period
- Arithmetic Mean Sangaku
- Bottema Shatters Japan's Seclusion
- Chain of Circles on a Chord
- Circles and Semicircles in Rectangle
- Circles in a Circular Segment
- Circles Lined on the Legs of a Right Triangle
- Equal Incircles Theorem
- Equilateral Triangle, Straight Line and Tangent Circles
- Equilateral Triangles and Incircles in a Square
- Five Incircles in a Square
- Four Hinged Squares
- Four Incircles in Equilateral Triangle
- Gion Shrine Problem
- Harmonic Mean Sangaku
- Heron's Problem
- In the Wasan Spirit
- Incenters in Cyclic Quadrilateral
- Japanese Art and Mathematics
- Malfatti's Problem
- Maximal Properties of the Pythagorean Relation
- Neuberg Sangaku
- Out of Pentagon Sangaku
- Peacock Tail Sangaku
- Pentagon Proportions Sangaku
- Proportions in Square
- Pythagoras and Vecten Break Japan's Isolation
- Radius of a Circle by Paper Folding
- Review of Sacred Mathematics
- Sangaku à la V. Thebault
- Sangaku and The Egyptian Triangle
- Sangaku in a Square
- Sangaku Iterations, Is it Wasan?
- Sangaku with 8 Circles
- Sangaku with Angle between a Tangent and a Chord
- Sangaku with Quadratic Optimization
- Sangaku with Three Mixtilinear Circles
- Sangaku with Versines
- Sangakus with a Mixtilinear Circle
- Sequences of Touching Circles
- Square and Circle in a Gothic Cupola
- Steiner's Sangaku
- Tangent Circles and an Isosceles Triangle
- The Squinting Eyes Theorem
- Three Incircles In a Right Triangle
- Three Squares and Two Ellipses
- Three Tangent Circles Sangaku
- Triangles, Squares and Areas from Temple Geometry
- Two Arbelos, Two Chains
- Two Circles in an Angle
- Two Sangaku with Equal Incircles
- Another Sangaku in Square
- Sangaku via Peru
- FJG Capitan's Sangaku
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny
73209450
