Heron's Problem: What is it?
A Mathematical Droodle

Explanation

|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener|

Copyright © 1996-2018 Alexander Bogomolny

Heron's Problem

This one is a basic optimization problem. It's quite famous, being discussed in Heron's Catoptrica (On Mirrors from the Greek word Katoptron Catoptron = Mirror) that, in all likelihood, saw the light of day some 2000 years ago.

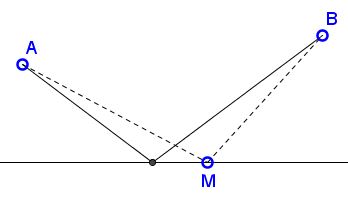
Think of l as a mirror. Heron posed that the shortest distance between A and B via l is exactly the path traversed by a ray of light emitted from A and observed at B. From here he deduced that when light is reflected in a mirror the angle of incidence f_i is equal to the angle of reflection f_r, where the angles at hand are formed by AM and MB with the perpendicular to l at M.

Why so?

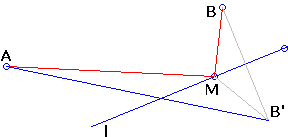
Let B' be the symmetric image of B in l, such that BB' is perpendicular to l and is divided by the latter in halves. MB = MB', for any point M on l. Therefore, AM + MB = AM + MB'. By the triangle inequality ( ΔAMB'),

AM + MB' ≥ AB'.

with the equality reached only when M lies on AB', in which case clearly the two angles coincide.

Heron's problem has applications to curved surfaces, ellipse for one.

Note: the problem has been known in Japan. It appeared on a now lost sangaku tablet from 1830 written in the Yamagata prefecture [Fukagawa and Pedoe, problem 4.1.1]. The Japanese variant underscored the computational aspects of the solution:

P is a variable point in the given segment AB, and C is a fixed point on the perpendicular AC to the line AB, and D is fixed, on the same side of AB as C, and lies on the perpendicular BD to AB. AC = c, BD = d, and AB = k. Find AP = p such that CP + PD is a minimum when P moves on AB.

It is not difficult to surmise the solution from the diagram

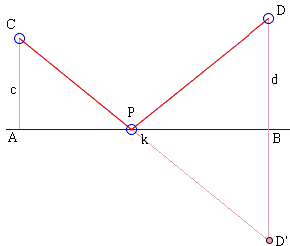
When P satisfies the minimality requirement, triangles APC and BPD' are similar from which

AP / AC = BP /BD = (AP + BP) / (AC + BD),

or,

p / c = k / (c + d),

so that

p = kc / (c + d).

References

1. H. Fukagawa, D. Pedoe, Japanese Temple Geometry Problems, The Charles Babbage Research Center, Winnipeg, 1989

   Write to:

   Charles Babbage Research Center
   P.O. Box 272, St. Norbert Postal Station
   Winnipeg, MB
   Canada R3V 1L6

2. P. J. Nahin, When Least Is Best, Princeton University Press, 2007 (Fifth printing).
3. V. M. Tikhomirov, Stories about Maximua and Minima, AMS & MAA, 1990
4. D. Wells, The Penguin Book of Curious and Interesting Geometry, Penguin, 1991
5. I. M. Yaglom, Geometric Transformations I, MAA, 1962

Sangaku

1. Sangaku: Reflections on the Phenomenon
2. Critique of My View and a Response
3. 1 + 27 = 12 + 16 Sangaku
4. 3-4-5 Triangle by a Kid
5. 7 = 2 + 5 Sangaku
6. A 49^th Degree Challenge
7. A Geometric Mean Sangaku
8. A Hard but Important Sangaku
9. A Restored Sangaku Problem
   • A better solution to a difficult sangaku problem
   • A Simple Solution to a Difficult Sangaku Problem
   • A Trigonometric Solution to a Difficult Sangaku Problem
10. A Sangaku: Two Unrelated Circles
11. A Sangaku by a Teen
12. A Sangaku Follow-Up on an Archimedes' Lemma
13. A Sangaku with an Egyptian Attachment
14. A Sangaku with Many Circles and Some
15. A Sushi Morsel
16. An Old Japanese Theorem
17. Archimedes Twins in the Edo Period
18. Arithmetic Mean Sangaku
19. Bottema Shatters Japan's Seclusion
20. Chain of Circles on a Chord
21. Circles and Semicircles in Rectangle
22. Circles in a Circular Segment
23. Circles Lined on the Legs of a Right Triangle
24. Equal Incircles Theorem
    • Equal Incircle Theorem, Angela Drei's Proof
25. Equilateral Triangle, Straight Line and Tangent Circles
26. Equilateral Triangles and Incircles in a Square
27. Five Incircles in a Square
28. Four Hinged Squares
29. Four Incircles in Equilateral Triangle
    • Four Incircles in an Equilateral Triangle, a Sangaku
30. Gion Shrine Problem
31. Harmonic Mean Sangaku
32. Heron's Problem
33. In the Wasan Spirit
34. Incenters in Cyclic Quadrilateral
35. Japanese Art and Mathematics
36. Malfatti's Problem
37. Maximal Properties of the Pythagorean Relation
38. Neuberg Sangaku
39. Out of Pentagon Sangaku
40. Peacock Tail Sangaku
41. Pentagon Proportions Sangaku
42. Proportions in Square
43. Pythagoras and Vecten Break Japan's Isolation
44. Radius of a Circle by Paper Folding
45. Review of Sacred Mathematics
46. Sangaku à la V. Thebault
47. Sangaku and The Egyptian Triangle
48. Sangaku in a Square
49. Sangaku Iterations, Is it Wasan?
50. Sangaku with 8 Circles
51. Sangaku with Angle between a Tangent and a Chord
52. Sangaku with Quadratic Optimization
53. Sangaku with Three Mixtilinear Circles
54. Sangaku with Versines
55. Sangakus with a Mixtilinear Circle
56. Sequences of Touching Circles
57. Square and Circle in a Gothic Cupola
58. Steiner's Sangaku
59. Tangent Circles and an Isosceles Triangle
60. The Squinting Eyes Theorem
61. Three Incircles In a Right Triangle
62. Three Squares and Two Ellipses
63. Three Tangent Circles Sangaku
    • An Extension of a Sangaku with Touching Circles
64. Triangles, Squares and Areas from Temple Geometry
65. Two Arbelos, Two Chains
66. Two Circles in an Angle
67. Two Sangaku with Equal Incircles
68. Another Sangaku in Square
69. Sangaku via Peru
70. FJG Capitan's Sangaku

Related material Read more...
	Heron's Formula: a Proof
	A Proof of the Pythagorean Theorem From Heron's Formula
	A Proof of the Pythagorean Theorem From Heron's Formula II
	A few corollaries from the Pythagorean Theorem

|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener|

Copyright © 1996-2018 Alexander Bogomolny