Three Tangent Circles Sangaku
This is one of the rather more popular on the web Sangaku problems:
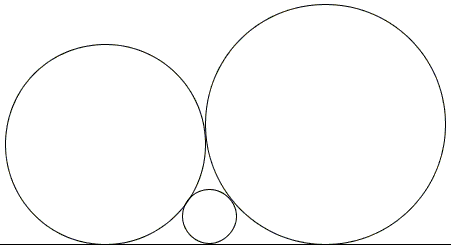
(See [Fukagawa & Pedoe, 1.1.2] where it is mentioned that the tablet survives in the Miyagai prefecture and is dated from 1892. There is a likely a misprint there as the authors refer for a solution to a publication from 1810.)
As some other Sangaku, this problem, too, requires nothing more than a few applications of the Pythagorean theorem. The main reason for its inclusion at the site is personal. I have only recently learned how to create in HTML the bar part of the symbol of square root. Because of the novelty, I am still enjoying doing that. As will be seen shortly, the Sangaku at hand provides an abundant opportunity to exercise the newly acquired skill.
References
H. Fukagawa, D. Pedoe, Japanese Temple Geometry Problems, The Charles Babbage Research Center, Winnipeg, 1989
Write to:
Charles Babbage Research Center
P.O. Box 272, St. Norbert Postal Station
Winnipeg, MB
Canada R3V 1L6
Sangaku
- Sangaku: Reflections on the Phenomenon
- Critique of My View and a Response
- 1 + 27 = 12 + 16 Sangaku
- 3-4-5 Triangle by a Kid
- 7 = 2 + 5 Sangaku
- A 49th Degree Challenge
- A Geometric Mean Sangaku
- A Hard but Important Sangaku
- A Restored Sangaku Problem
- A Sangaku: Two Unrelated Circles
- A Sangaku by a Teen
- A Sangaku Follow-Up on an Archimedes' Lemma
- A Sangaku with an Egyptian Attachment
- A Sangaku with Many Circles and Some
- A Sushi Morsel
- An Old Japanese Theorem
- Archimedes Twins in the Edo Period
- Arithmetic Mean Sangaku
- Bottema Shatters Japan's Seclusion
- Chain of Circles on a Chord
- Circles and Semicircles in Rectangle
- Circles in a Circular Segment
- Circles Lined on the Legs of a Right Triangle
- Equal Incircles Theorem
- Equilateral Triangle, Straight Line and Tangent Circles
- Equilateral Triangles and Incircles in a Square
- Five Incircles in a Square
- Four Hinged Squares
- Four Incircles in Equilateral Triangle
- Gion Shrine Problem
- Harmonic Mean Sangaku
- Heron's Problem
- In the Wasan Spirit
- Incenters in Cyclic Quadrilateral
- Japanese Art and Mathematics
- Malfatti's Problem
- Maximal Properties of the Pythagorean Relation
- Neuberg Sangaku
- Out of Pentagon Sangaku
- Peacock Tail Sangaku
- Pentagon Proportions Sangaku
- Proportions in Square
- Pythagoras and Vecten Break Japan's Isolation
- Radius of a Circle by Paper Folding
- Review of Sacred Mathematics
- Sangaku à la V. Thebault
- Sangaku and The Egyptian Triangle
- Sangaku in a Square
- Sangaku Iterations, Is it Wasan?
- Sangaku with 8 Circles
- Sangaku with Angle between a Tangent and a Chord
- Sangaku with Quadratic Optimization
- Sangaku with Three Mixtilinear Circles
- Sangaku with Versines
- Sangakus with a Mixtilinear Circle
- Sequences of Touching Circles
- Square and Circle in a Gothic Cupola
- Steiner's Sangaku
- Tangent Circles and an Isosceles Triangle
- The Squinting Eyes Theorem
- Three Incircles In a Right Triangle
- Three Squares and Two Ellipses
- Three Tangent Circles Sangaku
- Triangles, Squares and Areas from Temple Geometry
- Two Arbelos, Two Chains
- Two Circles in an Angle
- Two Sangaku with Equal Incircles
- Another Sangaku in Square
- Sangaku via Peru
- FJG Capitan's Sangaku
|Contact| |Front page| |Contents| |Geometry| |Up|
Copyright © 1996-2018 Alexander Bogomolny
As the diagram below shows we have three right triangles with the hypotenuses joining the centers of the three circles.

Using x and y to denote the horizontal distances between pairs of the circles, and R, R1, R2 at their radii, the triangles have the following sides:
R2 - R, y, R2 + R, and
R2 - R1, x + y, R2 + R1.
The Pythagorean theorem then yields three equations in three unknowns, x, y, and R:
(R2 - R)2 + y2 = (R2 + R)2, and
(R2 - R1)2 + (x + y)2 = (R2 + R1)2.
After simplification the equations become
y2 = 4R2R,
(x + y)2 = 4R1R2.
Taking the square roots and substituting the first two into the third we get
Divide now by √R√R1√R2 to obtain
Which is the desired formula.
|Contact| |Front page| |Contents| |Geometry| |Up|
Copyright © 1996-2018 Alexander Bogomolny
73284976
