The Law of Cosines (Cosine Rule)

The Law of Cosines (interchangeably known as the Cosine Rule or Cosine Law) is a generalization of the Pythagorean Theorem in that a formulation of the latter can be obtained from a formulation of the Law of Cosines as a particular case. However, all proofs of the former seem to implicitly depend on or explicitly consider the Pythagorean Theorem. For example, to be comprehensive, i.e. to cover the case µ = 0, the proof below should consider this case separately as it does not follow from the other two (µ<90 and µ>90). Thus, in the course of the proof of the Cosine Rule one proves directly the Pythagorean Theorem. For this reason I have difficulty asserting that the Cosine Rule implies the Pythagorean Theorem. I'll be extremely curious to learn of any proof of the Cosine Rule completely independent of the Pythagorean Theorem.

Note

Julian Gilbey took an issue with the last sentence: On the Law of Cosines page, you say "I'll be extremely curious to learn of any proof of the Cosine Rule completely independent of the Pythagorean Theorem." The answer is that there cannot be such a thing: the Law of Cosines is only true in Euclidean Geometry, not in spherical or hyperbolic geometry, so must depend upon the metric of the plane. And the metric of the Euclidean plane is precisely ds² = dx² + dy², which is equivalent to asserting that Pythagoras holds. With any other metric, Pythagoras does not hold, and therefore the Law of Cosines cannot hold, either.

However, John Molokach came up with a proof that does not appear to use the Pythagorean theorem. How can one explain the paradox?

The Law of Cosines

For a triangle with sides a,b, and c and the angle µ opposite the side c, one has

c² = a² + b² - 2ab·cos(µ)

Following is a proof of the Cosine Rule sent to me by Dr. Scott Brodie from the Mount Sinai School of Medicine, NY. In the beginning, when mentioning right triangles, Dr. Broddie refers to his proof of the Pythagorean Theorem.

Proof

If the original triangle is not right, one may still inquire as to the relationship between the lengths of the sides. To be specific, take as given a and b, the lengths of sides BC and AC, respectively, and consider the length, c, of side AB, as a function of µ, the magnitude of the angle at C. We will need the two additional forms of the "power of the point" theorem: If two such secant lines cut the same circle, the product of the distances along each to the near and far intersection points is the same for each secant; If two chords of a circle intersect in the interior of the circle, then the product of the distances from the intersection point to the circle in each direction along one chord is the same as the analogous product of the distances to the circle along the other chord.

There are three cases:

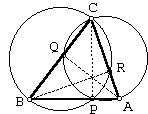
If the triangle is acute, construct the three altitudes, and, as before, the circles with diameters BC and AC. As before, the foot of the altitude from C to AB and the two circles coincide at a point, say P, which cuts AB into segments BP and PA of lengths x and y, respectively. If Q denotes the foot of the altitude from A, angle AQC is right, and Q lies on the circle with diameter AC. Inspection confirms that QC is of length bcos(µ). Similarly, let R denote the foot of the altitude from B; then R lies on circle BC, and RC is of length acos(µ). Then the Power Theorem for the case of two secants states, for the power of the point B with respect to circle AC, that a(a - bcos(µ)) = xc; for the power of the point A with respect to circle BC, we have b(b - acos(µ)) = yc. Adding the two equations yields immediately a² + b² - 2abcos(µ) = (x + y)c = c², which is the Law of Cosines.

If the original triangle is obtuse, we have two further cases:

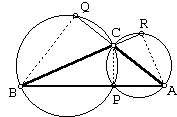
If angle C is obtuse, then the altitude from C cuts side AB say, at P, but the altitudes from A and B lie outside the triangle. The feet of these altitudes are found at the intersection of sides BC and AC, produced, with the circles with diameters AC and BC, respectively; call them Q and R. As before, let x and y denote the lengths of BP and PA. Since angle C is obtuse, cos(µ) is less than zero, and the lengths of RC and QC are and -acos(µ) and -bcos(µ), respectively. Then we have for the power of B with respect to circle AC a(a - bcos(µ)) = xc, and for the power of A with respect to circle BC b(b - acos(µ)) = yc. As before, adding the two equations yields the Law of Cosines.

Finally, if angle C is acute, but, say, angle B is obtuse, then the altitude from B cuts side AC, say at R, but the altitudes from A and C lie outside the triangle, and meet the sides CB and AB, produced, respectively, at, say Q and P. As before, Q and P lie on the circles with diameters AC and BC, respectively. Denote the distance BP by z. Since the length of RC is acos(µ), while the length of QC is bcos(µ), we have for the power of A with respect to circle BC b(b - acos(µ)) = (c + z)c, while, using the Power Theorem for an interior point, we have for the power of B with respect to circle AC a(bcos(µ) - a) = zc. In this case, subtracting the second equation from the first yields the Law of Cosines. QED.

Dr. Broddie also supplied a nice "dynamic" figure as a Geometer's Sketchpad file where moving vertex "C" nicely switches automatically between the various cases of the proof.

Other proofs can be found elsewhere. One proof without words is a direct generalization of Thâbit ibn Qurra's proof of the Pythagorean proposition. There is, as well, an "unfolded variant." Also, the Cosines Law admits a slightly different form discovered by Larry Hoehn that generalizes the Pythagorean theorem in a somewhat different way.

Trigonometry

72531787