On the Intersection of kx and |sin(x)|
Problem
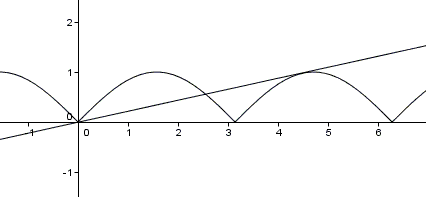
It's known that the curve $y=|\sin (x)|$ intercepts the line $y=kx$ ($k\gt 0)$ at exactly three points, the maximum $x$ coordinate of those points being $\alpha.$ Prove that
$\displaystyle\frac{\cos\alpha}{\sin\alpha +\sin 3\alpha}=\frac{1+\alpha^2}{4\alpha}.$
Hint
What can be said about the third intersection of $y=kx$ with $y=|\sin (x)|?$
Solution
The straight line $y=kx$ intersects the first arc of $y=|\sin (x)|$ in two points, leaving a single intersection for the second arc. A straight line and a convex arc (that, in this case, could be thought as closed) have a single intersection only when the two are tangent to each other.

The abscissa of the tangency point belongs to the interval $(\pi, 3\pi/2),$ where $\sin(x)\lt 0.$ In this interval, the given function equals $y=-\sin(x),$ with the derivative being $y'=-\cos(x).$ Thus, the slope $k$ of the line is, on one hand, $-\cos\alpha$ and, on the other hand, $\displaystyle -\frac{\sin\alpha}{\alpha},$ which leads to $\alpha =\tan\alpha.$
Now, use some basic trigonometry:
$\begin{align}\displaystyle \frac{\cos\alpha}{\sin\alpha +\sin 3\alpha}&=\frac{\cos\alpha}{2\sin 2\alpha \cos \alpha} =\frac{1}{4\sin\alpha \cos\alpha} \\ &=\frac{\sin^{2}\alpha+\cos^{2}\alpha}{4\sin\alpha \cos\alpha} =\frac{1+\tan^{2}\alpha}{4\tan\alpha} \\ &=\frac{1+\alpha^2}{4\alpha} \end{align}$
Generalize!
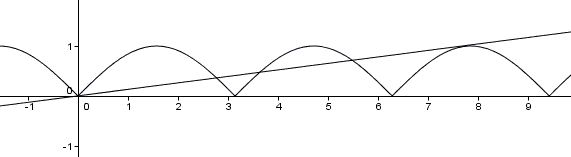
What if the number of intersections is $5$?
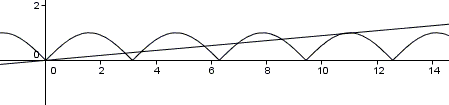
And what if it is $7$?
Acknowledgment
This is a problem from the 2009 Chinese Mathematics Competition [Xiong Bin, p 13].
References
- Xiong Bin, Lee Peng Yee, Mathematical Olympiads in China (2009-2010). Problems and Solutions, World Scientific, 2013
Trigonometry
- What Is Trigonometry?
- Addition and Subtraction Formulas for Sine and Cosine
- The Law of Cosines (Cosine Rule)
- Cosine of 36 degrees
- Tangent of 22.5o - Proof Wthout Words
- Sine and Cosine of 15 Degrees Angle
- Sine, Cosine, and Ptolemy's Theorem
- arctan(1) + arctan(2) + arctan(3) = π
- Trigonometry by Watching
- arctan(1/2) + arctan(1/3) = arctan(1)
- Morley's Miracle
- Napoleon's Theorem
- A Trigonometric Solution to a Difficult Sangaku Problem
- Trigonometric Form of Complex Numbers
- Derivatives of Sine and Cosine
- ΔABC is right iff sin²A + sin²B + sin²C = 2
- Advanced Identities
- Hunting Right Angles
- Point on Bisector in Right Angle
- Trigonometric Identities with Arctangents
- The Concurrency of the Altitudes in a Triangle - Trigonometric Proof
- Butterfly Trigonometry
- Binet's Formula with Cosines
- Another Face and Proof of a Trigonometric Identity
- cos/sin inequality
- On the Intersection of kx and |sin(x)|
- Cevians And Semicircles
- Double and Half Angle Formulas
- A Nice Trig Formula
- Another Golden Ratio in Semicircle
- Leo Giugiuc's Trigonometric Lemma
- Another Property of Points on Incircle
- Much from Little
- The Law of Cosines and the Law of Sines Are Equivalent
- Wonderful Trigonometry In Equilateral Triangle
- A Trigonometric Observation in Right Triangle
- A Quick Proof of cos(pi/7)cos(2.pi/7)cos(3.pi/7)=1/8
![]()
|Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73296085
