The Law of Cosines and the Law of Sines Are Equivalent
Sidney H. Kung
Cupertino, CA
December 2016
We show by using the projection property of triangle that the Law of Sines and the Law of Cosines are equivalent. Refer to the figure below:
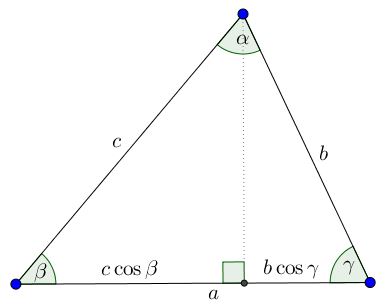
$\alpha+\beta+\gamma=\pi,\,$ $a=b\cos\gamma+c\cos\beta.\,$ (Note that the latter holds even when one of the angles $\beta,\gamma\,$ is obtuse.) By squaring,
$\begin{align} a^2 &= b^2\cos^2\gamma+c^2\cos^2\beta+2bc\cos\beta\cos\gamma\\ &=b^2(1-\sin^2\gamma)+c^2(1-\sin^2\beta)+2bc\cos\beta\cos\gamma\\ &=b^2+c^2+2bc[\cos\beta\cos\gamma-\sin\beta\sin\gamma]\\ &\qquad\qquad-[b^2\sin^2\gamma+c^2\sin^2\beta-2bc\sin\beta\sin\gamma]\\ &=[b^2+c^2+2bc\cos (\beta+\gamma)]-(b\sin\gamma-c\sin\beta)^2\\ &=[b^2+c^2-2bc\cos \alpha]-(b\sin\gamma-c\sin\beta)^2, \end{align}$
where we used the Addition Formula for Cosine. Thus,
(1)
$a^2=[b^2+c^2-2bc\cos (\alpha)]-(b\sin\gamma-c\sin\beta)^2.$
This expression indicates that the Law of Sines and the Law of Cosines are equivalent in that, the two conditions $a^2=b^2+c^2-2bc\cos (\alpha)\,$ and $b\sin\gamma-c\sin\beta=0\,$ either hold or do not hold simultaneously, the latter being equivalent to the Law of Sines $\displaystyle \frac{\sin\beta}{b}=\frac{\sin\gamma}{c},\,$ the former is the expression of the Law of Cosines.
There have been articles giving, separately, a proof for the Laws of Sines and Cosines or a proof of the Law of Sines using the Law of Cosines ([1],[2],[3]). But with (1) we can do both simultaneously.
References
- Law of cosines, Sec 3.7 Using the law of sines, wikipedia.org
- Patrik Nystedt, A proof of the law of sines using the law of cosines, to appear, 2017 Mathematics Magazine
- W. W. Sawyer, Prelude to Mathematics, Dover, 2011, pp 37-39
Trigonometry
- What Is Trigonometry?
- Addition and Subtraction Formulas for Sine and Cosine
- The Law of Cosines (Cosine Rule)
- Cosine of 36 degrees
- Tangent of 22.5o - Proof Wthout Words
- Sine and Cosine of 15 Degrees Angle
- Sine, Cosine, and Ptolemy's Theorem
- arctan(1) + arctan(2) + arctan(3) = π
- Trigonometry by Watching
- arctan(1/2) + arctan(1/3) = arctan(1)
- Morley's Miracle
- Napoleon's Theorem
- A Trigonometric Solution to a Difficult Sangaku Problem
- Trigonometric Form of Complex Numbers
- Derivatives of Sine and Cosine
- ΔABC is right iff sin²A + sin²B + sin²C = 2
- Advanced Identities
- Hunting Right Angles
- Point on Bisector in Right Angle
- Trigonometric Identities with Arctangents
- The Concurrency of the Altitudes in a Triangle - Trigonometric Proof
- Butterfly Trigonometry
- Binet's Formula with Cosines
- Another Face and Proof of a Trigonometric Identity
- cos/sin inequality
- On the Intersection of kx and |sin(x)|
- Cevians And Semicircles
- Double and Half Angle Formulas
- A Nice Trig Formula
- Another Golden Ratio in Semicircle
- Leo Giugiuc's Trigonometric Lemma
- Another Property of Points on Incircle
- Much from Little
- The Law of Cosines and the Law of Sines Are Equivalent
- Wonderful Trigonometry In Equilateral Triangle
- A Trigonometric Observation in Right Triangle
- A Quick Proof of cos(pi/7)cos(2.pi/7)cos(3.pi/7)=1/8
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny
73337158
