The Law of Cosines by Larry Hoehn

The essence of Larry Hoehn' paper (Forum Geometricorum, Volume 13 (2013) 133-134) is to prove the the Law of Cosines with no recourse to the Pythagorean theorem. We already have one such proof by John Molokach. Hoehn's proof fits nicely in the collection,

Theorem

For a triangle with sides $a$,$b$, and $c$ and the angle $\gamma$ opposite the side $c$, one has

$c^{2} = a^{2} + b^{2} - 2ab\cdot\cos\gamma.$

We let $ABC$ be any triangle whose incircle is tangent at $D,$ $E,$ and $F$ as shown in the diagram below, to the sides $AB,$ $BC,$ $AC.$ Let $a = BC,$ $b = CA,$ $c = AB,$ and $r$ be the radius of the incircle with incenter $I;$ $\gamma=\angle ACB.$ We derive our formula by equating independent expressions for $r.$

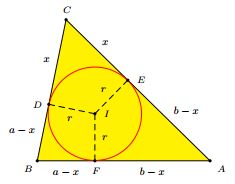
Larry Hoehn's proof of the Cosine Law

If $x = CD = CE,$ then $c = (a - x) + (b - x)$ so that $\displaystyle x = \frac{a + b - c}{2}.$ In the right triangle $CEI,$ $\displaystyle x = r \cot\frac{\gamma }{2}.$ Solving $\displaystyle \frac{1}{2}(a+b-c)=r\cot\frac{\gamma }{2}$ for $r$ yields

(1)

$\displaystyle r=\frac{a+b-c}{2\cot\frac{\gamma }{2}}.$

Computing the area of $\Delta ABC$ in two ways gives

$\displaystyle\frac{1}{2}ab\sin \gamma =\frac{1}{2}ar+\frac{1}{2}br+\frac{1}{2}cr,$

and

(2)

$\displaystyle r=\frac{ab\sin \gamma }{a+b+c}.$

From (1) and (2), we have

$\displaystyle\frac{a+b-c}{2\cot\frac{\gamma }{2}}=\frac{ab\sin \gamma }{a+b+c}.$

Cross multiplying and making use of some trigonometric identities leads to

$\begin{align}\displaystyle (a+b)^{2}-c^{2} &= 2ab\sin \gamma \cot\frac{\gamma }{2}\\ &=2ab\bigg(2\sin\frac{\gamma }{2}\cos\frac{\gamma }{2}\bigg)\frac{\cos\frac{\gamma }{2}}{sin\frac{\gamma }{2}}\\ &=4ab\cos ^{2}\frac{\gamma }{2}. \end{align}$

It follows that

$\begin{align}\displaystyle c^{2} &= (a+b)^{2}-4ab\space\cos ^{2}\frac{\gamma }{2}\\ &=a^{2}+b^{2}-2ab\bigg(\cos^{2}\frac{\gamma }{2}-1\bigg)\\ &=a^{2}+b^{2}-2ab\space\cos\gamma. \end{align}$

Since the identity $\sin^{2}x+\cos^{2}x=1$ (and hence $\cos 2x=2\cos^{2}x-1)$ can be derived independently of the Pythagorean theorem, the above shows that so can the Law of Cosines.

Remark

Since I first read this proof I had doubts about the validity of Larry Hoehn's designation as independent of the Pythagorean theorem. To justify that claim Hoehn refers to an article by Jason Zimba On the Possibility of Trigonometric Proofs of the Pythagorean Theorem, Forum Geometricorum, Volume 9 (2009) 275-278, where the identity $\sin^{2}x+\cos^{2}x=1$ is explcitly referred to as the Pythagorean theorem. In fact, Zimba derives this identity from the addition formulas for sine and cosine and thus declares that this is how the Pythagorean theorem can be demonstrated by trigonometric means, something that until then was thought impossible.

I am grateful to John Molokach whose comment below helped me set the record straight.

71945715