A Problem in Three Squares
What Is This About?
A Mathematical Droodle
Explanation
An interesting fact holds in a configuration of three equal squares placed side by side. If the lines drawn as shown then $\angle BDC + \angle BFC = \angle BEC.\,$ There are many proofs of this result. These are so simple, the problem has been included in many a puzzle book.
First note that $\angle BEC = 45^{\circ}.\,$ The task is then to show that $\angle BDC + \angle BFC = 45^{\circ}.\,$ Let's introduce $\alpha = \angle BDC\,$ and $\beta = \angle BFC.\,$ We are to prove that $\alpha + \beta = 45^{\circ}.\,$
Proof 1
Add another row of squares below the given three. $\Delta BKD\,$ is isosceles $(BK = DK)\,$ and, in addition, $\angle K = 90^{\circ}.\,$ Therefore, $\angle BDK = 45^{\circ}.\,$ However, since triangles $DEK\,$ and $FCB\,$ are equal, we also have $\angle KDE = \beta .$
Proof 2
Now with some trigonometry $(\arctan = \tan^{-1}),$
$\displaystyle \begin{align} \alpha + \beta &= \arctan(\frac{1}{3}) + \arctan(\frac{1}{2})\\ &= \arctan((\frac{1}{3} + \frac{1}{2})/(1 - \frac{1}{3}\cdot \frac{1}{2}))\\ &= \arctan(1). \end{align}$
where I used the standard formula for the tangent of a sum
$\displaystyle \tan(\alpha + \beta ) = \frac{\tan\alpha + \tan\beta}{1 - \tan\alpha\cdot \tan\beta}.$
Since $\arctan(1) = 45^{\circ},\,$ $\alpha + \beta = 45^{\circ}.$
Proof 3
Triangles $BDE\,$ and $FEB\,$ are similar.
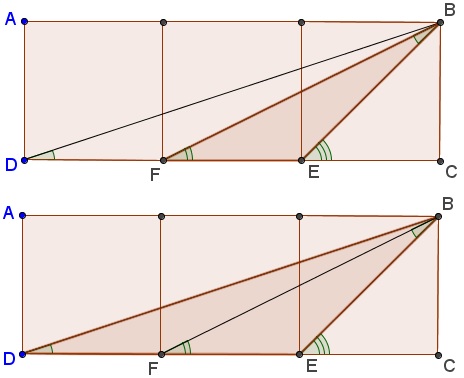
Indeed, assuming the side of the square equals $1,\,$ then
$\displaystyle \begin{array}{lll} \,DE=2, & BE=\sqrt{2}, &\displaystyle \frac{DE}{BE}=\sqrt{2},\\ BE=\sqrt{2}, & EF=1, &\displaystyle \frac{BE}{EF}=\sqrt{2},\\ BD=\sqrt{10}, & BF=\sqrt{5}, &\displaystyle \frac{BD}{BF}=\sqrt{2}. \end{array}$
which implies that $\angle BDC = \angle FBE = \alpha.\,$ Finally, the exterior angle $BEC\,$ of $\Delta FEB,\,$ equals the sum of the opposite interior angles, i.e., $\alpha + \beta.\,$ In short, we proved that
$\displaystyle \arctan(\frac{1}{2}) + \arctan(\frac{1}{3}) = \arctan(1).$
Observe that this implies another identity:
$\displaystyle \arctan(1) + \arctan(\frac{1}{2}) + \arctan(\frac{1}{3}) = \frac{\pi}{2}.$
Proof 4
Following a proof without words by Hasan Unal (Math Magazine, v 82, n 1, February 2009 , p. 56) we shall prove a more general statement. Let x and y be positive numbers satisfying $x^{2} + y^{2} = 1.\,$ Then
$\displaystyle\arctan\frac{1-x}{y}+\arctan\frac{1-y}{x}=\frac{\pi}{4}.$
The identity $\displaystyle \arctan(\frac{1}{2}) + \arctan(\frac{1}{3}) = \arctan(1) is obtained from the above by letting $\displaystyle x = \frac{3}{5}\,$ and $\displaystyle y = \frac4}{5},\,$ making it yet another appearance of the Egyptian 3-4-5 triangle. The proof is based on the following diagram.
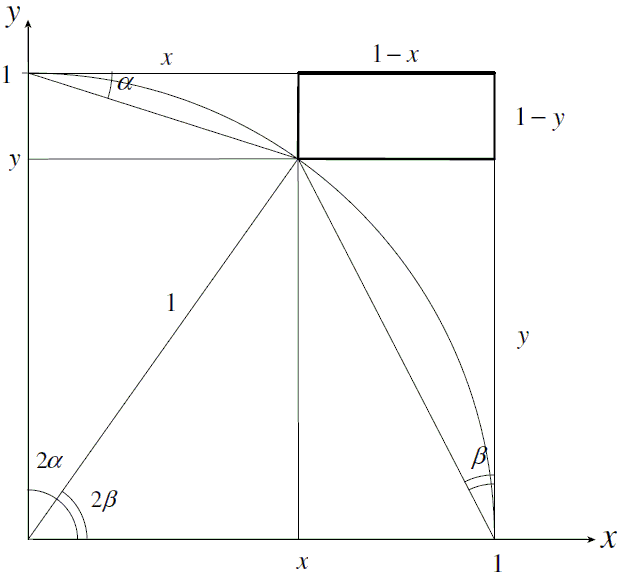
Set $\displaystyle \alpha = \frac{1 - y}{x},\,$ and $\displaystyle \beta = \frac{1 - x}{y}.\,$ We see at the origin that $\displaystyle 2\alpha + 2\beta = \frac{\pi}{2},\,$ implying $\displaystyle \alpha + \beta = \frac{\pi}{4} = \arctan(1).\,$
Elsewhere we prove another curiosity: $\arctan(1) + \arctan(2) + \arctan(3) = \pi.$
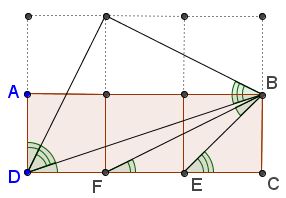
Proof 5
This and the next proof are from David Wells' newsletter, where more accurate references can be found.
This is a variant of proof #1 that qualifies as a proof without words.
Proof 6
The extra construction here is the circle circumscribing a five-square cross.
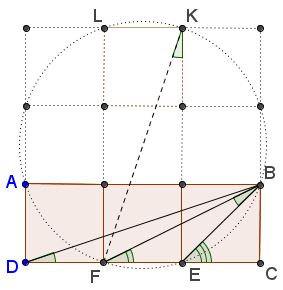
Here we have $\angle CDB=\angle EKF=\angle EBF,\;$ (as inscribed subtended by the same arc.) In addition, by the Exterior Angle Theorem, $\angle EBC=\angle EBF+\angle BFE.$
References
- B. Richardson, Three Squares Theorem, in The Changing Shape of Geometry, edited by C. Pritchard, Cambridge University Press, 2003
- A Decade of the Berkeley Mathematical Circle, The American Experience, Volume I, Z. Stankova, Tom Rike (eds), AMS/MSRI, 2008
- A Decade of the Berkeley Mathematical Circle, The American Experience, Volume II, Z. Stankova, Tom Rike (eds), AMS/MSRI, 2015, pp. 173-174
- D. Wells, Archimedes Mathematics Education Newsletter, #3, September 05 2016
The identity $\arctan(1)+\arctan(2)+\arctan(3)=\pi\,$ can be derived by paper folding and also becomes obvious in the 3-4-5 (Egyptian) triangle.
Trigonometry
- What Is Trigonometry?
- Addition and Subtraction Formulas for Sine and Cosine
- The Law of Cosines (Cosine Rule)
- Cosine of 36 degrees
- Tangent of 22.5o - Proof Wthout Words
- Sine and Cosine of 15 Degrees Angle
- Sine, Cosine, and Ptolemy's Theorem
- arctan(1) + arctan(2) + arctan(3) = π
- Trigonometry by Watching
- arctan(1/2) + arctan(1/3) = arctan(1)
- Morley's Miracle
- Napoleon's Theorem
- A Trigonometric Solution to a Difficult Sangaku Problem
- Trigonometric Form of Complex Numbers
- Derivatives of Sine and Cosine
- ΔABC is right iff sin²A + sin²B + sin²C = 2
- Advanced Identities
- Hunting Right Angles
- Point on Bisector in Right Angle
- Trigonometric Identities with Arctangents
- The Concurrency of the Altitudes in a Triangle - Trigonometric Proof
- Butterfly Trigonometry
- Binet's Formula with Cosines
- Another Face and Proof of a Trigonometric Identity
- cos/sin inequality
- On the Intersection of kx and |sin(x)|
- Cevians And Semicircles
- Double and Half Angle Formulas
- A Nice Trig Formula
- Another Golden Ratio in Semicircle
- Leo Giugiuc's Trigonometric Lemma
- Another Property of Points on Incircle
- Much from Little
- The Law of Cosines and the Law of Sines Are Equivalent
- Wonderful Trigonometry In Equilateral Triangle
- A Trigonometric Observation in Right Triangle
- A Quick Proof of cos(pi/7)cos(2.pi/7)cos(3.pi/7)=1/8
|Activities| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny
73296281
