Equal Incircles Theorem: What Is It About?
A Mathematical Droodle

---

This applet requires Sun's Java VM 2 which your browser may perceive as a popup. Which it is not. If you want to see the applet work, visit Sun's website at http://www.java.com/en/download/index.jsp, download and install Java VM and enjoy the applet.

---

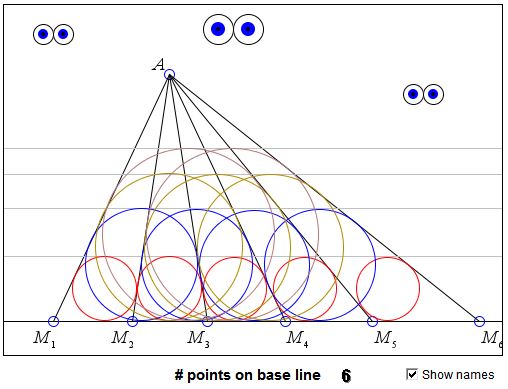
(The number of points on the base line which is originally 4 can be changed by clicking on it a little off its center line.)

Explanation

|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener| |Store|

Copyright © 1996-2012 Alexander Bogomolny

The applet suggests the following theorem [Wells, p. 67] from triangle geometry:

Let A be a point. Assume points M_i, i = 1, 2, ..., N (N > 3) lie on a line not through A. Assume further that the incircles of triangles M₁AM₂, M₂AM₃, ..., M_N-1AM_N all have equal radii. Then the same is true of triangles M₁AM₃, M₂AM₄, ..., M_N-2AM_N, and also of triangles M₁AM₄, M₂AM₅, ..., M_N-3AM_N, and so on.

---

This applet requires Sun's Java VM 2 which your browser may perceive as a popup. Which it is not. If you want to see the applet work, visit Sun's website at http://www.java.com/en/download/index.jsp, download and install Java VM and enjoy the applet.

---

I do not know of an elegant proof of that theorem. In [Wells, p. 67] it appears without a proof. There's no reference either.

The problem has been also discussed by R. Honsberger [Delights, section 17], where he mistakenly claims that the equality of the incircles only extends on the "triangles formed by sets of 2ⁿ consecutive triangles in the fan". The solution, nonetheless, works for a more general statement. Honsberger found this to be #2.2.5 in the Sangaku collection by H. Fukagawa and D. Pedoe. In fact problem #2.2.5 shows only two circles and the question is to find the length of the common tangent from the apex A in case the two circles are equal. In this form the problem appears on a surviving 1897 tablet from the Chiba prefecture. The answer to this problem can be found to be

AM₂ = √s(s - a),

where a is the base and s is the semiperimeter of the triangle.

However, the theorem is a consequence (and also a generalization) of a theorem published in 1986 by H. Demir, which, as was shown by J. B. Tabov, admits if not a more elegant proof, then quite an elegant generalization:

Assume n > 4, and the inradii of the "first level" triangles are equal in pairs: the inradius of ΔM_iAM_i+1 is equal to that of ΔM_i+2AM_i+3, i = 1, 2, ..., n-3. Then the incircles of the "second level" triangles M_iAM_i+2 are all equal.

(There is another, simple proof of the general statement.)

References

1. H. Fukagawa, D. Pedoe, Japanese Temple Geometry Problems, The Charles Babbage Research Center, Winnipeg, 1989
2. R. Honsberger, Mathematical Delights, MAA, 2004
3. D. Wells, Curious and Interesting Geometry, Penguin Books, 1991

Sangaku

1. Sangaku: Reflections on the Phenomenon
2. Critique of My View and a Response
3. 1 + 27 = 12 + 16 Sangaku
4. 3-4-5 Triangle by a Kid
5. 7 = 2 + 5 Sangaku
6. A 49^th Degree Challenge
7. A Geometric Mean Sangaku
8. A Hard but Important Sangaku
9. A Restored Sangaku Problem
   • A better solution to a difficult sangaku problem
   • A Trigonometric Solution to a Difficult Sangaku Problem
10. A Sangaku: Two Unrelated Circles
11. A Sangaku by a Teen
12. A Sangaku Follow-Up on an Archimedes' Lemma
13. A Sangaku with an Egyptian Attachment
14. A Sangaku with Many Circles and Some
15. A Sushi Morsel
16. An Old Japanese Theorem
17. Archimedes Twins in the Edo Period
18. Arithmetic Mean Sangaku
19. Bottema Shatters Japan's Seclusion
20. Chain of Circles on a Chord
21. Circles and Semicircles in Rectangle
22. Circles in a Circular Segment
23. Circles Lined on the Legs of a Right Triangle
24. Equal Incircles Theorem
    • Equal Incircle Theorem, Angela Drei's Proof
25. Equilateral Triangle, Straight Line and Tangent Circles
26. Equilateral Triangles and Incircles in a Square
27. Five Incircles in a Square
28. Four Hinged Squares
29. Four Incircles in Equilateral Triangle
30. Gion Shrine Problem
31. Harmonic Mean Sangaku
32. Heron's Problem
33. In the Wasan Spirit
34. Incenters in Cyclic Quadrilateral
35. Japanese Art and Mathematics
36. Malfatti's Problem
37. Maximal Properties of the Pythagorean Relation
38. Neuberg Sangaku
39. Out of Pentagon Sangaku
40. Peacock Tail Sangaku
41. Pentagon Proportions Sangaku
42. Proportions in Square
43. Pythagoras and Vecten Break Japan's Isolation
44. Radius of a Circle by Paper Folding
45. Review of Sacred Mathematics
46. Sangaku à la V. Thebault
47. Sangaku and The Egyptian Triangle
48. Sangaku in a Square
49. Sangaku Iterations, Is it Wasan?
50. Sangaku with 8 Circles
51. Sangaku with Angle between a Tangent and a Chord
52. Sangaku with Quadratic Optimization
53. Sangaku with Three Mixtilinear Circles
54. Sangaku with Versines
55. Sangakus with a Mixtilinear Circle
56. Sequences of Touching Circles
57. Square and Circle in a Gothic Cupola
58. Steiner's Sangaku
59. Tangent Circles and an Isosceles Triangle
60. The Squinting Eyes Theorem
61. Three Incircles In a Right Triangle
62. Three Squares and Two Ellipses
63. Three Tangent Circles Sangaku
64. Triangles, Squares and Areas from Temple Geometry
65. Two Arbelos, Two Chains
66. Two Circles in an Angle
67. Two Sangaku with Equal Incircles

|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener| |Store|

Copyright © 1996-2012 Alexander Bogomolny