Golden Ratio With Two Unequal Circles And a Line II

The following two constructions of the Golden Ratio are due to Nguyen Thanh Dung. The second one appears a fall back to the famous construction by George Odom, but the semblance is only superficial, the two constructions are distinct.

In the diagram, $AB=BC=CD,\;$ $AO=DO,\;$ $BM=MO;\;$ $TX\perp AD,\;$ $M\in TX.$

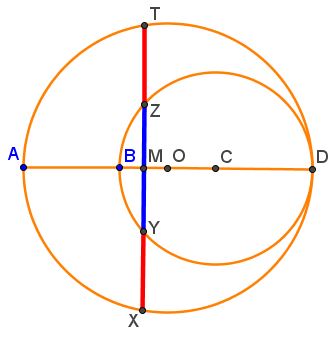
Golden Ratio With Two Unequal Circles And a Line II, Construction 1

Then the ratio of the blue segment to a red one is Golden: $\displaystyle\frac{YZ}{TZ}=\varphi.$

For a proof, assume $AB=4.\;$ Then in $\Delta CMZ,\;$ $CM=3,\;$ $CZ=4\;$ so that $MZ=\sqrt{7}\;$ and $YZ=2\sqrt{7}.$

In $\Delta OMT,\;$ $MO=1,\;$ $OT=6;\;$ so that $MT=\sqrt{35}.\;$ $TZ=\sqrt{35}-\sqrt{7}.\;$ It follows that

$\displaystyle\frac{YZ}{TZ}=\frac{2\sqrt{7}}{\sqrt{35}-\sqrt{7}}=\frac{2}{\sqrt{5}-1}=\varphi.$

For a related construction, let $AE\;$ and $AF\;$ be tangent to circle $(C),\;$ with $E,F\;$ on circle $(O).\;$ The $\Delta AEF\;$ is equilateral, with the circumcircle $(O)\;$ and a mixtilinear circle $(C).\;$ This could have been a point of departure.

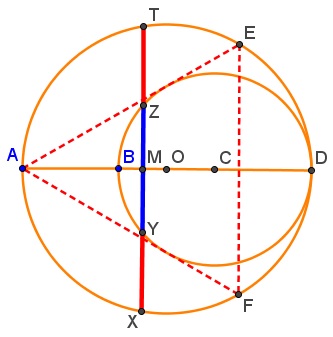
Golden Ratio With Two Unequal Circles And a Line II, Construction 2

To see that $\Delta AEF\;$ is equilateral, consider $\Delta ACG,\;$ as shown below:

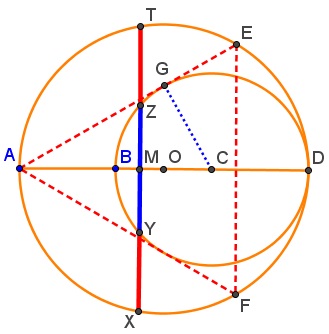
Golden Ratio With Two Unequal Circles And a Line II, Construction 2+

Since $AC=8\;$ and $\displaystyle CG=4=\frac{AC}{2},\;$ $\angle CAG=30^{\circ},\;$ so that $\angle EAF=60^{\circ}.$

Golden Ratio

72104212