Golden Ratio in Isosceles Triangle II
Source
This problem was posted by Ercole Suppa at the Peru Geometrico facebook group, and pointed to me by Leo Giugiuc. I post one solution below. More proofs can be found at the aforementioned link.

Problem
In an isosceles $\Delta ABC,$ $AB=AC\gt BC,$ circle $C(O,M,N)$ centered at the circumcenter $O$ and passing through the midpoints $M$ and $N$ of the side $AB$ and $AC,$ respectively also passes through $O_9$ the center of the nine-point circle. Let $r$ be the radius of $(MNO_9)$ and $r_9$ the radius of the nine-point circle $(LMN).$
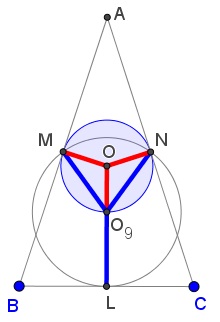
Prove that $\displaystyle\frac{AC}{BC}=\frac{r_9}{r}=\varphi,$ the Golden Ratio.
Solution
Let's denote $a=BC$ and $b=AC,$ and let $R$ be the circumradius of $\Delta ABC.$ Note that $R=2r_9.$
Triangles $AON$ and $ACL$ are similar, so that
$\displaystyle \frac{2r_9}{r}=\frac{R}{r}=\frac{AO}{ON}=\frac{AC}{CL}=\frac{b}{a/2}=\frac{2b}{a}.$
This proves $\displaystyle \frac{r_9}{r}=\frac{b}{a}.$ Let $t$ stand for that ratio.
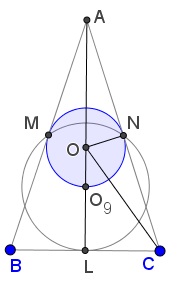
In $\Delta AON,$ $AO^2-ON^2=AN^2,$ i.e., $\displaystyle (2r_9)^2-r^2=\left(\frac{b}{2}\right)^2$.
In $\Delta OLC,$ $CO^2-OL^2=CL^2,$ i.e., $\displaystyle (2r_9)^2-(r+r_9)^2=\left(\frac{a}{2}\right)^2$.
Dividing the former by the latter, $\displaystyle \frac{4t^2-1}{3t^2-2t-1}=t^2,$ or
$3t^4-2t^3-5t^2+1=0.$
But $3t^4-2t^3-5t^2+1=(t^2-t-1)(3t^2+t-1).$ The only positive root of the equation $t^2-t-1=0$ is $t=\varphi,$ the Golden Ratio. The only positive root of the other factor $3t^2+t-1=0$ is $\displaystyle t=\frac{\sqrt{13}-1}{6}\lt\frac{4-1}{6}=\frac{1}{2},$ which could not be the ratio $\displaystyle \frac{b}{a}$ of the two sides of $\Delta ABC.$
Extra
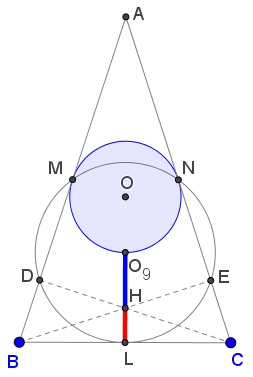
If $H$ is the orthocenter of $\Delta ABC,$ then $\displaystyle \frac{HO_9}{HL}=\varphi,$ the Golden ratio.
Golden Ratio
- Golden Ratio in Geometry
- Golden Ratio in Regular Pentagon
- Golden Ratio in an Irregular Pentagon
- Golden Ratio in a Irregular Pentagon II
- Inflection Points of Fourth Degree Polynomials
- Wythoff's Nim
- Inscribing a regular pentagon in a circle - and proving it
- Cosine of 36 degrees
- Continued Fractions
- Golden Window
- Golden Ratio and the Egyptian Triangle
- Golden Ratio by Compass Only
- Golden Ratio with a Rusty Compass
- From Equilateral Triangle and Square to Golden Ratio
- Golden Ratio and Midpoints
- Golden Section in Two Equilateral Triangles
- Golden Section in Two Equilateral Triangles, II
- Golden Ratio is Irrational
- Triangles with Sides in Geometric Progression
- Golden Ratio in Hexagon
- Golden Ratio in Equilateral Triangles
- Golden Ratio in Square
- Golden Ratio via van Obel's Theorem
- Golden Ratio in Circle - in Droves
- From 3 to Golden Ratio in Semicircle
- Another Golden Ratio in Semicircle
- Golden Ratio in Two Squares
- Golden Ratio in Two Equilateral Triangles
- Golden Ratio As a Mathematical Morsel
- Golden Ratio in Inscribed Equilateral Triangles
- Golden Ratio in a Rhombus
- Golden Ratio in Five Steps
- Between a Cross and a Square
- Four Golden Circles
- Golden Ratio in Mixtilinear Circles
- Golden Ratio With Two Equal Circles And a Line
- Golden Ratio in a Chain of Polygons, So to Speak
- Golden Ratio With Two Unequal Circles And a Line
- Golden Ratio In a 3x3 Square
- Golden Ratio In a 3x3 Square II
- Golden Ratio In Three Tangent Circles
- Golden Ratio In Right Isosceles Triangle
- Golden Ratio Poster
- Golden Ratio Next to the Poster
- Golden Ratio In Rectangles
- Golden Ratio In a 2x2 Square: Without And Within
- Golden Ratio With Two Unequal Circles And a Line II
- Golden Ratio in Equilateral and Right Isosceles Triangles
- Golden Ratio in a Butterfly Astride an Equilateral Triangle
- The Golden Pentacross
- 5-Step Construction of the Golden Ratio, One of Many
- Golden Ratio in 5-gon and 6-gon
- Golden Ratio in an Isosceles Trapezoid with a 60 degrees Angle
- Golden Ratio in Pentagon And Two Squares
- Golden Ratio in Pentagon And Three Triangles
- Golden Ratio in a Mutually Beneficial Relationship
- Star, Six Pentagons and Golden Ratio
- Rotating Square in Search of the Golden Ratio
- Cultivating Regular Pentagons
- Golden Ratio in an Isosceles Trapezoid with a 60 degrees Angle II
- More of Gloden Ratio in Equilateral Triangles
- Golden Ratio in Three Regular Pentagons
- Golden Ratio in Three Regular Pentagons II
- Golden Ratio in Wu Xing
- Golden Ratio In Three Circles And Common Secant
- Flat Probabilities on a Sphere
- Golden Ratio in Square And Circles
- Golden Ratio in Square
- Golden Ratio in Two Squares, Or, Perhaps in Three
- Golden Ratio in Isosceles Triangle
- Golden Ratio in Circles
- Golden Ratio in Isosceles Triangle II
- Golden Ratio in Yin-Yang
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73349495
