Radius and Construction of a Mixtilinear Circle: What is this about?
A Mathematical Droodle

Explanation

|Activities| |Contact| |Front page| |Contents| |Geometry| |Store|

The applet illustrates a simple construction of a mixtilinear circle based on a formula

where α = ∠BAC, r is the radius of the incircle of ΔABC and r_A is the radius of the mixtilinear incircle inscribed into angle BAC.

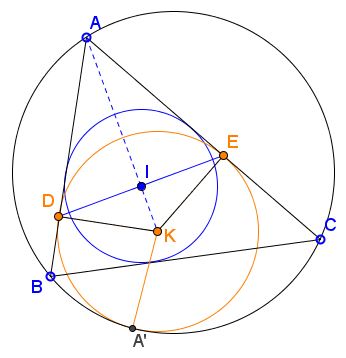
The construction is this: first draw a perpendicular to the bisector of ∠BAC through the incenter I. This line will meet the sides AB and AC in points D and E. Second, draw a perpendicular to AB at D and a perpendicular to AC at E. These these two lines will meet again on the bisector of ∠BAC in the center of the mixtilinear incircle touching AB and AC.

References

1. P. Yiu, Mixtilinear Circles, Am Math Monthly, v. 106, n. 10 (Dec., 1999), 952-955
2. P. Yiu, Notes on Euclidean Geometry, 1998, pp. 56-57

|Activities| |Contact| |Front page| |Contents| |Geometry| |Store|