Seven and the Eighth Circle Theorem

Problem

Three green circles touch each other externally, and a larger circle $\omega$ internally. Three additional (red) circles each touch externally two of the green neighbors and internally $\omega.$ Points $A_1,$ $A_2,$ $A_3,$ $A_4,$ $A_5,$ $A_6$ are the points of tangency as shown:

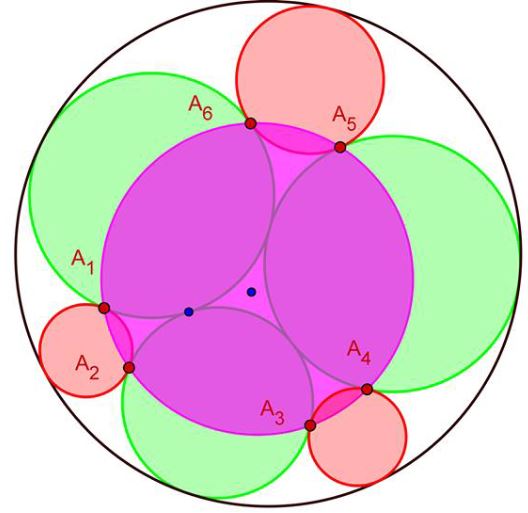
Seven and the Eighth Circle Theorem - problem

Prove that $A_1,$ $A_2,$ $A_3,$ $A_4,$ $A_5,$ $A_6$ are concyclic.

The proof below is based on the following

Lemma

Assume that inversion in circle $c$ maps circle $c_1$ onto circle $c_2.$ Then, for any point $X\in c,$ any inversion with center $X$ maps $c_1$ and $c_2$ into equal circles.

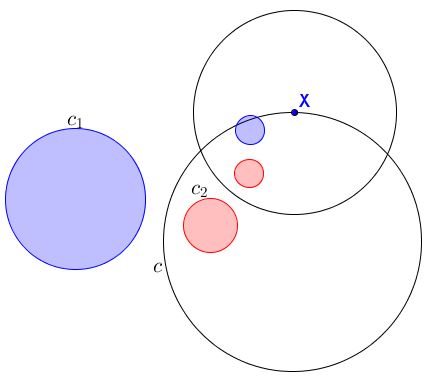
Seven and the Eighth Circle Theorem - lemma

The proof can be found on a separate page.

Proof of the statement

Denote the green circle $G_1,$ $G_2,$ $G_3,$ say $G_{1}=(A_{6}A_{1}),$ $G_{2}=(A_{2}A_{3}),$ $G_{3}=(A_{4}A_{5}).$ Circles $G_1,$ $G_2,$ are either equal or not. If they are not, find an inversion that maps one on top of the other (this would be an inversion in a circle centered at their center of similitude and passing through their point of tangency) and, following the lemma, another one that maps them into equal circles. The whole configuration will be inverted into an essentially same configuration but with two green circles $G_1,$ $G_2$ equal.

Any inversion centered on the common tangent of $G_1,$ $G_2$ (i.e., their radical axis) will keep their images equal. If $G_3$ is equal to the other two, we are done. If not, find an inversion that maps $G_1,$ $G_3$ onto each other. This is an inversion in the circle centered at their center of similitude and passing through their point of tangency. This circle is bound to cross the radical axis of $G_1,$ $G_2$ because this is where - due to the acquired symmetry - $G_3$ is now centered. Use the point of intersection to invert all three circles into equal ones.

In a configuration where all three green circles are equal, the six points $A_1,$ $A_2,$ $A_3,$ $A_4,$ $A_5,$ $A_6$ are concyclic due to the symmetry of the configuration. Performing the sequence of inversions backwards shows that the same is true for the original configuration.

Acknowledgment

The problem has been posted by Dao Thanh Oai (Vietnam) at the CutTheKnotMath facebook page. Lemma is problem 3.8.15 from H. Eves, A Survey of Geometry.

Inversion - Introduction

72347778