A Cyclic Inequality with Powers 2 through 7
Problem
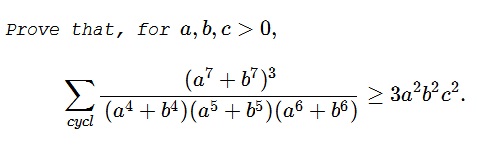
Solution 1
By the power-mean inequality, for $n\ge m,$
$\displaystyle \left(\frac{a^n+b^n}{2}\right)^{\frac{1}{n}}\ge\left(\frac{a^m+b^m}{2}\right)^{\frac{1}{m}}$
which can be rewritten as
$\displaystyle \frac{a^n+b^n}{a^m+b^m}\ge \left(\frac{a^m+b^m}{2}\right)^{\frac{n}{m}-1},$
implying, by the AM-GM inequality,
$\displaystyle \frac{a^n+b^n}{a^m+b^m} \ge \left(a^m+b^m\right)^{\frac{n}{m}-1}\ge (ab)^{\frac{n-m}{2}}.$
It follows that
$\displaystyle\begin{align} \frac{(a^7+b^7)^3}{(a^4+b^4)(a^5+b^5)(a^6+b^6)}&=\frac{a^7+b^7}{a^4+b^4}\cdot\frac{a^7+b^7}{a^5+b^5}\cdot\frac{a^7+b^7}{a^6+b^6}\\ &\ge (ab)^{\frac{7-4}{2}} (ab)^{\frac{7-5}{2}} (ab)^{\frac{7-6}{2}}=(ab)^3. \end{align}$
Again, with the AM-GM inequality,
$\displaystyle\begin{align}\sum_{cycl}\frac{(a^7+b^7)^3}{(a^4+b^4)(a^5+b^5)(a^6+b^6)}&\ge\sum_{cycl}(ab)^3\\ &\ge 3a^2b^2c^2. \end{align}$
Solution 2
For $k<7$,
$\displaystyle \begin{align} \frac{a^7+b^7}{a^k+b^k}&\geq 2^{\left(1-7/k\right)}(a^k+b^k)^{\left(7/k-1\right)}~\text{(Power-mean/numerator)}\\ &\geq 2^{\left(1-7/k\right)}2^{\left(7/k-1\right)}(ab)^{\left(\frac{7-k}{2}\right)}~\text{(AM-GM)}.\\ &=(ab)^{\left(\frac{7-k}{2}\right)} \end{align}$
Thus,
$\displaystyle \begin{align} \sum_{cyc} \frac{(a^7+b^7)^3}{(a^4+b^4)(a^5+b^5)(a^6+b^6)}&\geq \sum_{cyc} (ab)^{\left(\frac{3}{2}+1+\frac{1}{2}\right)}\\ &=\sum_{cyc} (ab)^3\geq 3a^2b^2c^2~\text{(AM-GM)}. \end{align}$
Acknowledgment
Dan Sitaru has kindly posted at the CutTheKnotMath facebook page the above problem of his with a solution (Solution 1) by Lazaros Reggina Zachariades. The problem was previously published in the Romanian Mathematical Magazine. Solution 2 is by Amit Itagi.
Cyclic inequalities in three variables
- ab + bc + ca does not exceed aa + bb + cc
- A Cyclic Inequality in Three Variables $\left(\displaystyle\frac{a^3}{b^2(5a+2b)}+\frac{b^3}{c^2(5b+2c)}+\frac{c^3}{a^2(5c+2a)}\ge\frac{3}{7}\right)$
- A Cyclic Inequality in Three Variables II $\left(\displaystyle\frac{10a^3}{3a^2+7bc}+\frac{10b^3}{3b^2+7ca}+\frac{10c^3}{3a^2+7ab}\ge a+b+c\right)$
- A Cyclic Inequality in Three Variables III $\left(\displaystyle\sqrt{\frac{a}{b+c}}+\sqrt{\frac{b}{c+a}}+\sqrt{\frac{c}{a+b}}\ge 2\right)$
- A Cyclic Inequality in Three Variables IV $\left(\displaystyle 2\sum_{cycl}(a+b)^3+5\sum_{cycl}a^3\ge 21\sum_{cycl}a^2b\right)$
- A Cyclic Inequality in Three Variables V $\left(\displaystyle \frac{a}{b}+\frac{b}{c}+\frac{c}{a}\ge\frac{\sqrt{3(a^2+b^2+c^2)}\cdot (a+b+c)}{ab+bc+ca}\right)$
- A Cyclic Inequality in Three Variables VI $\left(\displaystyle \frac{2(a+b+c)}{abc}\ge\sum_{cycl}\left(\sqrt{\frac{a+b}{2ac}}+\sqrt{\frac{2a}{c(a+b)}}\right)\right)$
- A Cyclic Inequality in Three Variables VII $\left(\displaystyle\sum_{cycl}x\sqrt{x^2z^2+y^4}\ge\sqrt{2}\sum_{cycl}xz\sqrt{yz}\right)$
- A Cyclic Inequality in Three Variables VIII $\left(\displaystyle\sum_{cycl}(x^2+y^2)z+\sum_{cycl}\frac{xy}{(x+y)^2}\ge 27xyz\right)$
- A Cyclic Inequality in Three Variables IX $\left(\displaystyle 9\left(\sum_{cycl}\frac{x^2}{y^2}\right)^2\ge 8\left(\sum_{cycl}\frac{x}{y}\right)\left(\sum_{cycl}\frac{x^3}{y^3}-3\right)\right)$
- A Cyclic Inequality in Three Variables X $\left(\displaystyle\sum_{cycl}\frac{1}{(a+1)^3}+4\sum_{cycl}\frac{1}{(a+1)^4}\ge\frac{9}{8}\right)$
- A Cyclic Inequality in Three Variables XI $\left(\displaystyle\sum_{cycl}\frac{1}{(a^2-ab+b^2)(b^2-bc+c^2)}\le\sum_{cycl}\frac{1}{a^4}\right)$
- A Cyclic Inequality in Three Variables XII $\left(\displaystyle\left(\sum_{cycl}\frac{1}{(a^2-ab+b^2)^6}\right)^2\le 3\sum_{cycl}\left(\frac{a+b}{a^2+b^2}\right)^{24}\right)$
- A Cyclic Inequality in Three Variables XIII $\left(\displaystyle\sum_{cycl}\frac{a^2+b^2}{a+b}+11\sum_{cycl}\frac{ab}{a+b}\gt 6\sum_{cycl}\sqrt{ab}\right)$
- A Cyclic Inequality in Three Variables XIV $\left(\displaystyle\sum_{cycl}\frac{xy}{xy+y^2+zx}\le 1\right)$
- A Cyclic Inequality in Three Variables XV $\left(\displaystyle \frac{a(a^2+b^2)}{a^3+b^3}+\frac{b(b^2+c^2)}{b^3+c^3}+\frac{c(c^2+a^2)}{c^3+a^3}\leq \sqrt{\frac{a}{b}}+\sqrt{\frac{b}{c}}+\sqrt{\frac{c}{a}}\right)$
- A Cyclic Inequality in Three Variables XVI $\left(\displaystyle \sum_{cycl}|(a+b)(1-ab)|\lt\frac{3}{2}+\sum_{cycl}a^2+\frac{1}{2}\sum_{cycl}a^4\right)$
- A Cyclic Inequality in Three Variables XVII $\left(\displaystyle \left(\sum_{cycl}\frac{x^2}{y^2}\right)^5 \ge 9\left(\sum_{cycl}\frac{x^3}{y^2z}\right)\left(\sum_{cycl}\frac{x}{\sqrt{yz}}\right)\left(\sum_{cycl}\frac{y}{z}\right)\right)$
- A Cyclic Inequality in Three Variables XVIII $\left(\displaystyle \left(\sum_{cycl}\sqrt{ab}\right)^6 \le 27\prod_{cycl}(a^2+ab+b^2)\right)$
- A Cyclic Inequality in Three Variables XIX $\left(\displaystyle \frac{x}{(y+z)^3}+\frac{y}{(z+x)^3}+\frac{z}{(x+y)^3}\ge\frac{27}{8(x+y+z)^2}\right)$
- A Cyclic Inequality in Three Variables XX $\left(\displaystyle 5\sum_{cycl}\sqrt{ab}\le\sum_{cycl}\sqrt[4]{(a+4b)(2a+3b)(3b+2a)(4a+b)}\le 5\right)$
- A Cyclic Inequality in Three Variables XXI $\left(\displaystyle \frac{abc}{7\sqrt{7}}\le\prod_{cycl}\frac{a^2-ab+b^2}{\sqrt{a^2+5ab+b^2}}\right)$
- A Cyclic Inequality in Three Variables XXII $\left(\displaystyle \sum_{cycl}\frac{a^3}{a^2+ab+b^2}\ge\frac{a+b+c}{3}\right)$
- A Cyclic Inequality in Three Variables XXIII $\left(\displaystyle 3(a^2+b^2+c^2)^2\ge 8abc(a+b+c)+\sum_{cycl}(a^2+b^2-c^2)^2\right)$
- A Cyclic Inequality in Three Variables XXIV $\left(\displaystyle \sum_{cycl} \frac{a^2b^2 (1+a^2)(1+b^2)}{(1+a)(1+b)}\geq 4(3-2\sqrt{2})abc(a+b+c)\right)$
- A Cyclic Inequality in Three Variables XXV $\left(\displaystyle \sum_{cycl} (a-\sqrt{ab}+b)^2\cdot\sum_{cycl}(a^2-ab+b^2)^2\ge 9a^2b^2c^2\right)$
- A Cyclic Inequality in Three Variables And One More $\left(\displaystyle \left(\sum_{cycl}x^{2m+2}\right)\cdot\left(\sum_{cycl}\frac{1}{(x+y)^{2m+2}}\right)\ge\frac{9}{4^{m+1}}\right)$
- Dorin Marghidanu's Cyclic Inequality in Three Variables $\left(\displaystyle\sum_{cycl}\sqrt{a^2-ab+b^2}\sqrt{b^2-bc+c^2}\ge a^2+b^2+c^2\right)$
- Dorin Marghidanu's Cyclic Inequality in Three Variables II
$\left(\displaystyle\sum_{cycl}\frac{ab}{(a+c)(b+c)} \ge\frac{3}{4}\right)$
Dorin Marghidanu's Cyclic Inequality in Three Variables III
$\left(\displaystyle \frac{a^3}{b^2+c}+\frac{b^3}{c^2+a}+\frac{c^3}{a^2+b}\gt \frac{3}{2}(abc)^{\frac{2}{3}}\right)$
- Leo Giugiuc's Cyclic Inequality in Three Variables $\left(\displaystyle a^2+b^2+c^2\ge 3\sqrt[3]{\frac{1}{4}(a-b)^2(b-c)^2(c-a)^2}+ab+bc+ca\right)$
- Tran Hoang Nam's Cyclic Inequality in Three Variables $\left(\displaystyle\sum_{cycl}(a-b)^3(a-c)^3 \le \left(\sum_{cycl}a^2-\sum_{cycl}ab\right)^3\right)$
- Cyclic Inequality with Square Roots $\left(\displaystyle 2\sqrt{2}\sum_{cycl}xy\ge\sqrt{2xyz}\sum_{cycl}\sqrt{x}+\sum_{cycl}\sqrt{x^2z^2+y^2z^2}\right)$
- Cyclic Inequality with Logarithms $\left(\displaystyle \ln \left(a^b\cdot b^c\cdot c^a\right)+6\sum_{cycl}\frac{b(1+2a)}{1+4a+a^2}\ge 3(a+b+c)\right)$
- A Cyclic Inequality with Many Sums $\left(\displaystyle\small{ \left(\sum_{cycl}a^4\right)\left(\sum_{cycl}\frac{a}{b}\right)\left(\sum_{cycl}a^3\right)\left(\sum_{cycl}\frac{a}{c}\right)\left(\sum_{cycl}a^2\right) \ge \left(\sum_{cycl}a\right)^3\left(\sum_{cycl}\frac{1}{a}\right)^2}\right)$
- Dan Sitaru's Cyclic Inequality in Three Variables III $\left(\displaystyle 4\sqrt{\sum_{cycl}\frac{a}{(a-1)^2}}\ge\sqrt{6}(10-a-b-c)\right)$
- Imad Zak's Cyclic Inequality in Three Variables $\left(\displaystyle \frac{(a+b+c)^2}{ab+bc+ca} + \frac{ab+bc+ca}{a^2+b^2+c^2}\ge 4\right)$
- Imad Zak's Cyclic Inequality in Three Variables II $\left(\displaystyle \frac{ab+bc+ca}{(a+b+c)^2} + \frac{a^2+b^2+c^2}{ab+bc+ca}\ge \frac{4}{3}\right)$
- A Simple Cyclic Inequality in Three Variables $\left(\displaystyle 3\left(\sum_{cycl}a^2\right)^2\ge 8abc(a+b+c)+\sum_{cycl}(a^2+b^2-c^2)^2\right)$
- An Inequality with Cyclic Sums on Both Sides $\left(\displaystyle \sum_{cycl}\frac{a^9}{b^6c^2}\ge\sum_{cycl}\sqrt[6]{\frac{a^{28}}{b^{17}c^5}}\right)$
- An Inequality with Cyclic Sums on Both Sides II $\left(\displaystyle \sum_{cycl}\sqrt[6]{ab^2c^3}\ge\sum_{cycl}\sqrt[30]{a^{9}b^{10}c^{11}}\right)$
- An Inequality with Cyclic Sums on Both Sides III $\left(\displaystyle \frac{x^6z^3+y^6x^3+z^6y^3}{x^2y^2z^2}\geq \frac{x^3+y^3+z^3+3xyz}{2}\right)$
- A Cyclic Inequality in Three Variables with a Variable Hierarchy $\left(\displaystyle 2(x^2y + y^2z + z^2x+xyz) \ge (x+y) (y+z) (z+x)\right)$
- Dan Sitaru's Cyclic Inequality In Three Variables $\left(\displaystyle \frac{(5a+b)(5b+c)(5c+a)}{27(a+8c)(b+8a)(c+8b)}\geq \frac{8abc}{(5a+4b)(5b+4c)(5c+a)}\right)$
- Dan Sitaru's Cyclic Inequality in Three Variables V $\left(\displaystyle (x+y+z)^2\le \sum_{cycl}\sqrt{(x^2+xy+y^2)(y^2+yz+z^2)}\right)$
- Dan Sitaru's Cyclic Inequality in Three Variables VII $\left(\displaystyle \frac{5x+3y+z}{5z+3y+x}+\frac{5y+3z+x}{5x+3z+y}+\frac{5z+3x+y}{5y+3x+z}\ge 3\right)$
- Problem 11867 from the American Mathematical Monthly $\displaystyle \left(\left(\frac{a^2}{a^2-ab+b^2}\right)^{\frac{1}{4}} + \left(\frac{b^2}{b^2-bc+c^2}\right)^{\frac{1}{4}} + \left(\frac{c^2}{c^2-ca+a^2}\right)^{\frac{1}{4}} \le 3\right)$
- An Inequality from the 1967 IMO Shortlist $\left(\displaystyle \frac{1}{a}+\frac{1}{b}+\frac{1}{c}\le\frac{a^8+b^8+c^8}{a^3b^3c^3}\right)$
- Birth of an Inequality $\displaystyle\left(3(a^2+b^2+c^2)^2\ge 24abc\sqrt[3]{abc}+\sum_{cyc}(a^2+b^2-c^2)^2\right)$
- A Simple Inequality in Three Variables $\displaystyle\left(\sum_{cyc}ac\left(\frac{1}{2a+b}+\frac{1}{2c+b}\right)\le \sqrt{3(a^2+b^2+c^2)}\right)$
- Cyclic Inequality in Three Variables by Marian Cucoanes $\displaystyle\left( \prod_{cycl}(\sqrt{(a+b)(a+c)}-\sqrt{bc})\ge abc\right)$
- Hung Viet's Inequality IV $\left(\displaystyle \sum_{cycl}\frac{1}{a+5b}\ge\sum_{cycl}\frac{1}{a+2b+3c}\right)$
- Cyclic Inequality with Arctangents $\left(\displaystyle 4\sum_{cycl}ab\cdot\arctan\frac{c}{b}\le\pi\sum_{cycl}a^2\right)$
- A Cyclic Inequality with Powers 2 through 7 $\left(\displaystyle \sum_{cycl}\frac{(a^7+b^7)^3}{(a^4+b^4)(a^5+b^5)(a^6+b^6)}\ge 3a^2b^2c^2\right)$
- A Long Cyclic Inequality of Degree 4 $\left(\displaystyle 4\cdot\sum_{cycl}ab\cdot\sum_{cycl}a-\left(\sum_{cycl}a\right)^3\ge\frac{\displaystyle 3\sum_{cycl}ab\left[4\sum_{cycl}ab-\left(\sum_{cycl}a\right)^2\right]}{\displaystyle \sum_{cycl}a}\right)$
- A Cyclic Inequality from the 6th IMO, 1964 $\left(\displaystyle \sum_{cycl}a^2(b+c-a)\le 3abc\right)$
- Inequality from Math Phenomenon $\left(\displaystyle \frac{a^{2n+1}}{\sqrt{bc}}+\frac{b^{2n+1}}{\sqrt{ac}}+\frac{c^{2n+1}}{\sqrt{ab}}\ge a^{2n}+b^{2n}+c^{2n}\right)$
- A Cyclic Inequality from Math Phenomenon $\left(\displaystyle \frac{1}{a(b+c)}+\frac{1}{b(c+a)}+\frac{1}{c(a+b)}\ge\frac{12}{(a+b+c)^2}\right)$
- Trigonometric Inequality with Integrals $\left(\small{\Omega(a,b)+\Omega(b,c)+\Omega(c,a)\le \sqrt{2}(a^2+b^2+c^2),\;\Omega(a,b)=\int_a^{2a}\int_b^{2b}|\sin (x-y)\cos (x+y)-\sin (x+y)|dxdy}\right)$
- A Cyclic Inequality in Three Variables by Uche E. Okeke $\left(\displaystyle \frac{(a+b)(b+c)(c+a)}{2}\ge abc+ \frac{(ab+bc+ca)^2}{a+b+c}\right)$
- Problem 4142 From Crux Mathematicorum $\displaystyle\left(\Bigr(1+\frac{a^2+b^2+c^2}{ab+bc+ca}\Bigr)^{\frac{(a+b+c)^2}{a^2+b^2+c^2}}\leq \Bigr(1+\frac{a}{b}\Bigr)\Bigr(1+\frac{b}{c}\Bigr)\Bigr(1+\frac{c}{a}\Bigr)\right)$
- Two-Sided Inequality by Dorin Marghidanu $\left(\displaystyle \sqrt{2}\le\sum_{cycl}\frac{b+c}{a+\sqrt{2(b^2+c^2)}}\le 2\right)$
- A Cyclic Inequality In Three Variables by Sorin Radulescu $\left(\displaystyle \left[\sum_{cycl}x(y-z)^2\right]^3\ge 54\prod_{cycl}x(y-z)^2\right)$
- Problem 1 from the 2017 Canada MO $\left(\displaystyle\left(\frac{a}{b-c}\right)^2+\left(\frac{b}{c-a}\right)^2+\left(\frac{c}{a-b}\right)^2\gt 2\right)$
- An Easy Cyclic Inequality And a Remark $\left(\displaystyle 1\le\frac{a}{a+b}+\frac{b}{b+c}+\frac{c}{c+a}\le 2\right)$
- A Cyclic Inequality from India In Three Variables And More $\left(\displaystyle \sum_{cycl}\sqrt{a^4+a^2b^2+b^4}+(6-\sqrt{3})\sum_{cycl}ab\ge 2\left(\sum_{cycl}a\right)^2\right)$
- Dan Sitaru's Cyclic Inequality in Three Variables VIII $\left(\displaystyle (xy+yz+zx)\sum_{cycl}\sqrt{x^2+xy+y^2}\le 3\sqrt{\prod_{cycl}(x^2+xy+y^2)}\right)$
- Hadamard's Determinant Inequalities and Applications I $\left((2-a-b-c+abc)^2\le (a^2+2)(b^2+2)(c^2+2)\right)$
- An Inequality with Two Triples of Variables II $\left(\displaystyle\small{ ax+by+cz+\sqrt{\left(\sum_{cycl}a^2\right)\left(\sum_{cycl}x^2\right)}\ge\frac{2}{3}\left(\sum_{cycl}a\right)\left(\sum_{cycl}x\right)}\right)$
- Two Cyclic Inequalities $\left(\displaystyle \frac{1}{a(1+b)}+\frac{1}{b(1+c)}+\frac{1}{c(1+a)}\ge\frac{3}{1+abc}\right),$ $\left(\begin{align} \displaystyle 3+(A+M+S)+\bigg(\frac{1}{A}+\frac{1}{M}+\frac{1}{S}\bigg)&+\bigg(\frac{A}{M}+\frac{M}{S}+\frac{S}{A}\bigg)\\&\ge\frac{3(A+1)(M+1)(S+1)}{AMS+1}\end{align}\right)$
- Ji Chen's Inequality $\left(\displaystyle (xy+yz+zx)\left(\frac{1}{(x+y)^2}+\frac{1}{(y+z)^2}+\frac{1}{(z+x)^2}\right)\ge\frac{9}{4}\right)$
- A Cyclic Inequality of Degree Four $\left(\displaystyle a^4b+b^4c+c^4a+2(a+b+c)\ge \sqrt{3}(ab+bc+ca)\right)$
- A Little More of Algebra for an Inequality, A Little Less of Calculus for a Generalization $\left(\displaystyle \sum_{cycl}(a-b)\cdot\left(\frac{a}{b}\right)^n\ge \sum_{cycl}(a-b)\cdot\frac{a}{b}\right)$
- A Cyclic Inequality in Three Variables XXVI $\left(\displaystyle \sum_{cycl}\frac{(x+y)^4+1}{(x+y)^6+1}\le\frac{1}{2}\left(\frac{1}{x}+\frac{1}{y}+\frac{1}{z}\right)\right)$
- Dorin Marghidanu's Inequality with Powers and Reciprocals $\left(\displaystyle \sum_{cycl}\frac{a}{a^2bc+b^4+c^4}\le\frac{1}{abc}\right)$
|Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73297261
