Concurrence in Equilateral Triangle
What Might This Be About?
Problem
$\ell$ is a line through the center $G$ of an equilateral $\Delta ABC;$ $A',$ $B',$ $C'$ are the midpoints of the sides $BC,$ $AC,$ and $AB;$ $A_1,$ $B_1,$ $C_1$ are the feet of th perpendiculars from the vertices of $\Delta ABC$ to $\ell.$
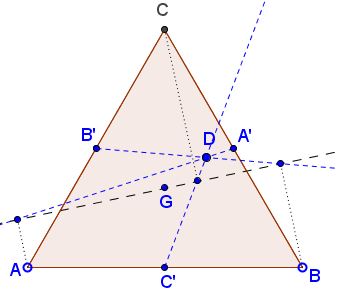
Prove that $A'A_1,$ $B'B_1,$ and $C'C_1$ are concurrent.
Proof
The solution makes use of complex numbers.
Assume $G$ is the center of the coordinate system, $\ell$ coincides with the $x-axis,$ and the vertices are given by $A=2e^{it},$ $B=2\omega e^{it},$ $C=2\omega^{2} e^{it},$ where $\omega = e^{\displaystyle i\frac{2\pi}{3}}.$ Note that $\omega$ satisfies the equation $\omega^{2}+\omega +1=0.$
We further find $A'=(\omega +\omega^{2} )e^{it}=-e^{it},$ $B'=-\omega e^{it},$ $C'=-\omega^{2}e^{it}.$
In addition, the projections on $\ell$ are given by their coordinates: $A_1 =(2\cos t,0),$ $\displaystyle B_1=(2\cos \left(t+\frac{2\pi}{3}\right), 0),$ $\displaystyle C_1=(2\cos \left(t+\frac{4\pi}{3}\right), 0).$
We thus in the position to write equations of the three lines:
$\displaystyle\begin{cases} A'A_1: & x\sin t - 3y\cos t =\sin 2t,\\ B'B_1: & x\sin\left(t+\frac{2\pi}{3}\right)-3y\cos\left(t+\frac{2\pi}{3}\right)=\sin 2\left(t+\frac{2\pi}{3}\right),\\ C'C_1: & x\sin\left(t+\frac{4\pi}{3}\right)-3y\cos\left(t+\frac{4\pi}{3}\right)=\sin 2\left(t+\frac{4\pi}{3}\right). \end{cases}$
The three lines concur iff
$\displaystyle\left|\begin{array}{ccc} \,\sin t & \cos t & \sin 2t\\ \sin\left(t+\frac{2\pi}{3}\right) & \cos\left(t+\frac{2\pi}{3}\right) & \sin 2\left(t+\frac{2\pi}{3}\right)\\ \sin\left(t+\frac{4\pi}{3}\right) & \cos\left(t+\frac{4\pi}{3}\right) & \sin 2\left(t+\frac{4\pi}{3}\right) \end{array} \right|=0.$
To verify that this is indeed so, add the last two rows to the first one whose terms will become
$\displaystyle Im\left(( 1+\omega +\omega^{2}) e^{it}\right),\; Re\left(( 1+\omega +\omega^{2}) e^{it}\right),\; Im\left(( 1+\omega^{2} +\omega^{4}) e^{2it}\right),$
each clearly equal to $0.$
Acknowledgment
The above problem has been posted by Dào Thanh Oai at the GeoGebra tube; the solution, posted at the CutTeKnotMath facebook page, is by Leo Giugiuc.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73294608
