The Eutrigon Theorem
Eutrigon is a triangle (in general scalene), with one angle equal \(60^{\circ}\). The side opposite the \(60^{\circ}\) angle is called (unfortunately in my view) the hypotenuse, the other two sides are called the legs.
The area of any eutrigon is equal to the sum of the areas of the equilateral triangles on its legs, minus the area of the equilateral triangle on its hypotenuse.
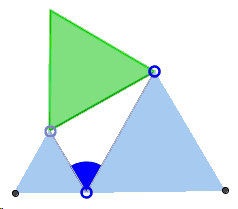
The applet below serves a dynamic illustration of a visual proof:
Let the legs of an eutrigon are \(a\) and \(b\) and the hypotenuse \(c\), \(A\) its area and \(A(x)\) the area of an equilateral triangle with side \(x\), then what we see in the applet can be expressed by two equations:
\(A(a+b)=A(a)+A(b)+2A\) and
\(A(a+b)=A(c)+3A\),
from which the theorem follows.
(There is a newer HTML5 version of this page.)
|Contact| |Front page| |Content| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny
73240740
