Further Properties of Peculiar Circles

Problem 2 from the 2015 Romanian TST (Team Selection Test) seniors dealt with circles tangent to the circumcircle $(ABC)$ of $\Delta ABC$ at vertices and the opposite side.

Let $r$ be the inradius of $\Delta ABC.$ Form circles $(O_a),(O_b),(O_c)$ tangent to the circumcircle $(ABC)$ at the vertices $A,B,C,$ respectively, and tangent to the sides opposite the corresponding vertices. The radii of the circles are denoted $R_a,R_b,R_c,$ respectively.

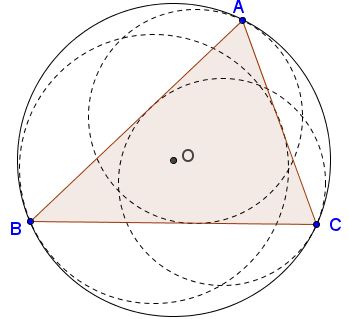
Dorin Andrica's problem, problem

Prove that

$\displaystyle \frac{1}{R_a}+\frac{1}{R_b}+\frac{1}{R_c}\le \frac{2}{r}.$

It is practically obvious that the condition that the circles in question touch the circumcircle is a red herring - it is not necessary for the above inequality to hold. However, Tran Quang Hung found additional properties that appear specific to those circles.The statements and the proofs below are Tran Quang Hung's. In the above notations,

$\displaystyle R_{a}=\frac{l_{a}^2}{2h_{a}},$ where $l_a$ is length of the bisector of the angle at $A$ and $h_a$ is altitude from $A.$
$\displaystyle\frac{1}{\sqrt{R_{a}\cdot r_{a}}}+\frac{1}{\sqrt{R_{b}\cdot r_{b}}}+\frac{1}{\sqrt{R_{c}\cdot r_{c}}}=\frac{2}{\sqrt{R\cdot r}},$ where $r_{a},r_{b},r_{c}$ are the exradii of $\Delta ABC.$
Prove that $\displaystyle\frac{1}{R_{a}}+\frac{1}{R_{b}}+\frac{1}{R_{c}}\ge \frac{4}{R}.$

Proof

It is well known that if $(Oa)$ touches $BC$ at $X_a,$ the foot of the angle bisector at $A.$

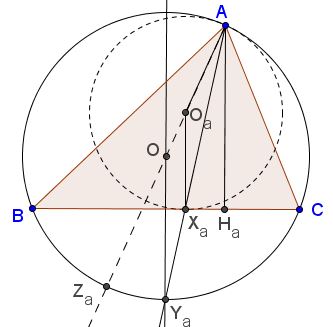
Indeed, assuming $X_a$ to be the point of tangency of $(O_a)$ and $BC,$ let $Y_a$ and $Z_a$ be the second intersections with $(ABC)$ of $AX_a$ and $AO,$ respectively. Then $\angle O_aAX_a=\angle O_aX_aA$ and $\angle Z_aO_aX_a=2\angle Z_aAY_a=\angle Z_aOY_a,$ which makes $OY_a\parallel O_AX_a$ and, therefore, $OY_a\perp BC,$ implying that $Y_a$ is the midpoint of the arc $BC$ and $AY_a$ the bisector of $\angle BAC.$

Let $M$ be the midpoint of $AX_a$ and let $O_{a}M$ cut $AH_a$ at $N.$

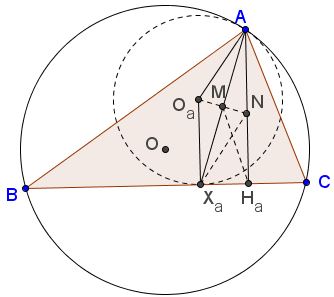
Then $ANDO_a$ is a rhombus. Now, isosceles triangles $NAX_a$ and $MAH_a$ are similar. Thus, $\displaystyle\frac{NA}{MA}=\frac{AD}{AH},$ i.e. $\displaystyle\frac{R_{a}}{l_{a}/2}=\frac{l_{a}}{h_{a}},$ and $\displaystyle R_{a}=\frac{l_{a}^2}{2h_{a}}.$
Note that $\displaystyle l_{a}^2=p(p-a)\frac{4bc}{(b+c)^2}$ where $p=(a+b+c)/2,$ $h_{a}=2S/a,$ $p-a=S/r_{a}$ so

$\displaystyle R_{a}=p(p-a)\frac{4bc}{(b+c)^2}\cdot\frac{1}{4S/a}=\frac{abc\cdot p}{r_{a}(b+c)^2}.$

From this, $\displaystyle\frac{1}{R_{a}\cdot r_{a}}=\frac{(b+c)^2}{abc\cdot p},$ or, $\displaystyle\frac{1}{\sqrt{R_{a}\cdot r_{a}}}=\frac{b+c}{\sqrt{abc\cdot p}}.$ Similarly, $\displaystyle\frac{1}{\sqrt{R_{b}\cdot r_{b}}}=\frac{c+a}{\sqrt{abc\cdot p}}$ and $\displaystyle\frac{1}{\sqrt{R_{c}\cdot r_{c}}}=\frac{a+b}{\sqrt{abc\cdot p}}.$ Thus,

$\begin{align}\displaystyle \frac{1}{\sqrt{R_{a}\cdot r_{a}}}+\frac{1}{\sqrt{R_{b}\cdot r_{b}}}+\frac{1}{\sqrt{R_{c}\cdot r_{c}}} &=\frac{4p}{\sqrt{abc\cdot p}}\\ &=4\sqrt{\frac{p}{abc}}\\ &=\frac{2}{\sqrt{R\cdot r}} \end{align}$

because $S=pr$ and $abc=4RS.$
We have $\displaystyle\frac{1}{\sqrt{2R_a\cdot r_{a}}}+\frac{1}{\sqrt{2R_b\cdot r_{b}}}+\frac{1}{\sqrt{2R_c\cdot r_{c}}}=\frac{2}{\sqrt{2R\cdot r}}.$ By Cauchy-Schwarz inequality,

$\begin{align}\displaystyle \frac{4}{2Rr}&=\bigg(\frac{1}{\sqrt{2R_a\cdot r_{a}}}+\frac{1}{\sqrt{2R_b\cdot r_{b}}}+\frac{1}{\sqrt{2R_c\cdot r_{c}}}\bigg)^2\\ &\le \bigg(\frac{1}{2R_a}+\frac{1}{2R_b}+\frac{1}{2R_c}\bigg)\bigg(\frac{1}{r_{a}}+\frac{1}{r_{b}}+\frac{1}{r_{c}}\bigg)\\ &=\bigg(\frac{1}{2R_a}+\frac{1}{2R_b}+\frac{1}{2R_c}\bigg)\cdot \frac{1}{r}. \end{align}$

It follows that $\displaystyle\frac{1}{R_{a}}+\frac{1}{R_{b}}+\frac{1}{R_{c}}\ge \frac{4}{R}.$ Now combine this with an earlier inequality to obtain

$\displaystyle\frac{2}{r}\ge\frac{1}{R_{a}}+\frac{1}{R_{b}}+\frac{1}{R_{c}}\ge\frac{4}{R}.$

What Is Red Herring
|Contact| |Front page| |Contents| |Geometry|

Copyright © 1996-2018 Alexander Bogomolny

72347834

Further Properties of Peculiar Circles

Proof

What Is Red Herring