A Cyclic Inequality in Three or More Variables

Problem

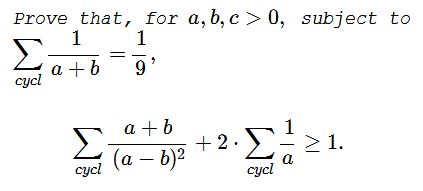
A Cyclic Inequality in Three or More Variables

Solution 1

We start with proving a lemma:

Prove that, for $a,b\gt 0,$

$\displaystyle \frac{a+b}{(a-b)^2}+\frac{1}{a}+\frac{1}{b}\ge \frac{9}{a+b}.$

By cross-multiplying, the above inequality reduces to

$a^4-8a^3b+18a^2b^2-8ab^3+b^4=(a^2-4ab+b^2)^2\ge 0.$

Equality occurs for $a=(2\pm\sqrt{3})b.$

The given inequality is an immediate consequence of the lemma:

$\displaystyle \begin{align} \sum_{cycl}\frac{a+b}{(a-b)^2}+2\cdot\sum_{cycl}\frac{1}{a}&=\sum_{cycl}\left[\frac{a+b}{(a-b)^2}+\frac{1}{a}+\frac{1}{b}\right]\\ &\ge\sum_{cycl}\frac{9}{a+b}=9\cdot\frac{1}{9}=1. \end{align}$

A little extra

The appearance of the above inequality and the proof suggest a little more general result:

Prove that, for an integer $n\ge 3\,$ and $n\,$ positive real numbers $a,b,c,\ldots,\,$ subject to $\displaystyle \sum_{cycl}\frac{1}{a+b}=\frac{1}{9},$

$\displaystyle \sum_{cycl}\frac{a+b}{(a-b)^2}+2\cdot\sum_{cycl}\frac{1}{a}\ge 1.$

It is interesting that by just looking at the constraint and the inequality, it is impossible to ascertain the number of variables involved. The situation changes if we replace "cyclic" sums with "symmetric" sums: symmetric in the sense that every variable is paired with any other exactly once. For $n=3\,$ the is no difference, but for $n=4,\,$ the cyclic pairing consists of the four pairs $(a,b),\,$ $(b,c),\,$ $(c,d),\,$ $(d,a),\,$ whereas the symmetric pairing consists of six pairs: $(a,b),\,$ $(a,c),\,$ $(a,d),\,$ $(b,c),\,$ $(b,d),\,$ $(c,d).\,$ If we write the inequality in the lemma for each of the six pairs and, subsequently, add them all up, we'll get

$\displaystyle \sum_{sym}\frac{a+b}{(a-b)^2}+3\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}+\frac{1}{d}\right)\ge 1,\,$

provided $\displaystyle \sum_{sym}\frac{1}{a+b}=\frac{1}{9}.$

In general,

Prove that, for an integer $n\ge 3\,$ and $n\,$ positive real numbers $a,b,c,\ldots,\,$ subject to $\displaystyle \sum_{sym}\frac{1}{a+b}=\frac{1}{9},$

$\displaystyle \sum_{sym}\frac{a+b}{(a-b)^2}+(n-1)\cdot\sum_{cycl}\frac{1}{a}\ge 1.$

It should be noted that in the above example, replacing, say, the pair $(a,b)\,$ with the pair $(b,a)\,$ would not change any of the expressions we encountered above, which allowed us to disregard half the pairs. In general, a symmetric sum in four variables of the expressions that depend on only two of them would include twelve terms, and, for five variables, twenty.

Solution 2

Using Bergstrom's inequality,

$\displaystyle \begin{align} \sum_{cycl}\frac{a+b}{(a-b)^2}+2\sum_{cycl}\frac{1}{a} &=\sum_{cycl}(a+b)\left(\frac{1}{(a-b)^2}+\frac{4}{4ab}\right)\\ &\ge \sum_{cycl}(a+b)\cdot\frac{(1+2)^2}{(a-b)^2+4ab}\\ &=9\sum_{cycl}\frac{1}{a+b}=1. \end{align}$

Acknowledgment

Dan Sitaru has kindly posted the problem of his from the Romanian Mathematical Magazine at the CutTheKnotMath facebook page. The post has been commented on by Leo Giugiuc who supplied the lemma from which the solution is immediate. Solution 2 is by Dieog Alvariz.

 

|Contact| |Up| |Front page| |Contents| |Algebra|

Copyright © 1996-2018 Alexander Bogomolny

71935743