Getting Ahead by Two Points

Getting Ahead by Two Point

Solution 1

It is easy to see that $\xi\,$ can only be 2,4,6;1,3,5;1,3,6;2,3,5;2,4,6. So, we divide six games into three rounds of two consecutive games each. If one of the players wins two games in the first round, the match ends with the probability of

\displaystyle \left(\frac{2}{3}\right)^2+\left(\frac{1}{3}\right)^2=\frac{5}{9};$\displaystyle \left(\frac{2}{3}\right)^2+\left(\frac{1}{3}\right)^2=\frac{5}{9}$;$\displaystyle 1-\left[\left(\frac{2}{3}\right)^2+\left(\frac{1}{3}\right)^2\right]=\frac{4}{9}$;$\displaystyle \left(\frac{2}{3}\right)\left(\frac{1}{3}\right)=\frac{2}{9}$;$\displaystyle 1-\left(\frac{2}{3}\right)\left(\frac{1}{3}\right)=\frac{7}{9}$.

Otherwise, the players tie with each other, earning 1;$0$;$1$;$2$ point(s) each, and the match enters the second round, the probability of this happening is \displaystyle \frac{4}{9};$\displaystyle \frac{2}{9}$;$\displaystyle \frac{3}{9}$;$\displaystyle \frac{4}{9}$;$\displaystyle \frac{5}{9}$

We have similar discussions for the second and the third rounds. Thus, $\displaystyle P(\xi =2)=\frac{5}{9},\,$ $\displaystyle P(\xi =4)=\frac{4}{9}\cdot\frac{5}{9}=\frac{20}{81},\,$ $P(\xi =6)=$\displaystyle \left(\frac{4}{9}\right)^2=\frac{16}{81};$\displaystyle \left(\frac{4}{9}\right)^2=\frac{16}{81}$;$\displaystyle \left(\frac{4}{9}\right)^3=\frac{64}{729}$;$\displaystyle \left(\frac{4}{9}\right)^2\cdot\frac{5}{9}=\frac{100}{729}$

Finally,

$E\xi=$2;2;4;6$\cdot\displaystyle\frac{5}{9}+$4;2;4;6$\cdot\displaystyle\frac{20}{81}+$6;2;4;6$\cdot\displaystyle\frac{16}{81}=\frac{266}{81}.$

Solution 2

Let $A_k\,$ denote the event that $A\,$ wins the $k\text{th}\,$ game, while $\overset{\_}{A_k}\,$ means that $B\,$ wins the game. Since $A_k\,$ and $\overset{\_}{A_k}\,$ are incompatible, and are independent of the other events, we have

$\displaystyle\begin{align}P(\xi=2)&=P(A_1A_2)+P(\overset{\_}{A_1}\overset{\_}{A_2})=\frac{5}{9},\\ P(\xi=4)&=P(A_1\overset{\_}{A_2}A_3A_4)+P(A_1\overset{\_}{A_2}\overset{\_}{A_3}\overset{\_}{A_4})+P(\overset{\_}{A_1}A_2A_3A_4)+P(\overset{\_}{A_1}A_2\overset{\_}{A_3}\overset{\_}{A_4})\\ &=2\left[\left(\frac{2}{3}\right)^2\left(\frac{1}{3}\right)+\left(\frac{1}{3}\right)^2\left(\frac{2}{3}\right)\right]\\ &=\frac{20}{81}\\ P(\xi=6)&=P(A_1\overset{\_}{A_2}A_3\overset{\_}{A_4})+P(A_1\overset{\_}{A_2}\overset{\_}{A_3}A_4)+P(\overset{\_}{A_1}A_2A_3\overset{\_}{A_4})+P(\overset{\_}{A_1}A_2\overset{\_}{A_3}A_4)\\ &=4\left(\frac{2}{3}\right)^2\left(\frac{1}{3}\right)^2\\ &=\frac{16}{81} \end{align}$

It follows that,

$E\xi=2\cdot$\displaystyle\frac{5}{9};$\displaystyle\frac{5}{9}$;$\displaystyle\frac{20}{81}$;$\displaystyle\frac{16}{81}$$+4\cdot$\displaystyle\frac{20}{81};$\displaystyle\frac{5}{9}$;$\displaystyle\frac{20}{81}$;$\displaystyle\frac{16}{81}$$+6\cdot$\displaystyle\frac{16}{81};$\displaystyle\frac{5}{9}$;$\displaystyle\frac{20}{81}$;$\displaystyle\frac{16}{81}$$\displaystyle =\frac{266}{81}.$

Solution 3

Amit Itagi came up with a graphical representation of the solution.

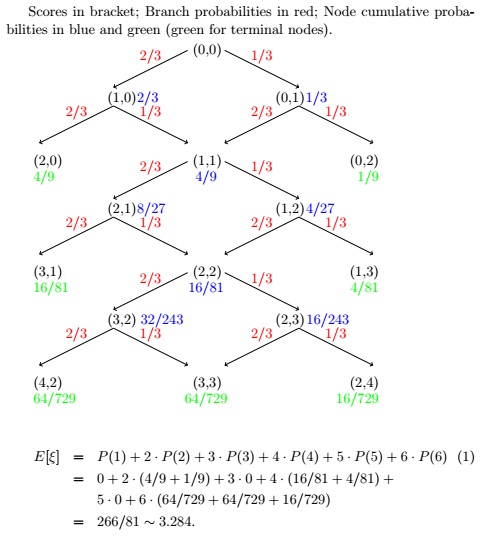
Getting Ahead by Two Point, solution 3

Solution 4

Nassim Nicholas Taleb has offered his interpretation:

Getting Ahead by Two Point, solution 4

Acknowledgment

Xiong Bin, Lee Peng Yee, Mathematical Olympiads in China (2009-2010). Problems and Solutions, World Scientific, 2013, #3

72505095