The Eyeball Theorem
What is this about?
The Eyeball Theorem
Let there be two circles $C(A, R_{A})$ and $C(B, R_{B}),$ one with center $A$ and radius $R_{A},$ the other with center $B$ and radius $R_{B}.$ Assume the tangents from $A$ to $C(B, R_{B})$ intersect $C(A, R_{A})$ at points $P$ and $Q,$ whereas the tangents from $B$ to $C(A, R_{A})$ intersect $C(B, R_{B})$ at points $R$ and $S.$
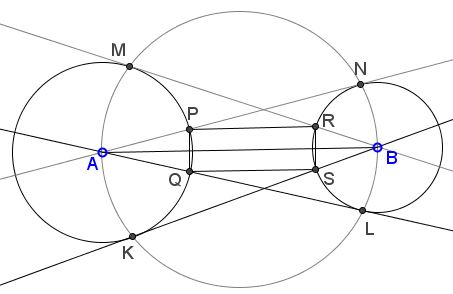
Then $PQ = RS.$
Proof 1

Angles $AMB$ and $ANB$ are right. Therefore, the quadrilateral $AMNB$ is cyclic, which implies
(1)
$\angle MAN = \angle MBN,$
since the two angles subtend the same arc. Now,
$\angle PMR = \angle MAP/2 = \angle MAN/2\\ \angle PNR = \angle RBN/2 = \angle MBN/2$
since the two angles are formed by a tangent and a chord. Along with (1) this gives
(2)
$\angle PMR = \angle PNR,$
which means that the quadrilateral $MPRN$ is also cyclic. From here,
(3)
$\angle NMR = \angle NPR,$
while, for a similar reason in the circle circumscribing the quadrilateral $AMNB,$
(4)
$\angle NAB = \angle NMB = \angle NMR,$
so that from (3) and (4) we conclude that
$\angle NPR = \angle NAB.$
In other words, $PR$ is parallel to $AB.$ Since $PQ \perp AB$ and $RS \perp AB$, and because of the symmetry in $AB,$ the quadrilateral $PQSR$ is a rectangle, so that $PQ = RS,$ indeed.
Proof 2
(NRich Program, University of Cambridge)

Let $C$ be the midpoint of $PQ.$ $C$ lies on $AB,$ and therefore $\Delta ACP$ is right. As such, it is similar to the right triangle $ANB,$ since the two share an angle. From the similarity of the triangles we derive the proportion $CP/AP = BN/AB,$ which means that
(5)
$CP = R_{A}R_{B}/AB,$ or $PQ = 2\cdot R_{A}R_{B}/AB.$
The latter expression is symmetric in $A$ and $B.$ Therefore also $RS = 2\cdot R_{A}R_{B}/AB.$ Q.E.D.
Proof 2'
We could have used (5) a little differently. Assume the circle $C(A, R_{A})$ is fixed as is the point $B.$ What happens when $R_{B}$ changes? Obviously (or, e.g., because all circles are similar), $DR,$ where $D$ is the center of $RS,$ changes linearly in proportion to $R_{B}.$ From (5), the same is true of $CP.$ The rates are bound to be the same, since when $R_{A} = R_{B},$ we trivially have $CP = DR.$ Therefore, the latter identity holds for any $R_{B}.$
Proof 3
(E. J. Barbeau, M. S. Klamkin, W. O. J. Moser, Problem 185)

Triangles $AMU$ and $BNU$ are similar, from which
(6)
$AM/AU = BN/BU$
On the other hand, $AM = AP$ and $BN = BR,$ such that (6) implies
(6')
$AP/AU = BR/BU$
Because of the symmetry of the configuration, $UV$ is orthogonal to $AB$ and is, therefore, parallel to $PQ$ and $RS.$ From here triangles $AUV$ and $APQ$ are similar as are triangles $BUV$ and $BRS.$ In combination with (6'), we obtain
$PQ/UV = AP/AU = BR/BU = RS/UV,$
which simply means that $PQ = RS.$
Proof 3'
Floor van Lamoen has suggested a different ending following (6'). The latter says that $PR$ is parallel to $AB$ as $P$ and $R$ divide the sides $AU$ and $BU$ of triangle $ABU$ in the same ratio. The same holds for $QS:$ $QS\parallel AB.$ It follows that $QSRP$ is a rectangle which proves the theorem.
Floor also came up with an entirely novel proof, which, although, lengthy brings to light additional features of the configuration, see Proof 4.
Proof 4
(By Floor van Lamoen, March 2006, private communication)
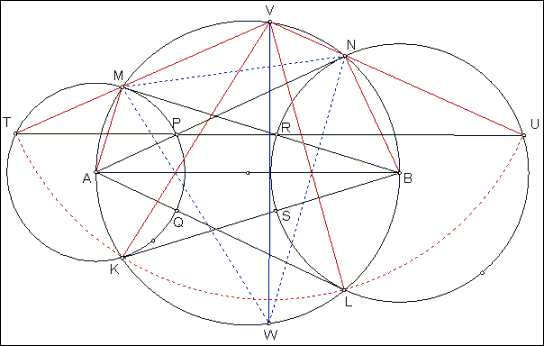
Extend $PR$ to $TU$ with $T$ on circle $A$ and $U$ on circle $B.$ Let $TM$ and $UN$ meet in $V.$
Note that $\angle MAN = \angle MBN$ and thus
$\angle VTU = \angle MTP = \frac{1}{2}\angle MAN = \frac{1}{2}\angle MBN = \angle RUN = \angle VUT,$
so triangle $TUV$ is isosceles with $VT = VU.$
We also see that
$\angle MBN = \angle VTU + \angle TUV$
so that $\angle MBN$ and $\angle NVM$ add to $180^{\circ}$, implying that $V$ lies on the circle with diameter $AB.$
Since inscribed angles $TVA$ and $KVA$ subtend equal arcs they are equal: $\angle TVA = \angle KVA$ and, similarly, $\angle UVB = \angle LVB$. By reflection through $AV$ and $BV$ respectively, we see that in fact $VT = VK = VL = VU.$
Let $W$ be the reflection of $V$ through $AB.$ $\Delta WMN$ is isosceles just as $\Delta KLV.$ Hence
$\angle WVN = \angle WMN = \angle WNM = \angle WVM,$
so $VW$ bisects angle $TVU,$ and $VW$ is perpendicular to $PR,$ and thus $PR$ is parallel to $AB.$ By symmetry $PQSR$ is a rectangle.
Proof 5
Talk about the length of a proof. Here is quite a short one also by Floor van Lamoen (private communication, March 28, 2006):
With a reference to the following diagram
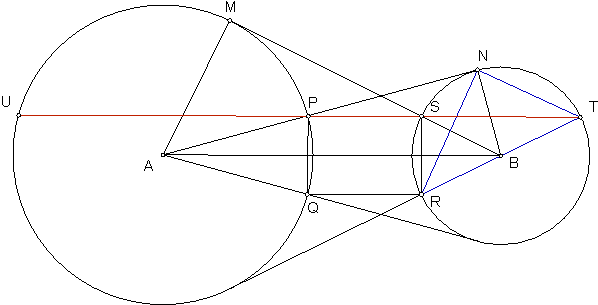
Note that $\angle RNA = \angle RTN$ by inscribed angles.
By isosceles $\Delta NBR,$ $\angle BNR = \angle BRN.$
So
$\begin{align} \angle RTN + \angle TRN &= \angle RTN + \angle BRN\\ &= \angle RNA + \angle BNR\\ &= 90^{\circ}. \end{align}$
This shows that $TR$ is a diameter of circle $(B).$ Hence $\angle RST$ is right. By symmetry $PQRS$ is a rectangle.
Remark: This proof makes an unwarranted assumption. Can you locate it?
Proof 6
Dao Thanh Oai has noticed that the Eyeball theorem is a consequence of a Japanese sangaku, concerning a convex quadrilateral $ABCD.$ If the quadrilateral is cyclic, the incenters of triangles $BCD,$ $ACD,$ $ABD,$ and $ABC$ form a rectangle. As applied to the Eyeball theorem, the quadrilateral in question is $MKLN$ whereas points $P,$ $Q,$ $R,$ and $S$ are the incenters of the corresponding triangles. I'll show that this is true of $P$ and $\Delta MKN.$ Three other points are treated similarly.
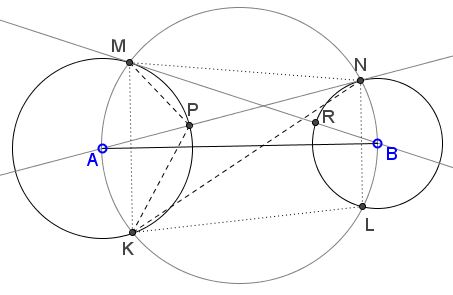
Indeed, in circle $(AB),$ $\angle MAN=\angle MKN.$ In circle $(A),$ $\angle MKP=\frac{1}{2}\angle MAP=\frac{1}{2}\angle MAN.$ It follows that $\angle MKP=\angle PKN$ as both equal half of $\angle MKN.$ This means that $KP$ is the angle bisector of $\angle MKN.$
Points $M$ and $K$ are symmetric in $AB,$ implying that arcs $MA$ and $AK$ of circle $(AB)$ are equal, so that too $\angle MNA=\angle ANK,$ which shows that NP is the bisector of $\angle MNK,$ making $P$ the incenter of $\Delta MKN.$
Remark
There is another interesting fact with ophthalmological connotations that I dubbed the Squinting Eyes Theorem. There are actually several more, see the list at the bottom of the page.
References
- E. J. Barbeau, M. S. Klamkin, W. O. J. Moser, Five Hundred Mathematical Challenges, MAA, 1995
- J. Konhauser, D. Velleman, S. Wagon, Which Way Did the Bicycle Go?, MAA, 1996, #33
- D. Wells, Curious and Interesting Geometry, Penguin Books, 1991
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny71752914
