When the vertices of a square are joined to the midpoints of the nonadjacent sides -- eight lines in all, a starry shape is formed in which one may discern the famous 3:4:5 triangle, known as the Egyptian or the rope stretchers triangle.

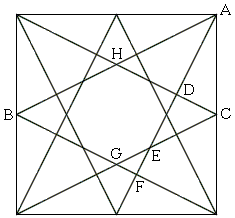
Thus the diagram provides an elegant way to form this triangle by paper folding.

In fact there are thirty two such triangles, eight of each type BFA, GFE, CDE, and HDA. A proof without words makes the assertion obvious for ΔBFA. The other triangles are clearly similar to the latter.

References

1. L. Bankoff, C. W. Trigg, The Ubiquitous 3:4:5 Triangle, Math Magazine, v 47, n 2 (Mar., 1974), pp. 61-70

Paper Folding Geometry

• Angle Trisection by Paper Folding
• Angles in Triangle Add to 180^o
• Broken Chord Theorem by Paper Folding
• Dividing a Segment into Equal Parts by Paper Folding
• Egyptian Triangle By Paper Folding
• My Logo
• Paper Folding And Cutting Sangaku
• Parabola by Paper Folding
• Radius of a Circle by Paper Folding
• An Interesting Example of Angle Trisection by Paperfolding