A Simple Integral of a Peculiar Function

Here is problem from Mathematics Magazine (Volume 91, 2018 - Issue 1) posed by D. M. Batinetu-Giurgiu and Neculai Stanciu. Solution is by Ulrich Abel.

There is a way to find this integral actually without computing integrals at all. Well, almost.

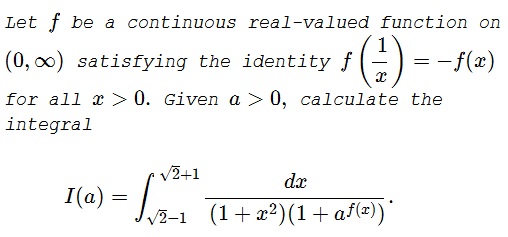
Let $f$ be a continuous real-valued function on $(0,\infty)$ satisfying the identity $\displaystyle f\left(\frac{1}{x}\right)=-f(x)$ for all $x\gt 0.$ Given $a\gt 0,$ calculate the integral

$\displaystyle I(a)=\int_{\sqrt{2}-1}^{\sqrt{2}+1}\frac{dx}{(1+x^2)(1+a^{f(x)})}.$

Solution 1

Using $(\sqrt{2}+1)(\sqrt{2}-1)=1,$ the change of variable $\displaystyle z=\frac{1}{t}$ shows that $I(a)=I(a^{-1}). From the identity

$\displaystyle \frac{1}{1+a^u}+\frac{1}{1+a^{-u}}=1,$

true for all $u\in\mathbb{R},$ we obtain

$\displaystyle \begin{align} I(a)&=\frac{I(a)+I(a^{-1})}{2}=\frac{1}{2}\int_{\sqrt{2}-1}^{\sqrt{2}+1}\frac{dx}{1+x^2}\\ &=\frac{\arctan(\sqrt{2}+1)-\arctan(\sqrt{2}-1)}{2}=\frac{\pi}{8}, \end{align}$

where the last identity follows from $\displaystyle \arctan 1=\frac{\pi}{4}$ and

$\displaystyle \arctan x-\arctan y=\arctan\left(\frac{x-y}{1+xy}\right),$

Curiously, the integral does not depend on $a.$

true for $xy\gt -1.$

Solution 2

(By Amit Itagi.)

$\displaystyle I(a)=\int_{x=\sqrt{2}-1}^{\sqrt{2}+1} \frac{dx}{\left(1+x^2\right)\left(1+a^{f(x)}\right)}.$

Making the substitution: $x=1/y$ (and $dx=-dy/y^2$),

$\displaystyle \begin{align} I(a)&=\int_{y=\sqrt{2}+1}^{\sqrt{2}-1}\frac{-dy}{y^2\left(1+\frac{1}{y^2}\right)\left(1+a^{f(1/y)}\right)}\\ &=\int_{y=\sqrt{2}-1}^{\sqrt{2}+1}\frac{dy}{\left(1+y^2\right)\left(1+a^{-f(y)}\right)}. \end{align}$

Adding the two expressions for $I$,

$\displaystyle \begin{align} I(a)&=\frac{1}{2}\int_{x=\sqrt{2}-1}^{\sqrt{2}+1} \frac{dx}{\left(1+x^2\right)}\left[\frac{1}{1+a^{f(x)}}+\frac{1}{1+a^{-f(x)}}\right] \\ &=\frac{1}{2}\int_{x=\sqrt{2}-1}^{\sqrt{2}+1}\frac{dx}{\left(1+x^2\right)} = \frac{1}{2}\left[\tan^{-1}(\sqrt{2}+1)-\tan^{-1}(\sqrt{2}-1)\right]. \end{align}$

Let $p=\tan^{-1}(\sqrt{2}+1)$ and $q=\tan^{-1}(\sqrt{2}-1)$. Thus, $\tan p=\sqrt{2}+1$ and $\tan q=\sqrt{2}-1$.

$\displaystyle \tan(p-q)=\frac{\tan p - \tan q}{1+\tan p \tan q}=1.$

Both $p$ and $q$ have to belong to the same branch of $\tan^{-1}$. Thus, $p-q=\pi/4$.

Thus, $I=\frac{\pi}{8}$.

71212790