A Triple Integral Inequality
Problem
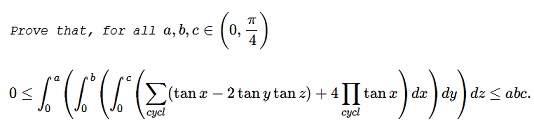
Solution
Believe it or not,
$\displaystyle\begin{align} T&=\sum_{cycl}(\tan x-2\tan y\tan z)+4\prod_{cycl}\tan x\\&=\frac{1}{2}\left(1-\prod_{cycl}\left(1-2\tan x\right)\right). \end{align}$
Assuming $a,b,c\in \left(0,\displaystyle\frac{\pi}{4}\right),\,$ $0\le T\le 1.\,$ Thus,
$\displaystyle\begin{align} 0&\le\small{\int_{0}^{a}\left(\int_0^b\left(\int_0^c\left(\sum_{cycl}(\tan x-2\tan y\tan z)+4\prod_{cycl}\tan x\right)dx\right)dy\right)dz}\\& \le \int_{0}^{a}\int_{0}^{b}\int_{0}^{c}1dxdydz\\& =abc. \end{align}$
Acknowledgment
Dan Sitaru has kindly posted the above problem (from the Romanian Mathematical Magazine) at the CutTheKnotMath facebook page. The solution is by Leo Giugiuc.
- A simple integral, I
- A simple integral, II
- A simple integral, III
- Another simple integral
- Yet Another Simple Integral
- Integral in Normal Distribution
- Sum Without Adding
- An Integral Inequality from the RMM
- Another Integral Inequality from the RMM
- A Triple Integral Inequality
- An Integral from the RMM
- An Integral Identity with Two Functions
- A Simple Integral of a Peculiar Function
![]()
|Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73289136
