Integral Is Area

I learned of this example from Kunihiko Chikaya's post on facebook. The task is to compute the integral

$\displaystyle\int_{a}^{b}\sqrt{(x-a)(b-x)}dx.$

The solution is to recollect the geometric meaning of the definite integral.

Compute the followng integral

$\displaystyle\int_{a}^{b}\sqrt{(x-a)(b-x)}dx.$

Solution

Let $y=\sqrt{(x-a)(b-x)}.$ For $y\gt 0,\;$ this is equivalent to $\displaystyle\left(x-\frac{a+b}{2}\right)^2+y^2=\left(\frac{b-a}{2}\right)^2\;$ which is a semicircle with center at $\displaystyle (\frac{a+b}{2},0)\;$ and radius $\displaystyle\frac{b-a}{2}:$

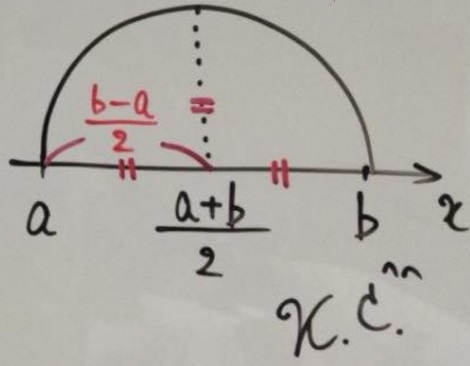
integral is area

Thus, the integral is half the area of a circle with radius $\displaystyle\frac{b-a}{2}:$

$\displaystyle\frac{1}{2}\pi\left(\frac{b-a}{2}\right)^2=\frac{\pi (b-a)^2}{8}.$

A simple integral, I
A simple integral, II
A simple integral, III
Another simple integral
Yet Another Simple Integral
Integral in Normal Distribution
Sum Without Adding
An Integral Inequality from the RMM
Another Integral Inequality from the RMM
A Triple Integral Inequality
An Integral from the RMM
An Integral Identity with Two Functions
A Simple Integral of a Peculiar Function

73362134