Equilateral Triangle on Three Lines

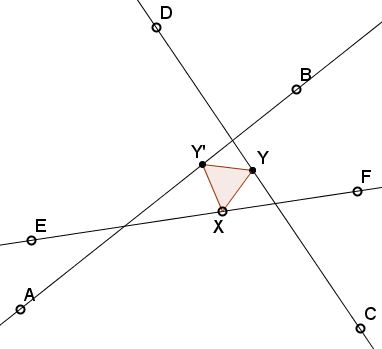
Choose an arbitrary point on one of the line, say $X$ on $EF.$ Rotate $CD$ around $X$ $60^{\circ}$ into $C'D'.$ Let $Y'$ be the intersection of $C'D'$ with $AB$ and $Y$ the point that was mapped into $Y'$ by the rotation.

Then $\Delta XYY'$ is equilateral and $X\in EF,$ $Y'\in AB,$ and $Y\in CD.$

In general, the solution is not unique, e.g.,

The construction is classic. For three parallel lines (when the solution is unique up to isomorphism), it can be found in I. M. Yaglom, Geometric Transformations I (MAA, 1962), problem 18. The latter problem has been discussed elsewhere.