Viviani in Isosceles Triangle: What is it?
A Mathematical Droodle
| What if applet does not run? |
|Activities| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander BogomolnyViviani in Isosceles Triangle
The applet attempts to illustrate the following proposition:
The sum of distances of a point in the base of an isosceles triangle to the sides does not depend on the position of the point on the base.
| What if applet does not run? |
For the proof, let PA'||AC, with A'∈AB. Draw BT'⊥AC and denote S' the intersection of BT' and BS'. Then, since BA'P is isosceles,
PS + PT = BS' + S'T' = BT',
the altitude from B to side AC, which is independent of the position of P.
This statement leads directly to Viviani's theorem:
The sum of distances of a point inside an equilateral triangle or on one of its sides equals the length of its altitude.
With the reference to the diagram below,
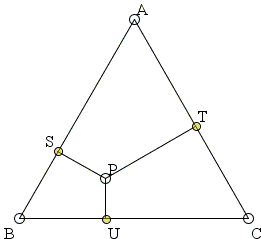
Viviani's theorem asserts that the sum PS + PT + PU is indendent of P.
Draw BaCa||BC:

As we just proved, in the isosceles ΔBaACa, the sum PS + PT is independent of the position of P on BaCa. Since BaCa||BC, this is also true of PU. Therefore, for P∈BaCa, the sum
Since ΔABC is equilateral the same holds for P on AbCb||AC:
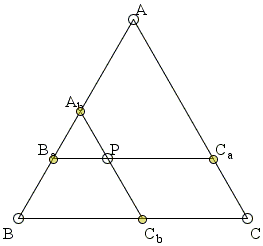
It follows that the sum PS + PT + PU is independent of the position of P on the union of the two lines. Now, when P glides over BaCa, AbCb sweeps the whole of ΔABC, showing that the sum remains the same for any position of P inside ΔABC, thus proving Viviani's theorem.
For another proof, simply observe that
Area(ΔABP) + Area(ΔACP) = Area(ΔABC).
Writing that explicitly we obtain
AB·PS/2 + AC·PT/2 = AC·BT'/2,
and, taking into account that AB = AC,
PS + PT = BT' = const.
|Activities| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73296324
