Carpets in Right Triangle

By the fundamental property of angle bisectors,

$\displaystyle \frac{AF}{BF}=\frac{AC}{BC}$ and $\displaystyle \frac{AE}{EH}=\frac{AC}{CH}=\frac{BC}{AC},$ the latter since triangles $HCA$ and $ABC$ are similar.

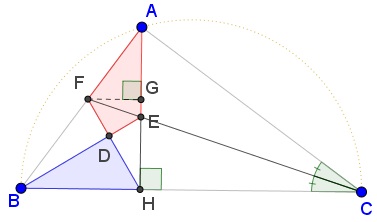
Let $FG\perp AH,\,$ $G$ on $AH.$ We are to prove that $[\Delta AFH]=[\Delta BEH].$ Indeed,

$\displaystyle \begin{align} \frac{[\Delta AFH]}{[\Delta BEH]} &= \frac{FG\cdot AH}{BH\cdot EH}=\frac{FG}{BH}\cdot\frac{AH}{EH}\\ &=\frac{AF}{AB}\cdot\left(\frac{AE}{EH}+1\right)=\frac{AF}{AF+BF}\cdot\left(\frac{AE}{EH}+1\right)\\ &=\frac{\displaystyle 1}{\displaystyle \frac{BC}{AC}+1}\cdot\left(\frac{AC}{CH}+1\right)\\ &=\frac{AC}{AC+BC}\cdot\left(\frac{BC}{AC}+1\right)\\ &=\frac{AC}{AC+BC}\cdot\frac{AC+BC}{AC}=1. \end{align}$

Now we may continue in two ways. A longer one, with a reference to Carpets theorem, and another - more direct.

$\Delta ABH=\Delta AFH\,\cup\,\Delta BFH=\Delta ABE\,\cup\,\Delta BEH.$ Since $[\Delta AFH]=[\Delta BEH],$ we have, by the Carpets theorem,

$\begin{align}[AEDF]&=[\Delta AFH \cap \Delta ABE]=[\Delta BFH \cap \Delta BEH]\\ &=[\Delta BDH].\end{align}$

More directly,

$\begin{align}[AEDF]&=[\Delta AFH \setminus \Delta DEH]=[\Delta BEH \setminus \Delta DEH]\\ &=[\Delta BDH].\end{align}$

The problem was posted at the Peru Geometrico facebook group by another group, Problema de Academia. More solutions could be found at the posted comments.