An Inequality with Just Two Variables IV
Problem
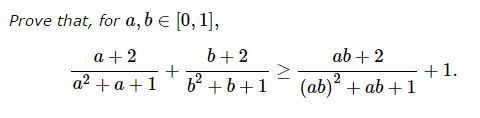
Solution 1
If $a=2\,$ then the inequality becomes $\displaystyle 2+\frac{b+2}{b^2+b+1}\ge 2+1\,$ which is equivalent to $1\ge b^2,\,$ which is true, due to $b\in [0,1].\,$ Similarly, for $b=0.\,$ So assume $a,b\in (0,1].$
Consider the function $f:\,(-\infty,0]\to\mathbb{R},\,$ defined by $\displaystyle f(x)=\frac{e^x+2}{a^{2x}+e^x+1}.\,$ We have $\displaystyle f'(x)=-\frac{e^x(e^{2x}+e^x+1)}{(e^{2x}+e^x+1)^2}\,$ and $\displaystyle f''(x)=\frac{e^x(e^x-1)(e^{3x}+8e^{2x}+8e^x+1)}{(e^{2x}+e^x+1)^3}\le 0\,$ for $x\in (-\infty,0].\,$ It follows that $f\,$ is concave on $(-\infty,0].\,$ Denote $x=\ln a\,$ and $y=\ln b.\,$ Then $x+y\in [x+y,0]\,$ and $x+y=0+(x+y),\,$ making Karamata's inequality applicable: $f(x)+f(y)\ge f(0)+f(x+y),\,$ i.e.,
$\displaystyle\frac{a+2}{a^2+a+1}+\frac{b+2}{b^2+b+1}\ge \frac{ab+2}{(ab)^2+ab+1}+1.$
Solution 2
$\displaystyle\begin{align} &\frac{a+2}{a^2+a+1}+\frac{b+2}{b^2+b+1}- \frac{ab+2}{(ab)^2+ab+1}-1\\ &\qquad\qquad=\frac{1-a^2}{a^2+a+1}+\frac{1-b^2}{b^2+b+1}- \frac{1-(ab)^2}{(ab)^2+ab+1}-1\\ &\qquad\qquad=\frac{(1-a)(1-b)(1-ab)(1+a+ab)(1+b+ab)}{(a^2+a+1)(b^2+b+1)[(ab)^2+ab+1]}\\ &\qquad\qquad\ge 0, \end{align}$
for $a,b\in [0,1].$
Expansion
Let $n\,$ be an integer, $n\ge 2,\,$ and let $a_k\in [0,1],\,$ $k\in\overline{1,n}.\,$ Then
$\displaystyle\sum_{k=1}^n\frac{a_k+2}{a_k^2+a_k+1}\ge\frac{\displaystyle\prod_{k=1}^na_k+2}{\displaystyle\prod_{k=1}^na_k^2+\displaystyle\prod_{k=1}^na_k+1}+n-1.$
The proof is by induction, with the original statement serving as the induction base, while the inductive step proceeds, with setting $\displaystyle a\prod_{k=1}^na_k\,$ and $b=a_{n+1}.$ We have
$\displaystyle\sum_{k=1}^n\frac{a_k+2}{a_k^2+a_k+1}\ge\frac{\displaystyle\prod_{k=1}^na_k+2}{\displaystyle\prod_{k=1}^na_k^2+\displaystyle\prod_{k=1}^na_k+1}+n-1$
and
$\displaystyle\frac{\displaystyle\prod_{k=1}^na_k+2}{\displaystyle\prod_{k=1}^na_k^2+\displaystyle\prod_{k=1}^na_k+1}+\frac{a_{n+1}+2}{a_{n+1}^2+a_{n+1}+1}\ge \frac{\displaystyle\prod_{k=1}^{n+1}a_k+2}{\displaystyle\prod_{k=1}^{n+1}a_k^2+\displaystyle\prod_{k=1}^{n+1}a_k+1}+1.$
Adding the two completes the induction.
Acknowledgment
The problem above has been kindly posted to the CutTheKnotMath facebook page by Leo Giugiuc; the result (and Solution 1) is due to Leo Giugiuc, Droberta Turnu Severin, Romania and (together with Solution 2) to Michael Rosenberg (Tel-Aviv, Israel). Originally, this was the basis for their inductive proof of a more general statement (Expansion).
Inequalities in Two Variables
- An Inequality with Just Two Variable $\left(\displaystyle\left(\frac{2ab}{a+b}+\sqrt{\frac{a^2+b^2}{2}}\right)\left(\frac{a+b}{2ab} + \sqrt{\frac{2}{a^2+b^2}}\right) \le \frac{(a+b)^2}{ab}\right)$
- An Inequality with Just Two Variable II $\left(\displaystyle\small{\left(\frac{2ab}{a+b}+\sqrt{ab}+\frac{a+b}{2}\right)\left(\frac{a+b}{2ab} + \frac{1}{\sqrt{ab}}+\frac{2}{a+b}\right) \le 5 +2 \left(\frac{a}{b}+\frac{b}{a}\right)}\right).$
- An Inequality with Just Two Variable III $\left(\displaystyle\frac{a}{b\sqrt{2}}+\frac{b\sqrt{2}}{a}+2\left(\frac{\sqrt{a^2+b^2}}{b}+\frac{b}{a^2+b^2}\right)\ge \frac{9\sqrt{2}}{2}\right)$
- An Inequality with Just Two Variables IV
- An Inequality with Just Two Variables V $\left(\displaystyle \left(\ln\left(\frac{1}{2a}+\frac{1}{2b}\right)\right)^{a+b}\ge \left(\ln\frac{1}{a}\right)^b\left(\ln\frac{1}{b}\right)^a\right)$
- An Inequality with Just Two Variables VI $\left(|x-y|(x+1)(y+1)\le 2\right)$
- An Inequality with Just Two Variable VII $\left(\displaystyle (x^3+y^3)^3(x^2-xy+y^2)\ge x^2y^2\sqrt{xy}(x^2+y^2)^3\right)$
- An Inequality with Just Two Variable VIII $\left(\displaystyle \frac{a^2}{b+2}+\frac{b^3}{a+2}+(2-a)b^2\le 12\right)$
- The power of substitution III: proving an inequality with two variables $\left(\displaystyle\frac{1}{\sqrt{1+a^2}}+\frac{1}{\sqrt{1+b^2}}\le\frac{2}{\sqrt{1+ab}}\right)$
- Simple Yet Uncommon Inequalities with Absolute Value $\left((|x|-|y|)^2\le |x^2-y^2|,\,|\sqrt{|x|}-\sqrt{|y|}|\le\sqrt{|x-y|}\right)$
- An Inequality with Just Two Variable And an Integer $\small{\left(\displaystyle \Bigr(\frac{a}{b^n}+\frac{b}{a^n}\Bigr)\Bigr(\frac{a^n}{b}+\frac{b^n}{a}\Bigr)\Bigr(\frac{a^n}{b^n}+\frac{b}{a}\Bigr)\Bigr(\frac{b^n}{a^n}+\frac{a}{b}\Bigr)\geq 8\left(\sqrt{\displaystyle\left(\frac{a}{b}\right)^{n-1}}+\sqrt{\displaystyle\left(\frac{b}{a}\right)^{n-1}}\right)\right)}$
- An Inequality with Exponents $\left(\displaystyle b^b\cdot e^{a+\frac{1}{a}}\ge 2e^b\right)$
- Problem 790 from Pentagon: an Inequality in Two Variables $\left(\displaystyle\ln \left|\left(\frac{2+\sin 2b}{2+\sin 2a}\right)\right|\leq \frac{2\sqrt{3}}{3}(b-a)\right)$
- An Inequality with Two Variables from Awesome Math $\left(\displaystyle \frac{6ab-b^2}{8a^2+b^2}\lt\sqrt{\frac{a}{b}}\right)$
![]()
|Contact| |Front page| |Contents| |Algebra|
Copyright © 1996-2018 Alexander Bogomolny73336161
