Squares and Straight Tetrominoes
Here is a problem from the 2009-2010 International Internet Mathematics Olympiad run by the Ariel University Center of Samaria (Israel).
Workers were supposed to cover the floor of a rectangular room with tiles of two different sizes: 2×2 and 1×4 (the area of the floor is such that it can be covered completely by a certain combination of the two kinds of tiles). The necessary amount of tiles was obtained, but during their transportation to the destination three tiles of the size 2×2 were broken. However, there were three spare 1×4 tiles. Prove that it is now impossible to cover the entire area of the floor with the available tiles. (Cutting tiles is not allowed.)
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander BogomolnyWorkers were supposed to cover the floor of a rectangular room with tiles of two different sizes: 2×2 and 1×4 (the area of the floor is such that it can be covered completely by a certain combination of the two kinds of tiles). The necessary amount of tiles was obtained, but during their transportation to the destination three tiles of the size 2×2 were broken. However, there were three spare 1×4 tiles. Prove that it is now impossible to cover the entire area of the floor with the available tiles. (Cutting tiles is not allowed.)
Solution
Imagine the floor covered by a rectangular grid of 1×squares. Color each odd grid square in every odd row:
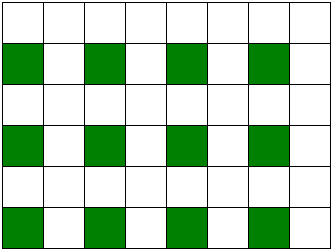
It should be clear that every 2×2 square, align with a grid, covers exactly one painted 1×1 square. On the other hand, a 1×4 pieces always covers an even number of painted squares. Three broken squares would have covered 3 - an odd number - of painted squares, something the 1×4 pieces could not do.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73299024
