Non-Euclidean Geometries, Models

Non-Euclidean Geometries
As Good As Might Be

In 1823, Janos Bolyai wrote to his father: "Out of nothing I have created a new universe." By which he meant that starting from the first 4 of Euclid's postulates and a modified fifth, he developed an expansive theory that, although quite unusual, did not seem to lead to any logical contradiction. Gauss expressed his conviction in consistency of the theory he had in mind in a letter in 1824. However, hesitant of the public reaction to the idea that, by the side of Euclidean, there is another geometry, he never published anything on the subject.

From our perspective, the situation was exactly the same as with Euclidean geometry. Euclid built an axiomatic theory by deriving a lot of theorems from his five postulates. No one had ever proved that continuing in Euclid's footstep would not lead to a contradiction. However, a 2000 year history elevated Elements on a pedestal of infallibility. Kant even stipulated that the universe had been built according to Euclid. Lobachevsky was quite aware of the problem and in later publications tried without success to redefine the notions of line and plane.

A breakthrough came in 1868 with the publication of Saggio di interpretazoine della geometria non euclidea by the Italian mathematician Eugenio Beltrami (1835-1900). Beltrami discovered that Lobachevsky's geometry admits an interpretation in terms of Euclidean geometry. From here it follows that if Lobachevsky's geometry leads to a contradiction, Euclidean geometry is as well contradictory. In other words, consistency of Euclidean geometry implies consistency of the geometry of Lobachevsky. The construction is now known as Beltrami-Klein model of Lobachevsky geometry. Sometimes it's called the projective model because it may be extended to conic sections and builds on an unusual usage of usual straight lines.

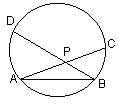
The Beltrami-Klein model considers the region strictly inside a circle as a model of a plane. Note that the region does not include points on the circle itself. Lines are chords connecting points on the circle with the endpoints excluded. (For lines should belong to the plane.) Line AC and BD pass through point P and both are parallel to AB. Furthermore, all the lines between AC and BD (inside the angles APD and BPC) are also parallel to AB. It's very easy to verify that the first four Euclid's postulates hold but there is infinitely many lines through a given point and parallel to a given line.

There might be an uneasy feeling when calling a segment a line and a disk a plane. Poincare suggested a dictionary method. In a dictionary, most of the entries have several interpretations. Imagine a being that uses the same words as we do but imputes to them a different meaning. For example, in Flatland, birds do not have wings. The grammar is also the same. So that the sentences we earthlings construct make good sense to these other people. The meaning is different, however. And our intuitions vary.

The idea of axiomatic method so much associated with Euclid's Elements is in logical derivation of one statement from another. Intuition is not required. Beltrami's model easily passes the test of deduction. It's up to us now to adjust our intuition. Howard Eves called this development in the history of mathematics liberation of geometry.

The situation with the Beltrami-Klein model is somewhat more complex than might appear at the first glance. The assumption that lines have infinite extent was found to contradict the replacement (B) for the fifth postulate. Everyone before Riemann held an implicit belief in the infinitude of the line's length. In Lobachevsky's geometry, the lines are assumed to be infinite in extent. In the model, however, we deal with finite segments. Also, it's not quite so clear that the third Euclid's postulate does indeed hold. Can we draw circles of arbitrary radius with arbitrary center?

In so far as we agree that the disk represents a model of the plane, we may select an arbitrary point as a center for a future circle. The tricky (and not obvious) part of the model construction is in definition of the distance measurements. Lobachevsky's geometry is of infinite extent. To fit it into a bounded region, the distance function must be changed. It is so modified as to have all points of the disk lie at an infinite distance from the defining circle. Euclidean segments of constant length grow in the non-Euclidean length when moved towards that circle.

Let a line that passes through two points P and Q crosses (in the Euclidean sense) the circle at points S and T. Then the distance between P and Q is defined by the following strangely looking formula:

dist(P, Q) = log((|QS|*|PT|)/(|PS|*|QT|))

where vertical bars denote Euclidean length of a line segment. It's a good exercise to check that thus defined function really satisfies the three metric axioms. It's also easily seen that if Q moves away from a fixed P staying on the same line, the distance between the two points grows without bound. In this metric, the length of the line ST is indeed infinite.

71542787