Similarity and the Parallel Postulate

Scott E. Brodie
4/19/02

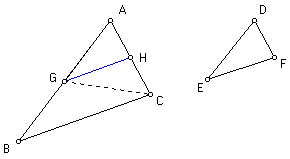
Suppose there exist similar, unequal triangles. Let ABC be the larger one, DEF the smaller. Then we can copy triangle DEF onto triangle ABC: on segment AB, mark point G so that AG = DE; on segment AC, mark point H so that AH = DF. Since angle A equals angle D, triangle AGH equals triangle DEF.

Of course, angle AGH and angle HGB sum to two right angles, as they are supplementary, as do angles AHG and GHC. But angle AGH equals angle B, and Angle AHG equals angle F, so the angles of the quadrilateral BGHC sum to four right angles.

Draw a diagonal of quadrilateral BGHC, say GC, cutting the quadrilateral into two triangles. The sum of the angles of the two triangles is clearly the sum of the angles of the quadrilateral. But, since according to one of Legendre's lemmas, the sum of the angles of any triangle is at most two right angles, it follows that the sum of the angles of each of the subsidiary triangles BGC and CGH must in fact equal two right angles.

We have thus exhibited a triangle (in fact two of them) whose angles sum to two right angles. We have shown previously that this is equivalent to Euclid's Parallel Postulate.

QED.

• Non-Euclidean Geometries, Introduction
• The Fifth Postulate
• The Fifth Postulate is Equivalent to the Pythagorean Theorem
• The Fifth Postulate, Attempts to Prove.
• Similarity and the Parallel Postulate
• Non-Euclidean Geometries, Drama of the Discovery.
• Non-Euclidean Geometries, As Good As Might Be.
• The Many-Faced Geometry
• The Exterior Angle Theorem - an appreciation
• Angles in Triangle Add to 180°

|Contact| |Front page| |Contents| |Geometry|

Copyright © 1996-2018 Alexander Bogomolny