Monge's Theorem of three circles and common tangents
Let there be three circles of different radii lying completely outside each other. To exclude a trivial case, assume also that their centers are not collinear, i.e. the three centers do not lie on the same straight line. Under these conditions, six external tangents to two of the three circles, taken pairwise, intersect at three points. Then the three points are collinear.
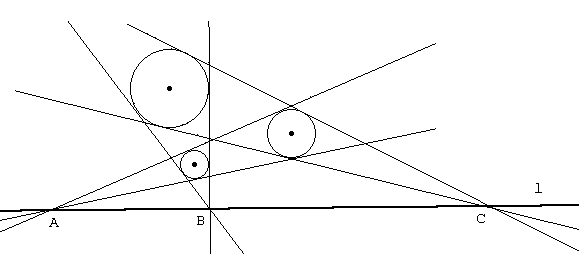
This is known as Monge's Circle Theorem.
This problem is almost on a par with the 4 Travelers and 9 dots problems. It has a "3D solution" but can also be solved without leaving the plane. D.Wells tells of John Edson Sweet, an engineer, whose reaction to the above diagram was, "Yes, that is perfectly self-evident."
Solutions|Contact| |Front page| |Contents| |Geometry| |Up|
Copyright © 1996-2018 Alexander Bogomolny
Solution 1
The following is the argment put forth by John Edson Sweet [Wells, p 172-174, Gardner, p. 119].
Imagine having three different spheres lying on a plane, say plane #1. In one particular case where there exists a second plane, plane #2, tangent to the three spheres but covering them from above the proof is particularly intuitive. For then planes #1 and #2 are bound to have a common line l, the line of their intersection. The bisector plane of the solid angle formed by planes #1 and #2 passes through the centers of all three spheres. This will be the plane, plane #3, depicted at the top of the page. By definition, plane #3 passes through l.
On the other hand, any two of the spheres can be inscribed into a cone. Any plane (externally) tangent to two spheres will also be tangent to the enclosing cone. Therefore, planes #1 and #2 are tangent to each of the three cones. Now, a plane tangent to a cone necessarily contains its vertex. Finally, vertices of the cones belong to both planes #1 and #2, hence to the line l, hence to the plane #3.
Note now that plane #3 intersects the cones along the common tangent lines as on the diagram.
Hubert Shutrick simplified the proof by reducing the number of planes to two.
Solution 1'
Consider the three circles as equatorial great circles of three spheres. There is a common tangent plane touching each in the northern hemispheres (plane #2 above) and its intersection with the given plane (plane #3 above) is the required line because the lines joining the pairs of contact points are generators of the tangential cones (see Solution 1) that also include the original tangents as generators.
Another 3D solution has been suggested by Nathan Bowler:
Solution 2
Construct 3 right cones on the three circles as bases, with equal apex angles. An external common tangent two a pair of the circles belongs to the plane defined by the cone generators at the points of tangency. This is because the generators form the same angle with the plane of the circles. There are three pairs of such common tangent planes. They intersect in three lines A', B' and C' corresponding to the three points A, B and C in the theorem. Now, A' and B' intersect at the apex of one of the cones. Similarly, B' and C' intersect and so do C' and A'. Thus A', B' and C' are coplanar, and so A, B and C are collinear.
Solution 3
This solution is due to Stuart Anderson.
Name the three circles a, b, and c, so that the common tangents of a and b meet at C, those of a and c at B, and those of b and c at A. We assign each circle a mass
Now it is a known fact that the coordinates of the center of mass of a combined system is just the weighted average of the coordinates of the centers of each system separately, and hence is collinear with them. If I choose systems
By the way, this also shows why you can't prove the theorem for internal tangent pairs: if all the masses are positive, you can't combine two pairs of circles to get the remaining pair, because no cancellation is possible. The theorem does work again with one external tangent pair and two internal tangent pairs:
Solution 4
This solution is by Martin Rebas.
See the three circles as three spheres of equal size, but projected in 3d, so the biggest circle represents the sphere that is closest to the viewer, and so on. Then, the three pairs of tangents define three infinitely long cylinders, which obviously (being in the same plane) have to recede towards the same horizon line.
Solution 5
This vector solution comes from M. Erickson's Beautiful Mathematics:
Suppose the three circles are C1, C2, C3, with centers c1, c2, c3 and radii r1, r2, r3. (Note that the symbols in bold designate vectors.) Let pij,
pij = ci + [ri/(ri - rj)](ci - cj) = (ricj - rjci) / (ri - rj).
It follows that
r1(r2 - r3)p23 + r2(r3 - r1)p13 + r3(r1 - r2)p12 = 0,
and therefore p13, p12, p23 are collinear because the scalar coefficients sum up to 0.
Remark
Another proof (and a generalization) can be obtained with the help of the Menelaus' theorem. Further, any two circles are similar and could be obtained from each other by a similarity transformation (homothety) centered at a point of intersection of their common tangents. It's known that, in general, if one figure is homothetic to another, and the second to the third, and the product of the coefficients of homothety is positive, but distinct from 1, then the product of two homotheties is a homothety and the three homothety centers are collinear, see Yaglom, p. 27. Additional proofs follow as easily from Desargues' theorem.
As applied to circles, two circles may have 0, 1, or 2 pairs of common tangents. In the latter case, one pair is called internal, the other external. (In the diagram, three pairs of external tangents are shown, but any two circles have also internal tangents that meet in-between the circles.) If the number of external tangencies is odd (1 or 3) then the centers of homotheties are collinear. To understand this rule, think of circles and straight lines as oriented - an arrow is attached to either pointing in the positive direction of traversal. The opposite direction is considered negative.
An oriented circle may touch another oriented circle or an oriented line in one of two ways. Either the traversal directions of the two figures at the point of tangency are compatible or "against the grain" - depending on whether the figures have the opposite orientations or the same.
The above rule becomes clear if applied to oriented lines. Of the three circles, either all have the same orientation or the orientation of one differs from that of the other two. In both cases, the theorem applies to oriented tangent lines whose orientation is compatible with the orientation of the circles.
The line through the centers of homothety of three (oriented) circles is known as their axis of homothety or axis of similitude.
References
- M. Erickson, Beautiful Mathematics, MAA, 2011
- M. Gardner, The Colossal Book of Short Puzzles and Problems, W. W. Norton & Co., 2006
- D. Wells, You are a Mathematician, John Wiley & Sons, 1995
- I. M. Yaglom, Geometric Transformations II, MAA, 1968
Monge's Theorem
- Three Circles and Common Tangents
- Monge from Desargue
- Monge via Desargue
2D Problems That Benefit from a 3D Outlook
- Four Travellers, Solution
- Desargues' Theorem
- Soddy Circles and Eppstein's Points
- Symmetries in a Triangle
- Three Circles and Common Chords
- Three Circles and Common Tangents
- Three Equal Circles
- Menelaus from 3D
- Stereographic Projection and Inversion
- Stereographic Projection and Radical Axes
- Sum of Squares in Equilateral Triangle
|Contact| |Front page| |Contents| |Geometry| |Up|
Copyright © 1996-2018 Alexander Bogomolny
73289155
