McDougall's Generalization of Ptolemy's Theorem
Recall that with the help of Brahmagupta-Mahavira Identities, we obtained the second Ptolemy theorem:
$\displaystyle\frac{m}{n} = \frac{a\cdot b + c\cdot d}{a\cdot d + b\cdot c},$
where $a,b,c,d,m,n$ stand for the side and diagonal lengths as shown below:
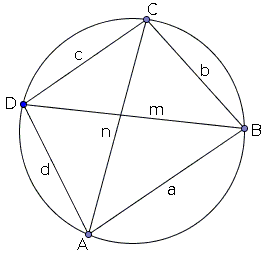
The ratio can be rewritten as
(1)
$\displaystyle\frac{1}{nbc} +\frac{1}{nad} = \frac{1}{mcd}+\frac{1}{mbc}$
In The American Mathematical Monthly (March 2004), Chamberland and Zeilberger report a 2012 result by Jane McDougall that generalizes the latter identity and supply a short proof. For the sake of generalization, we shall consider and even number of points $\{P_k\},\space k=1,2,...,2n$ on a circle. Let $d_{k,m}$ be the distance between $P_k$ and $P_m.$ In addition, define
$\displaystyle R_{k}=\prod_{m\ne k}^{2n}d_{k,m}.$
In these notations, (1) appears as
(1')
$\displaystyle\frac{1}{R_1} +\frac{1}{R_3} = \frac{1}{R_2}+\frac{1}{R_4},$
or
(1'')
$\displaystyle\sum_{k=1}^{4}(-1)^{k}\frac{1}{R_k}=0.$
Both forms beg for a generalization:
Let $n$ be a positive integer and $P_k,$, for $1\le k\le 2n,$ be cyclically ordered points on a circle. With, $R_{k}$ defined above,
$\displaystyle\sum_{k=1}^{2n}(-1)^{k}\frac{1}{R_k}=0,$
or
$\displaystyle\sum_{k=1}^{n}\frac{1}{R_{2k}}=\sum_{k=1}^{n}\frac{1}{R_{2k-1}}.$
A key to the proof is the Lagrange Interpolation formula:
If $P(z)$ is a polynomial whose degree does not exceed $N-1,$ then
$\displaystyle P(z)=\sum_{k=1}^{N}\frac{(z-z_{1})\cdots (z-z_{k-1})(z-z_{k+1})\cdots (z-z_{N})}{(z_{k}-z_{1})\cdots (z_{k}-z_{k-1})(z_{k}-z_{k+1})\cdots (z_{k}-z_{N})}P(z_k).$
for $N$ distinct points $z_k.$
Without loss of generality, assume that the circle is centered at the origin and has radius one. Denote the points as $P_{k}=\cos 2t_{k}+i\sin 2t_{k},$ where $0\le t_{1}\lt t_{2}\lt\ldots\lt\pi.$ Basic trigonometry can be used to show that $d_{k,m}=2\sin(t_{m}-t_{k}).$ Setting $u_{k}=e^{it_{k}},$
$\displaystyle d_{k,m}=-i\frac{u_{k}^{2}-u_{m}^{2}}{u_{k}u_{m}},$
for $k\lt m.$ This leads to
$\displaystyle i^{2n-1}(-1)^{k-1}\frac{1}{R_{k}}=\bigg(\prod_{m=1}^{2n}u_{m}\bigg)\frac{u_{k}^{2n-2}}{\displaystyle\prod_{m\ne k}(u_{k}^{2}-u_{m}^{2})}.$
What needs to be proved reduces now to
(2)
$\displaystyle i^{2n-1}\sum_{k=1}^{2n}(-1)^{k-1}\frac{1}{R_{k}}=\bigg(\prod_{m=1}^{2n}u_{m}\bigg)\sum_{k=1}^{2n}\frac{u_{k}^{2n-2}}{\displaystyle\prod_{m\ne k}(u_{k}^{2}-u_{m}^{2})}.$
Proving the theorem is therefore equivalent to proving that the sum on the right side of (2) equals zero.
We now apply the Lagrange interpolation to $P(z)=z^{r}.$ In the formula, if $r\lt N-1$ the coefficient by $z^{N-1}$ is naturally zero:
$\displaystyle 0=\sum_{k=1}^{N}\frac{z_{k}^{r}}{(z_{k}-z_{1})\cdots (z_{k}-z_{k-1})(z_{k}-z_{k+1})\cdots (z_{k}-z_{N})}.$
Taking $N=2N,$ $r=n-1,$ and $z_{k}=u_{k}^{2},$ $k=1,2,\ldots ,2n$ converts the latter equation into
$\displaystyle 0=\sum_{k=1}^{2n}\frac{u_{k}^{2n-2}}{\displaystyle\prod_{m\ne k}(u_{k}^{2}-u_{m}^{2})},$
which proves the theorem.
At the end of their article Chamberland and Zeilberger remark that by letting the radius of the circle grow to infinity, one obtains the validity of the result for a straight line, with an added benefit that it holds even for an odd number of points. Grégoire Nicollier noticed that no limiting procedure is required. The Lagrange interpolation formula applies - exactly as above - to any set of points, in particular all lying in the same line. Looking at the coefficient of $x^n,$ which is of course $0,$ proves the statement for any number of points $n\gt 1.$
References
- Marc Chamberland and Doron Zeilberger, A Short Proof of McDougall's Circle Theorem, Am Math Monthly, March 2014, 263-265
Ptolemy's Theorem
- Ptolemy's Theorem
- Sine, Cosine, and Ptolemy's Theorem
- Useful Identities Among Complex Numbers
- Ptolemy on Hinges
- Thébault's Problem III
- Van Schooten's and Pompeiu's Theorems
- Ptolemy by Inversion
- Brahmagupta-Mahavira Identities
- Casey's Theorem
- Three Points Casey's Theorem
- Ptolemy via Cross-Ratio
- Ptolemy Theorem - Proof Without Word
- Carnot's Theorem from Ptolemy's Theorem
|Contact| |Front page| |Contents| |Geometry| |Up|
Copyright © 1996-2018 Alexander Bogomolny
73296589
