Problem 1 From the 2016 Pan-African Math Olympiad

Problem

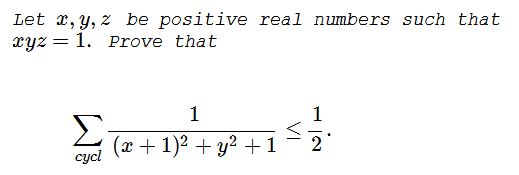
Solution

$\begin{align} (x+1)^2+y^2+1 &= (x^2+y^2) +2x+2\\ &\ge 2(xy+x+1). \end{align}$

The other two summands are modified appropriately. Introduce $a,b,c\,$ via $\displaystyle x=\frac{b}{a},\,$ $\displaystyle y=\frac{c}{b},\,$ $\displaystyle z=\frac{a}{c}.\,$ Note that $\displaystyle xy=\frac{c}{a}.\,$ It the follows that

$\begin{align} \sum_{cycl}\frac{1}{(x+1)^2+y^2+1} &\le \sum_{cycl}\frac{1}{2(xy+x+1)}\\ &=\frac{1}{2}\sum_{cycl}\frac{1}{\displaystyle \frac{c}{a}+\frac{b}{a}+1}\\ &=\frac{1}{2}\sum_{cycl}\frac{a}{a+b+c}\\ &=\frac{1}{2}\frac{a+b+c}{a+b+c}=\frac{1}{2}. \end{align}$

Acknowledgment

Dan Sitaru has kindly posted at the CutTheKnotMath facebook page the above problem from the $24^{th}\,$ Pan-African Mathematical Olympiad, along with his solution. Dan had also remarked that the problem was created in 2006 by Cristinel Mortici from Romania

Inequalities with the Product of Variables as a Constraint

An Application of Schur's Inequality II $\left(\sum (x^4+y^3+z) \ge \sum \left(\frac{x^2+y^2}{z}\right)+3\right)$
Problem 1 From the 2016 Pan-African Math Olympiad $\left(\displaystyle \sum_{cycl}\frac{1}{(x+1)^2+y^2+1}\le\frac{1}{2}\right)$
A Cyclic But Not Symmetric Inequality in Four Variables $\left(\displaystyle 5(a+b+c+d)+\frac{26}{abc+bcd+cda+dab}\ge 26.5\right)$
Problem 2, the 36th IMO (1995) $\left(\displaystyle \frac{1}{a^3(b+c)}+\frac{1}{b^3(c+a)}+\frac{1}{c^3(a+b)}\ge\frac{3}{2}\right)$
An Inequality with Two Cyclic Sums $\left(\displaystyle \sum_{cycl}(a+\sqrt[3]{a}+\sqrt[3]{a^2})\ge 9\sum_{cycl}\frac{1}{1+\sqrt[3]{b^2}+\sqrt[3]{c}}\right)$
The Roads We Take $(x(x-3(y+z))^2+(3x-(y+z))^2(y+z)\ge 27)$
Long Huynh Huu's Inequality and Solution $\left(\displaystyle \sum_{i=1}^n\arctan x_i\le (n-1)\frac{\pi}{2}\right)$
An Inequality with Integrals $\left(\Omega=\int_0^u\frac{u^4+7x^3-25x^2+37x+4}{x^4-3x^3+5x^2-3x+4}dx,\;\Omega(a)+\Omega(b)+\Omega(C)\ge3+5\ln\prod_{cycl}(1+a^2)\right)$
An Inequality with Cycling Sums $\left(\displaystyle\sum_{cycl}(x^4+y^3+z)\ge \sum_{cycl}\frac{x^2+y^2}{z}+3\right)$
Two Products: Constraint and Inequality $\left(\displaystyle\prod_{k=1}^n(1+a_k)\ge 2^n\right)$
Leo Giugiuc's Cyclic Inequality with a Constraint $\left(\displaystyle a^3+b^3+c^3+\frac{16}{(a+b)(b+c)(c+a)}\ge 5\right)$

72111977