A Generalized Cavalieri-Zu Principle
Sidney Kung

Area, Sector Area, and Segment Area of an Ellipse

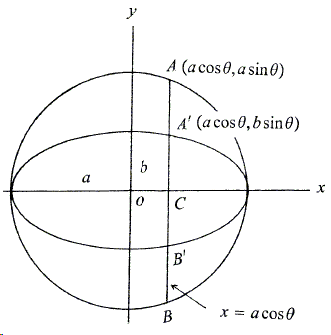
Area of ellipse by the Cavalier-Zu generalized principle

Let \(A_{1}\) and \(A_{2}\) be the areas of a circle and an ellipse, respectively. Line \(x=\mbox{cos}\theta\) intersects the circle at \(A\), \(B\) and the ellipse at \(A',\) and \(B'\), respectively. It's easy to see that \(\frac{A'C}{AC}=\frac{A'B'}{AB}=\frac{b}{a}\). Thus, from (*), the area of the ellipse is

(1)

\(A_{2}=\frac{b}{a}A_{1}=\frac{b}{a}\pi a^{2}=\pi ab\).

An elliptic sector is a region bounded by an arc and line segments connecting the center of the ellipse (the origin in our diagrams) and the endpoints of the arc.

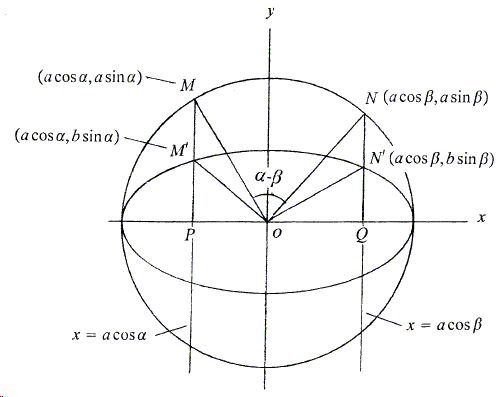
Area of an elliptic sector by the Cavalier-Zu generalized principle

Let lines \(x=a\space\mbox{cos}\alpha\) and \(x=a\space\mbox{cos}\beta\) be perpendicular to the \(x\)-axis, and let \([F]\) indicate the area of figure \(F\).

The coordinates of the points \(M\), \(M'\), \(N\), \(N'\) are \((a\space\mbox{cos}\alpha , a\space\mbox{sin}\alpha)\), \((a\space\mbox{cos}\alpha , b\space\mbox{sin}\alpha)\), \((a\space\mbox{cos}\beta , a\space\mbox{sin}\beta)\), and \((a\space\mbox{cos}\beta , b\space\mbox{sin}\beta)\), respectively. Since \(\frac{N'Q}{NQ}=\frac{M'P}{MP}=\frac{b}{a}\), we have \(\frac{[PM'N'Q]}{[PMNQ]}=\frac{[N'OQ]}{[NOQ]}=\frac{[M'OP\space]}{[MOP\space]}=\frac{b}{a}\). Thus,

\(\frac{[PM'N'Q]-[N'OQ]-[M'OP\space]}{[PMNQ]-[NOQ]-[MOP\space]}=\frac{b}{a}\), or \(\frac{[M'ON']}{[MON\space]}=\frac{b}{a}\).

Therefore, the area of the elliptic sector \(M'ON'\) is

(2)

\([M'ON']=\frac{b}{a}\left(\frac{\alpha -\beta}{2\pi}\right)\pi a^{2}=\frac{1}{2}(\alpha -\beta)ab\). \((\alpha \gt \beta)\).

An elliptic segment is a region bounded by an arc and the chord connecting the arc's endpoints.

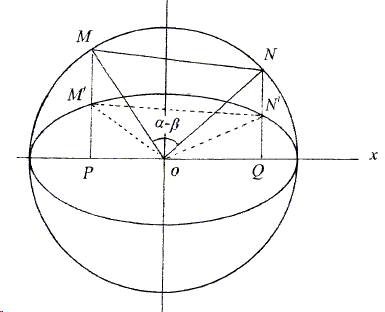
Area of an elliptic segment by the Cavalier-Zu generalized principle

Note that the area of the elliptic segment (in then diagram) is equal to the area of sector \(M'ON'\) minus the area of \(\triangle M'ON'\). Hence, the elliptic segment area is

(3)

\(\frac{ab}{2}(\alpha -\beta)-\frac{b}{a}\left(\frac{a^{2}}{2}\mbox{sin}(\alpha -\beta)\right) =\frac{ab}{2}\left((\alpha-\beta)-\mbox{sin}(\alpha-\beta)\right)\).

Cavalieri Principle
A Generalized Cavalieri-Zu Principle
Area, Sector Area, and Segment Area of an Ellipse
Volume of a Sphere and Volume of an Ellipsoid
Volume of an Elliptic Paraboloid
Volume of a Hyperboloid of One Sheet
Volume of a Hyperboloid of Two Sheet
Volume of a Paraboloid of Revolution

71867924