Area of Rectangle
I plan to argue here that the area of a rectangle needs to equal the product of its side lengths. This can be taken as an axiom. However the fact is sufficiently profound to merit an extended treatment.
We already accepted two axioms
Axiom 1
Congruent shapes have equal areas.
Axiom 2
If a polygon is split into a finite number of polygons then its area is the sum of the areas of the pieces it is split into.
We also agreed that $S(U)$ of the unit square $U$ should be $1.$
Theorem
For rectangle $ABCD$, $S(ABCD)=AB\times BC.$
Observe that this agrees with the stipulation that $S(U)=1$ for the unit square $U,$ because both sides of the latter each measures $1$ unit.
Proof
Pick two rectangles $ABCD$ and $ABC'D'$ that share a side:
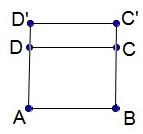
I claim that their areas are in the ratio of their sides:
$\displaystyle\frac{S(ABCD)}{S(ABC'D')}=\frac{BC}{BC'}.$
Proving that takes three steps (see the derivation of the equation of the linear function, it is very similar.) First, if $BC' = nBC$ then clearly $ABC'D'$ consists of $n$ identical copies of $ABCD$ such that, by Axiom 2,
$\displaystyle\frac{S(ABCD)}{S(ABC'D')}=\frac{1}{n}=\frac{BC}{BC'}.$
On the second step, be turn what we just arrived at on its head: Assume that $mBC' = BC.$ Then as before but switching the roles of the two rectangles,
$\displaystyle\frac{S(ABC'D')}{S(ABCD)}=\frac{1}{m}=\frac{B'C'}{BC}.$
Combining the two discoveries, if $\displaystyle BC' = \frac{n}{m}BC$ then also
$\displaystyle\frac{S(ABC'D')}{S(ABCD)}=\frac{n}{m}=\frac{B'C'}{BC}.$
This settles the question where the side lengths are in a rational ratio. For the third step we have to assume that the area function is continuous. If that is really so, then whatever holds for the rational factors also hold for the real ones.
Thus we see that the area $S(ABCD)$ is bound to be proportional to $BC.$ Now, observe that instead of fixing $AB$ we could have fixed, say $BC.$ The result would be that the area $S(ABCD)$ is bound to be proportional to $AB.$ It follows that the area $S(ABCD)$ ought to be proportional to the product $AB\cdot BC.$ However, to be consistent with the requirement that for the unit square $U,$ $S(U)=1,$ it is necessary that $S(ABCD)$ be equal not just proportional $AB\cdot BC.$
References
- J. Hadamard, Leçons de géométrie élémentaire, tome I, 13e édition, 1947, Editions Jacques Gabay, 1988, ISBN 2-87647-038-1.
- D. Hilbert, Foundations of Geometry, Open Court, 1999
- Kiselev's Geometry. Book I. PLANIMETRY, adapted from Russian by Alexander Givental, Sumizdat, 2006.
- What Is Area
- Elementary Introduction into the Concept of Area
- Area of Rectangle
- Area of Parallelogram
- Area of Triangle
- Area and Perimeter of Circle
- Equidecomposition of a Triangle and a Rectangle: first variant
- Pick's Theorem
- Area of a Circle by Rabbi Abraham bar Hiyya Hanasi
- Area of a Circle by Leonardo da Vinci
- Volume and Area of Torricelli's Trumpet
|Contact| |Front page| |Contents| |Algebra| |Up|
Copyright © 1996-2018 Alexander Bogomolny
71550390
