Concurrency in Arbelos

Concurrency in Arbelos: What is this about?
A Mathematical Droodle

If you are reading this, your browser is not set to run Java applets. Try IE11 or Safari and declare the site https://www.cut-the-knot.org as trusted in the Java setup.

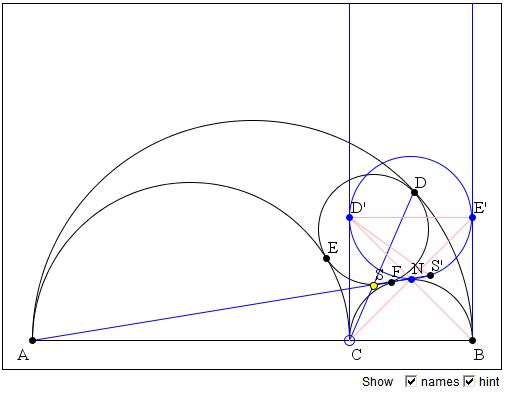
Arbelos is a geometric shape bounded by three semicircles. In the applet these are AC, CB and AB. Assume M is the midpoint of the semicircle on the diameter AC, N that of the semicircle CB. A circle is inscribed in the arbelos tangent to the semicircles in points E, F, and D. Then the lines AN, BM and CD are concurrent. The point of concurrency lies on the incircle.

Concurrency in Arbelos, proof

The statement above and the proof below are from [Woo].

Proof

Perform an inversion with center A and power AC·AB. This will invert B into C and vice versa. Hence the circle on the diameter CB is stationary. The big semicircle inverts into the line perpendicular to AB at C, and the left semicircle into a line perpendicular to AB at B. The incircle of the arbelos that is tangent to all three semicircles inverts into the circle tangent to the two vertical lines and the right semicircles. The image of F is N, the topmost point of the semicircle on BC. The images D' and E' of the points D and E lie on the vertical lines and, therefore form a diameter D'E' of the inversive image of the incircle. By Archimedes' Proposition 1, or due to the local symmetry of the configuration, points B, N, and D' are collinear.

Let S be the point of intersection of AN with the incircle other than F and S' its inversive image.

∠AS'D' = ∠NS'D' = ∠NE'D' = 45°.

Also, ∠ABD' = 45°. Therefore, the points A, B, S', D' are concyclic. The inversive image of their circle is the straight line that passes through C, S and D. We see that S lies on CD, with the conclusion that CD and AN meet (in S) on the incircle. Considering inversion centered at B, we get a similar conclusion that CD and BM meet in S on the incircle.

Remark

Note that the statement just proved provides a construction of the incircle: first, find the midpoints points M and N. Then, find S as the intersection of AN and BM. Finally, D is the intersection of CS with the big semicircle.

References

P. Y. Woo, Simple Constructions of the Incircle of an Arbelos, Forum Geometricorum, V. 1 (2001), 133-136

72109951