An Inequality with Constraint in Four Variables

Problem

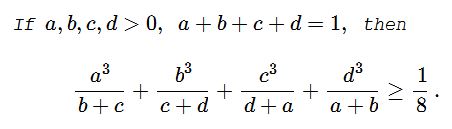
Inequality withConstraint in Four Variables

Solution 1

With Hölder's inequality,

$\displaystyle \left(\sum_{cycl}a\right)^3\le\left(\sum_{cycl}\frac{a^3}{b+c}\right)\left(\sum_{cycl}(b+c)\right)\sum_{cycl}1.$

It follows that

$\displaystyle 1\le\left(\sum_{cycl}\frac{a^3}{b+c}\right)\cdot 2\cdot 4,$

implying the required inequality.

Solution 2

By Bergstrom's inequality,

$\displaystyle\frac{a^3}{b+c}+\frac{b^3}{c+d}+\frac{c^3}{d+a}+\frac{d^3}{a+b}\ge\frac{(a+b+c+d)^3}{\displaystyle\left(\sum_{cycl}\sqrt{b+c}\right)^2}.$

Hence, suffice it to prove that

$\displaystyle\frac{(a+b+c+d)^3}{\displaystyle\left(\sum_{cycl}\sqrt{b+c}\right)^2}\ge\frac{1}{8}.$

Given the constraint, this is equivalent to

(1)

$\displaystyle\left(\sum_{cycl}\sqrt{b+c}\right)^2\le 8.$

Squaring on the left gives

(2)

$\displaystyle\left(\sum_{cycl}\sqrt{b+c}\right)^2 = 2\sum_{cycl}a+2\Pi,$

where $\Pi\,$ is the sum of six pairwise products of the terms $\sqrt{b+c},\,$ $\sqrt{c+d},\,$ $\sqrt{d+a},\,$ $\sqrt{a+b}.\,$ We replace each using the AM-GM inequality, e.g.,

$\displaystyle\sqrt{(b+c)(c+d)}\le\frac{(b+c)+(c+d)}{2},$

each sum on the right containing in the numerator two pairs of the terms $b+c,\,$ $c+d,\,$ $d+a,\,$ $a+b.\,$ Thus there are twelve such pairs in the total that, by symmetry includes equal amounts of each variable. There being four of them, each appears six times in the total. We deduce that $\Pi\le\displaystyle\frac{6(a+b+c+d)}{2}=3(a+b+c+d).$

Returning to (2), we see that (1) will be proved, provided

$\displaystyle 2\sum_{cycl}a+2\Pi \le 2\sum_{cycl}a+6\sum_{cycl}a\le 8.$

But this is true, due to the given constraint.

Illustration

Inequality withConstraint in Four Variables, illustration, Part 1

Acknowledgment

Dan Sitaru has kindly posted the above problem, with a solution, at the CutTheKnotMath facebook page. The problem is by George Apostolopoulos. Solution 1 is by Kevin Soto Palacios. The illustration is by Nassim Nicolas Taleb.

Inequalities with the Sum of Variables as a Constraint

An Inequality for Grade 8 $\left(\displaystyle\frac{1-x_1}{1+x_1}\cdot\frac{1-x_2}{1+x_2}\cdot\ldots\cdot\frac{1-x_n}{1+x_n}\ge\frac{1}{3}\right)$
An Inequality with Constraint $((x+1)(y+1)(z+1)\ge 4xyz)$
An Inequality with Constraints II $\left(\displaystyle abc+\frac{2}{ab+bc+ca}=p+\frac{2}{q}\ge q-2+\frac{2}{q}\right)$
An Inequality with Constraint V $\left(\displaystyle\prod_{k=1}^{n}x_k^{1/x_k}\le \frac{1}{n^{n^2}}\right)$
An Inequality with Constraint VI $\left(\displaystyle\prod_{k=1}^{n}\frac{1+x_k}{x_k}\ge \prod_{k=1}^{n}\frac{n-x_k}{1-x_k}\right)$
An Inequality with Constraint XI $(\sqrt{5a+4}+\sqrt{5b+4}+\sqrt{5c+4} \ge 7)$
Monthly Problem 11199 $\left(\displaystyle\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\ge\frac{25}{1+48abc}\right)$
Problem 11804 from the AMM $(10|x^3 + y^3 + z^3 - 1| \le 9|x^5 + y^5 + z^5 - 1|)$
Sladjan Stankovik's Inequality With Constraint $\left(abc+bcd+cda+dab-abcd\le\displaystyle \frac{27}{16}\right)$
Sladjan Stankovik's Inequality With Constraint II $(a^4+b^4+c^4+d^2+4abcd\ge 8)$
An Inequality with Constraint V $\left(\displaystyle\prod_{k=1}^{n}x_k^{1/x_k}\le \frac{1}{n^{n^2}}\right)$
An Inequality with Constraint VI $\left(\displaystyle\prod_{k=1}^{n}\frac{1+x_k}{x_k}\ge \prod_{k=1}^{n}\frac{n-x_k}{1-x_k}\right)$
An Inequality with Constraint XII $(abcd\ge ab+bc+cd+da+ac+bd-5)$
An Inequality with Constraint XIII $((3a-bc)(3b-ca)(3c-ab)\le 8a^2b^2c^2)$
Inequalities with Constraint XV and XVI $\left(\displaystyle\frac{a^2}{\sqrt{b^2+4}}+\frac{b^2}{\sqrt{c^2+4}}+\frac{c^2}{\sqrt{a^2+4}}\gt\frac{3}{5}\right)$ and $\left(\displaystyle\frac{a^2}{\sqrt{b^4+4}}+\frac{b^2}{\sqrt{c^4+4}}+\frac{c^2}{\sqrt{a^4+4}}\gt\frac{3}{5}\right)$
An Inequality with Constraint XVII $(a^3+b^3+c^3\ge 0)$
An Inequality with Constraint in Four Variables $\left(\displaystyle\frac{a^3}{b+c}+\frac{b^3}{c+d}+\frac{c^3}{d+a}+\frac{d^3}{a+b}\ge\frac{1}{8}\right)$
An Inequality with Constraint in Four Variables II $(a^3+b^3+c^3+d^3 + 6abcd \ge 10)$
An Inequality with Constraint in Four Variables III $\left(\displaystyle\small{abcd+\frac{15}{2(ab+ac+ad+bc+bd+cd)}\ge\frac{9}{a^2+b^2+c^2+d^2}}\right)$
An Inequality with Constraint in Four Variables IV $\left(\displaystyle 27+3(abc+bcd+cda+dab)\ge\sum_{cycl}a^3+54\sqrt{abcd}\right)$
Inequality with Constraint from Dan Sitaru's Math Phenomenon $\left(\displaystyle b+2a+20\ge 2\sum_{cycl}\frac{a^2+ab+b^2}{a+b}\ge b+2c+20\right)$
An Inequality with a Parameter and a Constraint $\left(\displaystyle a^4+b^4+c^4+\lambda abc\le\frac{\lambda +1}{27}\right)$
Cyclic Inequality with Square Roots And Absolute Values $\left(\displaystyle \prod_{cycl}\left(\sqrt{a-a^2}+\frac{1}{2\sqrt{2}}|3a-1|\right)\ge\frac{1}{6\sqrt{6}}\prod_{cycl}\left(\sqrt{a}+\frac{1}{\sqrt{3}}\right)\right)$
From Six Variables to Four - It's All the Same $\left(\displaystyle \frac{5}{2}\le a^2+b^2+c^2+d^2\le 5\right)$
Michael Rozenberg's Inequality in Three Variables with Constraints $\left(\displaystyle 4\sum_{cycl}ab(a^2+b^2)\ge\sum_{cycl}a^4+5\sum_{cycl}a^2b^2+2abc\sum_{cycl}a\right)$
Michael Rozenberg's Inequality in Two Variables $\left(\displaystyle \sqrt{x^2+3}+\sqrt{y^2+3}+\sqrt{xy+3}\ge 6\right)$
Dan Sitaru's Cyclic Inequality in Three Variables II $\left(\displaystyle \sum_{cycl}\sqrt{1+\frac{1}{a^2}+\frac{1}{(a+1)^2}}\geq \frac{9}{12-2(ab+bc+ca)}+3\right)$
Dan Sitaru's Cyclic Inequality in Three Variables IV $\left(\displaystyle \sum_{cycl}\frac{(x+y)z}{\sqrt{4x^2+xy+4y^2}}\le 2\right)$
Dan Sitaru's Cyclic Inequality in Three Variables VI $\left(\displaystyle \sum_{cycl}\left[\sqrt{a(a+2b)}+\sqrt{b(b+2a)}\,\right]\le 6\sqrt{3}\right)$
An Inequality with Arbitrary Roots $\left(\displaystyle \sum_{cycl}\left(\sqrt[n]{a+\sqrt[n]{a}}+\sqrt[n]{a-\sqrt[n]{a}}\right)\lt 18\right)$
Inequality 101 from the Cyclic Inequalities Marathon $\left(\displaystyle \sum_{cycl}\frac{c^5}{(a+1)(b+1)}\ge\frac{1}{144}\right)$
Sladjan Stankovik's Inequality With Constraint II $(a^4+b^4+c^4+d^2+4abcd\ge 8)$
An Inequality with Constraint in Four Variables $\left(\displaystyle\frac{a^3}{b+c}+\frac{b^3}{c+d}+\frac{c^3}{d+a}+\frac{d^3}{a+b}\ge\frac{1}{8}\right)$
An Inequality with Constraint in Four Variables IV $\left(\displaystyle 27+3(abc+bcd+cda+dab)\ge\sum_{cycl}a^3+54\sqrt{abcd}\right)$
Cyclic Inequality with Square Roots And Absolute Values $\left(\displaystyle \prod_{cycl}\left(\sqrt{a-a^2}+\frac{1}{2\sqrt{2}}|3a-1|\right)\ge\frac{1}{6\sqrt{6}}\prod_{cycl}\left(\sqrt{a}+\frac{1}{\sqrt{3}}\right)\right)$
From Six Variables to Four - It's All the Same $\left(\displaystyle \frac{5}{2}\le a^2+b^2+c^2+d^2\le 5\right)$
Michael Rozenberg's Inequality in Two Variables $(\displaystyle \sqrt{x^2+3}+\sqrt{y^2+3}+\sqrt{xy+3}\ge 6)$
Dan Sitaru's Cyclic Inequality in Three Variables II $\left(\displaystyle \sum_{cycl}\sqrt{1+\frac{1}{a^2}+\frac{1}{(a+1)^2}}\geq \frac{9}{12-2(ab+bc+ca)}+3\right)$
Dorin Marghidanu's Two-Sided Inequality $\left(\displaystyle \small{64(a+bc)(b+ca)(c+ab)}\le \small{8(1-a^2)(1-b^2)(1-c^2)}\le \small{(1+a)^2(1+b)^2(1+c^2)}\right)$
Problem 6 from Dan Sitaru's Algebraic Phenomenon $(x\sqrt{y+1}+y\sqrt{z+1}+z\sqrt{x+1}\le 2\sqrt{3})$
A Warmup Inequality from Vasile Cirtoaje $\left(a^4b^4+b^4c^4+c^4a^4\le 3\right)$
An Extension of the AM-GM Inequality $\left(x_{1}x_{2} + x_{2}x_{3} + x_{3}x_{4} + \ldots + x_{99}x_{100} \le \frac{1}{4}\right)$
- An Extension of the AM-GM Inequality: A second look $\left(x_{1}x_{2} + x_{2}x_{3} + x_{3}x_{4} + \ldots + x_{n-1}x_{n} \le \frac{a^2}{4}\right)$
Distance Inequality $\left(a^2+b^2+c^2\le\displaystyle\frac{9}{2}\right)$
Kunihiko Chikaya's Inequality with a Constraint $\left(4a^3+9b^3+36c^3\ge 1\right)$ An Inequality with Five Variables, Only Three Cyclic $\left(\displaystyle \left(a+\frac{b}{c}\right)^4+ \left(a+\frac{b}{d}\right)^4+ \left(a+\frac{b}{e}\right)^4\ge 3(a+3b)^4\right)$
Second Pair of Twin Inequalities: Twin 1 $\left(\displaystyle \prod_{i=1}^n\left(\frac{1}{a_i^2}-1\right)\ge (n^2-1)^n\right)$
Second Pair of Twin Inequalities: Twin 2 $\left(\displaystyle \prod_{i=1}^n\left(\frac{1}{a_i}+1\right)\ge (n+1)^{n}\right)$
Cyclic Inequality In Three Variables from the 2018 Romanian Olympiad, Grade 9 $\left(\displaystyle \frac{a-1}{b+1}+\frac{b-1}{c+1}+\frac{c-1}{a+1}\ge 0\right)$
Dan Sitaru's Cyclic Inequality in Three Variables IX $\left(\displaystyle \sum_{cycl}\sqrt{(x+y+1)(y+z+1)}\le 6+\sum_{cycl}\frac{x^3+y^3}{x^2+y^2}\right)$
Vasile Cirtoaje's Cyclic Inequality with Three Variables $\left(\displaystyle \sqrt{\frac{a}{b+c}}+\sqrt{\frac{b}{c+a}}+\sqrt{\frac{c}{a+b}}\ge 2\right)$ Leo Giugiuc and Vasile Cirtoaje's Cyclic Inequality $\left(\displaystyle \sqrt{\frac{a}{1-a}}+\sqrt{\frac{b}{1-b}}+\sqrt{\frac{c}{1-c}}+\sqrt{\frac{d}{1-d}}\ge 2\right)$

71946805