A Carpet With a Hole
One of the most likable stories I ever read to my little boy was Something from nothing by D. Blitz. In short it went like this:
When Yaakov was born, Grandpa Meir brought him a beautiful blanket. Yaakov liked the blanket very much. In time, the blanket grew worn out and needed fixing. Grandpa Meir had managed to make a pillowcase out of whatever remained of the blanket. Yaakov liked the pillowcase very much. When, after a few years, the pillow, too, became unusable, Grandpa Meir made out of the remainder a beautiful button. Yaakov warn proudly the button on his jacket until it went undone and got lost. This time, since Grandpa Meir could not create something from nothing, Yaakov sat down and wrote a story of the blanket's metamorphosis - apparently out of nothing.
The story came to mind while I was working on the problem presented by the applet below.
A rectangular carpet with a hole is to be mended by slicing it into two pieces which then combine into a square carpet.
The problem is pretty old and, in a Description of a similar puzzle, [Frederickson, p. 66] mentions both S. Loyd and H. E. Dudeney.
(In the applet place the cursor inside the grid and try to drag it - see what happens.)
| Buy this applet What if applet does not run? |
References
- S. Blitz, My First Book of Jewish Stories, Mesorah Publications, Ltd., 1999
- G. N. Frederickson, Dissections: Plain & Fancy, Cambridge University Press, 1997
|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener| |Store|
Copyright © 1996-2015 Alexander BogomolnyA rectangular carpet with a hole is to be mended by slicing it into two pieces which then combine into a square carpet.
To see the solution just hold the mouse down in the applet area and drag.
| Buy this applet What if applet does not run? |
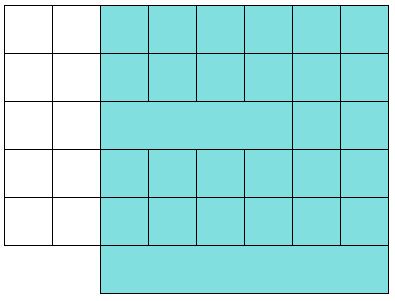
Several other dissection puzzles admit such a "stutter" solutions.
Equidecomposition by Dissiection
- Carpet With a Hole
- Equidecomposition of a Rectangle and a Square
- Equidecomposition of Two Parallelograms
- Equidecomposition of Two Rectangles
- Equidecomposition of a Triangle and a Rectangle
- Equidecomposition of a Triangle and a Rectangle II
- Two Symmetric Triangles Are Directly Equidecomposable
- Wallace-Bolyai-Gerwien Theorem
- Perigal's Proof of the Pythagorean Theorem
- A Proof Perigal and All Others After Him Missed
- Dissection of a Vase
- Curvy Dissection
|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener| |Store|
Copyright © 1996-2015 Alexander Bogomolny| 49552096 |
