Angle Trisection by Paul Vjecsner

I have recently received a letter from Paul Vjecsner, a New York thinker and an amateur mathematician. Among several claims made, there was also a neusis angle trisection illustrated by an applet below. Paul mentions an impossibility result I am not aware of:

Other than that, the construction is almost on a par with a better known ones by, say, Archimedes, Hippocrates or by paper folding.

To quote from a Paul Vjecsner's letter:

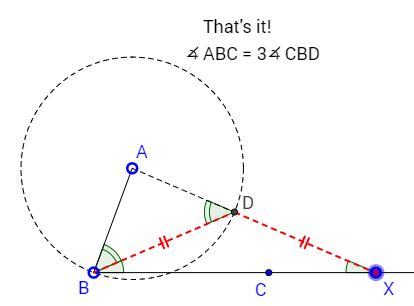
The proof a simple: assume A, C, D are collinear. Then triangles ABD and BCD are isosceles:

From the latter

On the other hand, ∠BDC is exterior to ΔABD such that:

If we compare the two identities we'll see that

or,

However it is worth pondering which geometric tools have been actually used in the construction. How exactly does one achieve the collinearity of A, C and D? The process appears to be a loop repeating the following steps:

1. Choose D on the circle centered at A and radius AB,
2. Find the intersection of the circle centered at D and radius BD with the other (not containing A) leg of the given angle,
3. Find the intersection of AD with that leg,
4. Repeat 1-3 until two intersections coincide.

Paul quotes from his correspondence with Prof. Robin Hartshorne of the University of California, Berkeley who wondered whether the tool in Paul's proof was equivalent to the marked ruler. Paul did not have an answer to that question and I do not either.

|Activities| |Contact| |Front page| |Contents| |Geometry| |Eye opener| |Store|

Copyright © 1996-2012 Alexander Bogomolny