Following is an excerpt from
The Mathematical Universe
by William Dunham

In the mid-nineteenth century, John Venn (1834-1923), a Fellow of Cambridge University, devised a scheme for visualizing logical relationships. Venn was a cleric in the Anglican Church, an authority on what was then called "moral science," and the compiler of a massive index of all Cambridge alumni. He was not terribly outstanding in mathematics. But, for better or worse, a single contribution has made him immortal.

That contribution is the Venn diagram. It is as much a fixture of' today's textbooks as the title page or table of contents. A Venn diagram is simply a field within which circular areas represent groups of items sharing common properties.

For instance, within the universe of all animals (the large rectangle in Figure V. 1), region C represents the camels, region B the birds, and region A the albatrosses. A glance at the diagram reveals that

Figure V.1

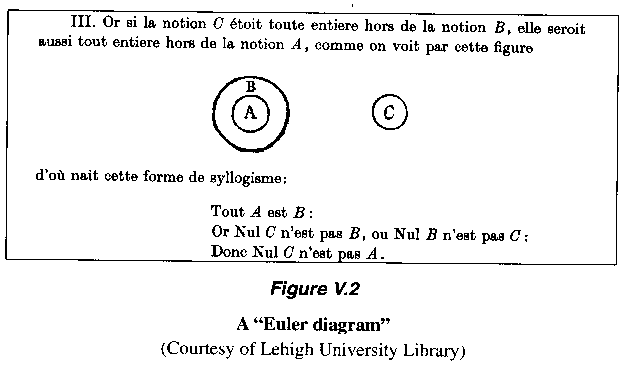
But there is more. Gottfried Wilhelm Leibniz, often regarded as the founder of symbolic logic, used little diagrams of this sort in the seventeenth century. And in Leonhard Euler's Opera Omnia we find the illustration depicted in Figure V.2. Look familiar? This is a "Venn diagram" a century before Venn. If justice is to be served, we should call this an "Euler diagram." Of course, such a name change would add little to Euler's stupendous fame but would obliterate John Venn's reputation altogether.

So, the Venn diagram is neither profound nor original. It is merely famous. Somehow within the realm of mathematics, John Venn's has become a household name. No one in the long history of mathematics ever became better known for less. There is really nothing more to be said.

As a matter of fact, Venn diagrams are used widely, even by professional mathematicians. See, for example, a recent review by Darren Glass of A. W. F. Edwards' Cogwheels of the Mind: The Story of Venn Diagrams. In October 2004, Frank Ruskey from University of Victoria kindly informed me of his correspondence with W. Dunham. Their exchange, which I use with permission, sheds additional light on the nature of controversy surrounding Venn diagrams. I plan to describe the latter at some length. Meanwhile, there is a Java applet (which requires Java 2, jre 1.3.1) that might help check one's understanding of Venn diagrams.

Frank Ruskey:

Dear William, I wonder whether you still agree with the opinions reproduced on the page http://www.cut-the-knot.org/LewisCarroll/dunham.shtml. It seems to me that the differences between Venn and Euler diagrams are well explained in Venn's book "J. Venn, Symbolic Logic" (which is still available through the AMS!). The diagrams referred to in the cut-the-knot reproduction are NOT Venn diagrams at all since they do not contain 2^n regions. Cordially, Frank

W. Dunham:

Frank: It may not surprise you that yours is not the first note I've received on this matter. Others have pointed out that I didn't give John Venn his due. Indeed, the diagram I included is not the full-blown 2^n Venn diagram, and perhaps I was overly harsh in my analysis of Mr. Venn's contribution. On the other hand, I continue to regard it as one of the least strenuous routes to mathematical immortality. Truth to tell, Chapter V was meant to be tongue-in-cheek. As The Mathematical Universe was coming together, I tried to add a less technical touch here and there. This accounts for Chapters M and W. Faced with Chapter V, I simply opted to have a little fun with a well-known concept. But, in the spirit of your message, I'll take a minute and tip my hat to J. Venn. Thanks for writing. W. Dunham


• Introduction
• Controversial Venn Diagrams
  • From K.Devlin, MATHEMATICS: The Science of Patterns
  • From W.Dunham, The Mathematical Universe
  • Venn Diagrams applet I
  • Venn Diagrams applet II
• Bilateral Diagrams
  • Interpretation of Bilateral diagrams
  • Bilateral diagrams applet
• Syllogisms
• Trilateral Diagrams
  • Interpretation of Trilateral diagrams
  • Trilateral diagrams applet
• Sample soriteses
• The Game