The Dual of Maxwell's Theorem

The following dual of Maxwell's theorem has been posted by Oai Thanh Dào at the CutTheKnotMath facebook page:

Let line $\ell$ be a transversal in $\Delta ABC$ crossing the sides $BC,$ $AC,$ $AB$ at $A_1,$ $B_1,$ $C_1,$ respectively. Let $A'B'C'$ be a triangle in the same plane, with $B'C',$ $A'C',$ $A'B'$ parallel to $AA_1,$ $BB_1,$ $CC_1,$ respectively.

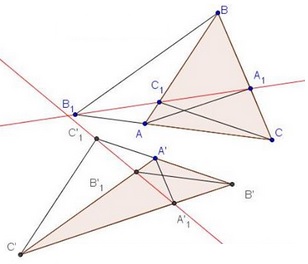
Dual to Maxwell's theorem

Then the three lines through $A',$ $B',$ $C'$ parallel to $BC,$ $AC,$ $AB$ meet $B'C',$ $A'C',$ $A'B',$ respectively, at three collinear points.

Proof

The proof is by Luis González.

Denote the three points in question $A'_1,$ $B'_1,$ $C'_1.$

$\frac{A'_1B'}{A'_1C'}=\frac{\sin \widehat{B'A'A'_1}}{\sin \widehat{C'A'A'_1}} \cdot \frac{A'B'}{C'A'}=\frac{\sin \widehat{BCC_1}}{\sin \widehat{CBB_1}} \cdot \frac{A'B'}{C'A'} \ \ (1).$

Similarly we have the expressions

$\frac{B'_1C'}{B'_1A'}=\frac{\sin \widehat{CAA_1}}{\sin \widehat{ACC_1}} \cdot \frac{B'C'}{A'B'} \ \ (2) \ , \ \frac{C'_1A'}{C'_1B'}=\frac{\sin \widehat{ABB_1}}{\sin \widehat{BAA_1}} \cdot \frac{C'A'}{B'C'} \ \ (3).$

Multiplying $(1),(2)$ and $(3)$ gives

$\frac{A'_1B'}{A'_1C'} \cdot \frac{B'_1C'}{B'_1A'} \cdot \frac{C'_1A'}{C'_1B'}=\frac{\sin \widehat{CAA_1}}{\sin \widehat{BAA_1}} \cdot \frac{\sin \widehat{BCC_1}}{\sin \widehat{ACC_1}} \cdot \frac{\sin \widehat{ABB_1}}{\sin \widehat{CBB_1}}= \frac{A_1C}{A_1B} \cdot \frac{B_1A}{B_1C} \cdot \frac{C_1B}{C_1A}.$

Hence, by Menelaus' theorem and its inverse, we conclude that since $A_1,B_1,C_1$ are collinear so are $A'_1,B'_1,C'_1.$

71753356