An Identity in Triangle with a 135 Degrees Angle
Problem
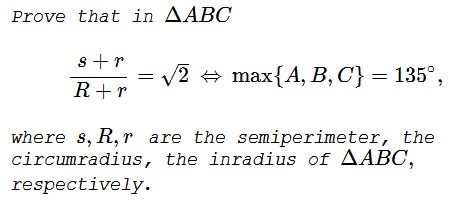
Solution
WLOG, assume $A=135^{\circ}\,$ so that, by the Law of Sines, $\displaystyle R=\frac{a}{a\sin A}=\frac{a\sqrt{2}}{2};\,$ $\displaystyle [\Delta ABC]=\frac{1}{2}\sin 135^{\circ}=\frac{\sqrt{2}bc}{4};\,$ and, by the Law of Cosines, $a^2=b^2+c^2+\sqrt{2}bc.\,$
Now we have a sequence of equivalent identities:
$\displaystyle \begin{align} \frac{s+r}{R+r} &\Leftrightarrow\,s=\sqrt{2}R+r(\sqrt{2}-1)r\\ &\Leftrightarrow\,s=a+r(\sqrt{2}-1)r\\ &\Leftrightarrow\,a+b+c=2a+2r(\sqrt{2}-1)\\ &\Leftrightarrow\,b+c-a=(\sqrt{2}-1)\frac{\sqrt{2}bc}{2s}\\ &\Leftrightarrow\,(a+b+c)(b+c-a)=(2-\sqrt{2})bc\\ &\Leftrightarrow\,(b+c)^2-a^2=(2-\sqrt{2})bc\\ &\Leftrightarrow\,(b+c)^2-b^2-c^2-\sqrt{2}bc=(2-\sqrt{2})bc\\ &\Leftrightarrow\,(2-\sqrt{2})bc=(2-\sqrt{2})bc\\ &\Leftrightarrow\,0=0. \end{align}$
Acknowledgment
The problem by Mehmet Sahin has been kindly posted by Dan Sitaru at the CutTheKnotMath facebook page, along with a solution of his.
|Contact| |Up| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73295009
