A Problem in a Configuration of Three Squares
What Is This About?
Source

Problem
Given a configuration of three squares $ABCD,$ $AFGE,$ $DEHI:$
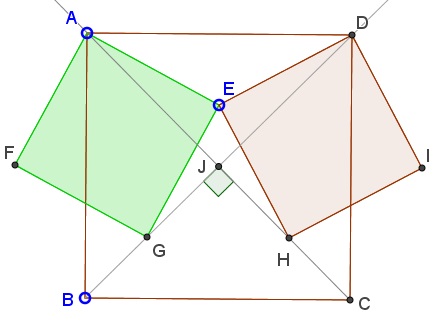
Prove that $BG\perp CH.$
Solution 1
Let $B=0,$ $C=1,$ $A=i,$ $D=1+i$ and $E=a+bi,$ where $0\lt ab\lt 1.$ We have
$\displaystyle \frac{G-E}{A-E}=i\;\Rightarrow\;G=a+b-1+(b-a)i.$
Similarly,
$\displaystyle \frac{H-E}{B-E}=-i\;\Rightarrow\; H=a-b+1+(a+b-1)i,$
But
$iG=(a-b)+(a+b-1)i\;\Rightarrow\;i(G-B)=(a-b)+(a+b-1)i=H-1=H-C.$
It follows that $\displaystyle \frac{H-C}{G-B}=i,$ so that $BG\perp CH.$
Solution 2
WLOG, let side of $ABCD$ be unity. Choose, positive $X$ and $Y$ axes along $AB$ and $AD$, respectively. Positive $Z$ points out of the plane. Let $AE=l$ and $\angle BAE=\phi$. Listing some vectors,
$\begin{align} &\vec{AD}=\vec{BC}=\hat{y} \\ &\vec{AB}=\vec{DC}=\hat{x} \\ &\vec{AE}=l\cos\phi\hat{x}+l\sin\phi\hat{y} \\ &\vec{EG}=\vec{AF}=\vec{AE}\times\hat{z} =l\sin\phi\hat{x}-l\cos\phi\hat{y} \\ &\vec{DE}=\vec{AE}-\vec{AD} =l\cos\phi\hat{x}+(l\sin\phi-1)\hat{y} \\ &\vec{EH}=\hat{z}\times\vec{DE} =-(l\sin\phi-1)\hat{x}+l\cos\phi\hat{y} \\ &\vec{CH}=-\vec{DC}+\vec{DE}+\vec{EH} =l(\cos\phi-\sin\phi)\hat{x}+(l\cos\phi+l\sin\phi-1)\hat{y} \\ &\vec{BG}=\vec{AE}+\vec{EG}-\vec{AB} =(l\cos\phi+l\sin\phi-1)\hat{x}+l(\sin\phi-\cos\phi)\hat{y} \end{align}$
Thus,
$\begin{align} &\vec{CH}\cdot\vec{BG} \\ &=l(\cos\phi-\sin\phi)(l\cos\phi+l\sin\phi-1) \\ &+l(\sin\phi-\cos\phi)(l\cos\phi+l\sin\phi-1) =0. \end{align}$
Hence, $CH$ is perpendicular to $BG$.
Acknowledgment
The problem was posted by Miguel Ochoa Sanchez at the Peru Geometrico facebook group and kindly communicated to me by Leo Giugiuc, along with a solution of his. More solutions can be found at the above link. Solution 2 is by Amit Itagi.
It is worth observing that the configuration of the three pairs of squares, each pair sharing a vertex, is a configuration rich in properties. Below are two availabe at this site.
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73301549
