Viviani's 3D Analogue
Viviani's theorem states that the sum of distances from a point within an equilateral triangle to the sides of the triangle is constant, i.e., it does not depend on position of the point.
In the diagram below, the sum of distances $d_a,$ $d_b,$ $d_c,$ is constant: $d_a +d_b +d_c =\mbox{constant}.$
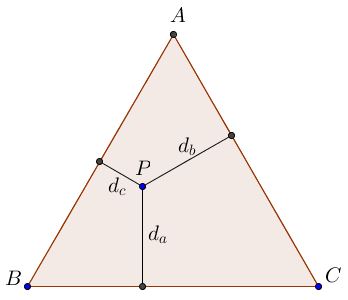
It is easy to determine the value of the constant. Join point $P$ to the vertices:

Then, square brackets $[\Delta]$ to denote the area of $\Delta,$
$\begin{align}\displaystyle [ABC] &= [BCP]+[ACP]+[ABP]\\ &=\frac{1}{2}ad_{a}+\frac{1}{2}bd_{b}+\frac{1}{2}cd_{c}\\ &=\frac{1}{2}a(d_{a}+d_{b}+d_{c})\\ &=\frac{1}{2}ah, \end{align}$
where $h$ is the altitude of $\Delta ABC.$ It follows that $d_{a}+d_{b}+d_{c}=h.$
This derivation will work in a tetrahedron with equiareal faces, for the volume of a pyramid, is $\displaystyle\frac{1}{3}hS,$ where $S$ is the area of the base and $h$ the corresponding altitude. Joining a point within a tetrahedron to the vertices splits the tetrahedron to four smaller ones whose volumes add up to the volume of the whole. If all faces have the same area then the distances $d_{a},$ $d_{b},$ $d_{c},$ $d_{d}$ from a point to the faces add up to the altitude of the tetrahedron: $d_{a}+d_{b}+d_{c}+d_{d}=h;$ incidentally we observe that in a tetrahedron with equiareal faces all four altitudes coincide. Since isosceles tetrahedron has equiareal faces, we have the following statement:
For a point in an isosceles tetrahedron the sum of distances to the faces is constant.
|Up| |Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73291951
