Uncommon Triangle Constructions
What makes the following problems of triangle construction uncommon is that some of the data includes a combination of triangle elements.
$A,a,b+c$
(Credited to John Horton Conway.)
Construct triangle $ABC,$ given $A,$ $a$ and $b+c.$
Draw $\Delta DBC$ with $\angle BDC=A/2,$ $CD=b+c,$ and $BC=a.$ Intersect the perpendicular bisector of $DB$ with $CD,$ finding $A.$
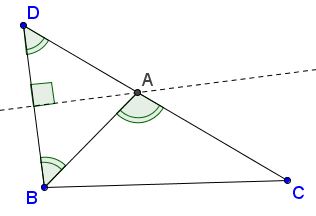
Indeed, by the construction, $\Delta DAB$ is isosceles in $A$ and $\angle CAB=A$ holds.
$A,b,a+c$
(Credited to John Horton Conway.)
Construct triangle $ABC,$ given $A,$ $b$ and $a+c.$
Draw $\Delta ACD$ with $\angle CAD=A,$ $AD=a+c,$ and $AC=b.$ Intersect the perpendicular bisector of $CD$ with $AD,$ finding $B.$
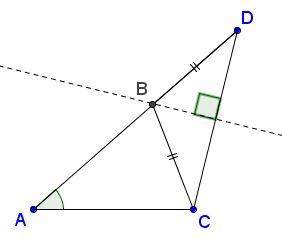
Indeed, by the construction, $\Delta CBD$ is isosceles in $B$ so that $BC+AB=a+c$ holds.
$A,a,b-c$
(Credited to John Horton Conway.)
Construct triangle $ABC,$ given $A,$ $a$ and $b-c.$
Draw $BC=a$ with $J$ on it such that $2 BJ = a-(b-c).$ On the perpendicular to $BC$ through $J$ find $I$ such that $2\angle BIC = \pi+A,$ then reflect the line $BC$ in $BI$ and $CI,$ finding $A$ at the intersection.
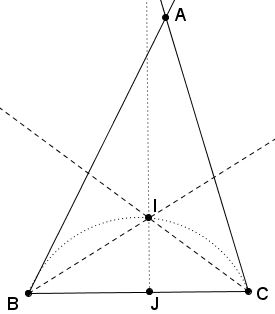
Indeed, by the construction, $\angle BCI+\angle CBI=90^{\circ}-A/2,$ so that $\angle BCA+\angle CBA=180^{\circ}-A,$ making $\angle BAC=A.$
$m_a,m_b,a/b$
(Credited to René Sperb.)
Construct triangle $ABC,$ given $m_a,$ $m_b$ and $a/b.$
We start with median $m_b,$ i.e. the line $BM_b$. Assume the ratio $a/b = \lambda.$ Then $\Delta BCM_b$ has two sides of length $a,$ $b/2$ and, therefore, a ratio $2\lambda.$ Now, it is known that the vertex $C$ must lie on the circle of Apollonius $a_p$. It can be constructed by choosing an arbitrary vertex $C'$ ,such that the sides $BC'$ and $M_bC'$ have the ratio 2$\lambda $.
Next, constructs the angle bisectors (internal and external) at $C',$ thus obtaining the points $L$ and $Q$ on $BM_b.$ The circle $a_p$ with diameter $LQ$ and center $A_p$ then is the circle of Apollonius.
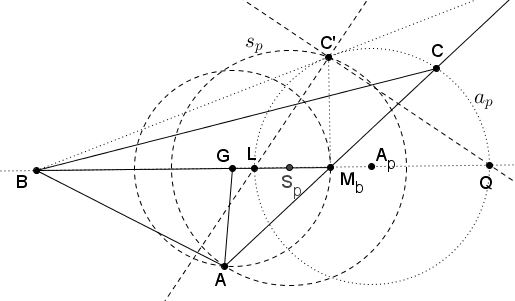
To every point $C'$ on $a_p$ corresponds a point $A'$ on the circle $s_p=(S_p)$, which is the mirror image of $a_p$ with respect to $M_b$. The circle $s_p$ is the locus of possible vertices $A$ with the property that $a/b =\lambda.$ Thus, one finds $A$ on $s_p$ at distance $\frac{2}{3}m_a$ from the centroid $G$ which, in turn, is at distance $\frac{2}{3}m_b$ from vertex $B.$ Vertex $C$ is on the point of intersection of the extension of $AM_b$ and $a_p$.
$a, A, m_{c}/m_{b}$
(Credited to René Sperb.)
Construct triangle $ABC,$ given $a,$ $A,$ $m_{c}/m_{b}.$
The barycenter $G$ of $\Delta ABC$ lies on the circle of Apollonius with ratio $m_{c}/m_{b}$ - this is circle $(KL)$ on $KL$ as a diameter. The midpoint $M_b$ of $AC$ lies, therefore, on circle $(c)$ obtained from $(KL)$ with a homothety with center $B$ and coefficient $\frac{3}{2}.$
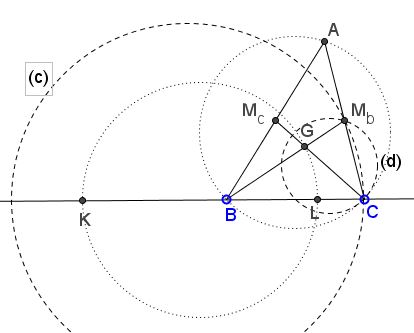
On the other hand, $M_b$ lies on the circle $(d)$ obtained from the circumcircle $(ABC)$ by a homothety centered at $C$ with coefficient $\frac{1}{2}.$ Hence, $M_b$ lies at the intersection of the two circles, $(c)$ and $(d).$ Thus be get the construction:
- Find points $K$ and $L$ that divide $BC$ externally and internally in the given ratio. Construct $(KL).$
- Construct $(c).$
- Construct circle $(ABC)$ by arbitrarily choosing angles at $B$ and $C$ whose sum supplements $A.$
- Construct circle $(d).$ At the intersection with $(c)$ (if exists) mark $M_b.$
- Line $CM_b$ meets $(ABC)$ in $A.$

|Contact| |Front page| |Contents| |Up|
Copyright © 1996-2018 Alexander Bogomolny
73325202
