An Identity in Triangle II

Here's a resized but authentic formulation of the problem by Miguel Ochoa Sanchez:

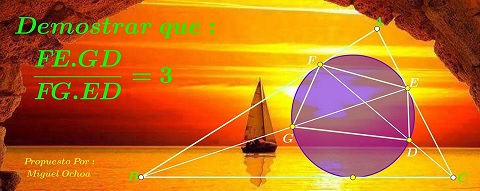
For convenience, here's a more formal version:

The incircle touches sides $AC$ and $AB$ in points $E$ and $F,$ respectively; $G$ is the intersection of $BE$ with the incircle, $D$ is the intersection of the incircle with $CF.$

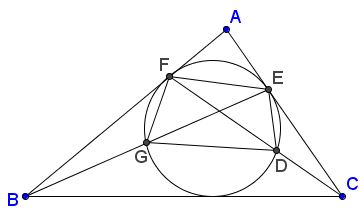
An Identity in Triangle II - problem

Prove that

$\displaystyle \frac{EF\cdot DG}{FG\cdot DE}=3.$

Proof

We denote $x=AE=AF,$ $y=BF,$ $z=CE.$ By Stewart's theorem in $\Delta ABC$ and cevian $BE,$

$BE^{2}\cdot AC+AE\cdot CE\cdot AC=AB^{2}\cdot CE+BC^{2}\cdot AE,$

or, in our notations,

$(x+z)BE^{2}+xz(x+z)=(x+y)^{2}z+(y+z)^{2}x,$

which leads to an expression of $BE$ in terms of $x,y,z:$

$\displaystyle BE=\sqrt{\frac{y(yz+xy+4xz)}{x+z}}.$

Similarly,

$\displaystyle CF=\sqrt{\frac{z(yz+4xy+xz)}{x+y}}.$

By the Power of a point theorem, $BG\cdot BE=y^{2}.$ Hence,

$\displaystyle \frac{BG}{BE}=\frac{y(x+z)}{yz+xy+4xz}$

such that

$\displaystyle \frac{EG}{BE}=\frac{4xz}{yz+xy+4xz}.$

Similarly,

$\displaystyle\frac{DF}{CF}=\frac{4xy}{yz+4xy+xz}.$

Next, in $\Delta AEF,$ $\displaystyle EF=2x\sin\frac{A}{2}=2x\sqrt{\frac{yz}{(x+y)(x+z)}}.$ By Stewart's theorem, in $\Delta FBE$ for cevian $FG,$ we obtain

$FG^{2}\cdot BE+EG\cdot BG\cdot BE=BF^{2}\cdot EG+EF^{2}\cdot BG,$

meaning

$\displaystyle FG^{2}\cdot BE+EG\cdot y^{2}=y^{2}\cdot EG+4x^{2}\frac{yz}{(x+y)(x+z)}\frac{y(x+z)}{yz+xy+4xz}\cdot BE,$

so that

$\displaystyle FG=2xy\sqrt{\frac{z}{(x+y)(yz+xy+4xz)}}.$

Similarly,

$\displaystyle DE=2xz\sqrt{\frac{y}{(x+z)(yz+4xy+xz)}}.$

By Ptolemy's theorem in $FGDE,$ $EG\cdot DF=EF\cdot DG+FG\cdot DE.$ Here

$\displaystyle\begin{align} EG\cdot DF&=\frac{4xz}{yz+xy+4xz}\sqrt{\frac{y(yz+xy+4xz)}{x+z}}\cdot \frac{4xy}{yz+4xy+xz}\sqrt{\frac{z(yz+4xy+xz)}{x+y}}\\ &=16x^{2}yz\sqrt{\frac{yz}{(x+y)(x+z)(yz+xy+4xz)(yz+4xy+xz)}}, \end{align}$

while

$\displaystyle FG\cdot DE=4x^{2}yz\sqrt{\frac{yz}{(x+y)(x+z)(yz+xy+4xz)(yz+4xy+xz)}},$

implying

$\displaystyle EF\cdot DG=12x^{2}yz\sqrt{\frac{yz}{(x+y)(x+z)(yz+xy+4xz)(yz+4xy+xz)}}=3FG\cdot DE,$

as required.

Acknowledgment

The problem with solution has been posted by Leo Giugiuc at the CutTheKnotMath facebook page. The identity is the discovery of Miguel Ochoa Sanchez.

71081226