Equilateral Triangle on Angle Bisectors
In $\Delta ABC$ points $A_1,\,B_1,\,C_1$ lie on the angle bisectors $AI,\,BI,\,CI,$ where $I$ is the incenter, and satisfy
$\begin{align}\displaystyle AA_{1}&=\bigg(\frac{b+c-a}{2}-\frac{r}{\sqrt{3}}\bigg)\cos\frac{A}{2}\\ BB_{1}&=\bigg(\frac{a+c-b}{2}-\frac{r}{\sqrt{3}}\bigg)\cos\frac{B}{2}\\ CC_{1}&=\bigg(\frac{a+b-c}{2}-\frac{r}{\sqrt{3}}\bigg)\cos\frac{C}{2}\\ \end{align}$
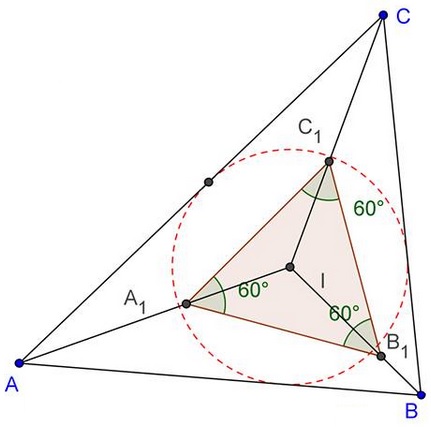
Prove that $\Delta A_{1}B_{1}C_{1}$ is equilateral.
Proof
We know that (with $\displaystyle p=\frac{a+b+c}{2}$ the semiperimeter of $\Delta ABC$ and $[ABC]$ its area)
$\displaystyle AI=\frac{bc}{p}\cos\frac{A}{2}=\frac{2[ABC]}{p\sin A}=\frac{r}{\sin\frac{A}{2}}. $
Also, $\displaystyle p-a=r\cot\frac{A}{2}.$ Using that,
$\begin{align}\displaystyle IA_{1} &= \frac{r}{\sin\frac{A}{2}}-r\cot\frac{A}{2}+\frac{r}{\sqrt{3}}\cdot\cos\frac{A}{2}\\ &=r\cdot\sin\frac{A}{2}+\frac{r}{\sqrt{3}}\cdot\cos\frac{A}{2}\\ &=\frac{2r}{\sqrt{3}}\sin\bigg(\frac{A}{2}+\frac{\pi}{6}\bigg). \end{align}$
Similarly,
$\begin{align}\displaystyle IB_{1} &= \frac{2r}{\sqrt{3}}\sin\bigg(\frac{B}{2}+\frac{\pi}{6}\bigg),\\ IC_{1} &= \frac{2r}{\sqrt{3}}\sin\bigg(\frac{C}{2}+\frac{\pi}{6}\bigg). \end{align}$
Denote for simplicity $\displaystyle u=\frac{A}{2}+\frac{\pi}{6},$ $\displaystyle v=\frac{B}{2}+\frac{\pi}{6},$ $\displaystyle w=\frac{C}{2}+\frac{\pi}{6},$ so that, $u,v,w\gt 0$ and $u+v+w=\pi.$ Observe that $\displaystyle \angle BIC=\frac{\pi +A}{2}=u+\frac{\pi}{3},$ $\displaystyle \angle AIC=v+\frac{\pi}{3},$ and $\displaystyle \angle AIB=w+\frac{\pi}{3}.$
Define complex numbers $m=\sin u,$ $\displaystyle n=\sin v\cdot e^{(w+{\pi}/{3})i},$ $\displaystyle p=\sin w\cdot e^{(u+w+{2\pi}/{3})i}.$ Suffice it to show that, for $\epsilon =e^{{2\pi i}/{3}},$ $P(\epsilon )=m+n\epsilon +p\epsilon^{2}=0.$ Substituting and using the addition and subtraction formulas for sine and cosine,
$\begin{align}\displaystyle P(\epsilon )&=\sin u+\sin v\cdot e^{(w+\pi )i}+\sin w\cdot e^{(u+w)i}\\ &=[\sin u-\sin v\cdot\cos w+\sin w\cdot\cos (u+w)]\\ &\,\,\,+[-\sin v\cdot\sin w+\sin w\cdot\sin (u+w)]i=0, \end{align}$
because $u+v+w=\pi.$
Acknowledgment
The problem has been posted by Dao Thanh Oai (Vietnam) at CutTheKnotMath facebook page; the solution is due to Leo Giugiuc (Romania). Leo stipulates that the above derivation is only valid when all angles in $\Delta ABC$ are less than $120^{\circ}.$ This is because the quantities $AA_1,$ $BB_1,$ $CC_1$ have been implicitly assumed to be positive. To see when this is so, consider, for example $\displaystyle AA_{1}=\bigg(\frac{b+c-a}{2}-\frac{r}{\sqrt{3}}\bigg)\cos\frac{A}{2}.$ The second factor is always positive. For the first factor to be positive we need $\displaystyle r\cot\frac{A}{2}\gt\frac{r}{\sqrt{3}},$ or, $\displaystyle\tan\frac{A}{2}\lt\sqrt{3},$ which is equivalent to $\displaystyle\frac{A}{2}\lt\frac{\pi}{3},$ i.e., $\displaystyle A\lt\frac{2\pi}{3}.$
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73298444
