Perspective Triangles in Circle
Given $\Delta ABC,$ its circumcircle $\omega,$ and point $K$ inside the triangle. $AK,$ $BK,$ $CK$ cut $\omega$ in $D,$ $E,$ and $F,$ respectively.
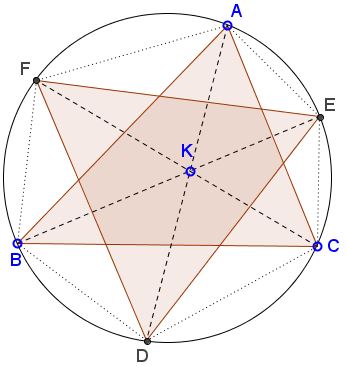
Prove that
$\displaystyle\frac{BD\times CE}{BC\times DE}+\frac{CE\times AF}{AC\times EF}+\frac{AF\times BD}{AB\times DF}=1.$
Proof
Let, as usual, $a=BC,\,b=AC,\,c=AB.$ For the areas, set $x=[\Delta BCK],$ $y=[\Delta ACK],$ $z=[\Delta ABK].$ Define also, $M$ as the intersection of $AK$ and $BC,$ $N$ the intersection of $BK$ and $AC,$ $P$ the intersection of $CK$ and $AB.$
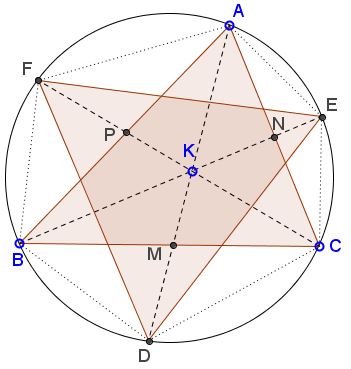
Then $\displaystyle\frac{BM}{CM}=\frac{z}{y}$ such that $\displaystyle BM=\frac{az}{y+z}$ and $\displaystyle CM=\frac{ay}{y+z}.$ Similarly, $\displaystyle CN=\frac{bx}{x+z}$ and $\displaystyle AN=\frac{bz}{x+z}.$
By the Intersecting chords theorem, $DM\cdot AM=BM\cdot CM,$ implying
$\displaystyle DM=\frac{a^{2}yz}{AM(y+z)^2}.$
Now, from the similarity of triangles $DEK$ and $BAK,$ $\frac{DE}{AB}=\frac{DK}{BK},$ with $DK=KM+DM.$
By van Aubel's theorem, $\displaystyle\frac{AK}{KM}=\frac{y+z}{x}$ so that $\displaystyle KM=\frac{x\cdot AM}{x+y+z}$ and, similarly, $\displaystyle BK=\frac{(x+z) BN}{x+y+z}.$ It follows that
$\displaystyle\begin{align} DK&=\frac{a^2yz}{AM(y+z)^2}+\frac{x\cdot AM}{x+y+z}\\ &=\frac{AM^2x(y+z)^2+a^2yz(x+y+z)}{AM(y+z)^2(x+y+z).} \end{align}$
By Stewart's theorem in $\Delta ABC$ and cevian $AM,$
$AM^2\cdot BC+BM\cdot CM\cdot BC=AC^2\cdot BM+AB^2\cdot CM,$
yielding $\displaystyle AM^2=\frac{b^2z^2+c^2y^2+yz(b^2+c^2-a^2)}{AM(y+z)^2(x+y+z)},$ such that
$\displaystyle\begin{align} DK&=\frac{b^2xz(y+z)+c^2xy(y+z)+a^2yz(y+z)}{AM(y+z)^2(x+y+z)}\\ &=\frac{a^2yz+b^2xz+c^2xy}{AM(y+z)(x+y+z)} \end{align}$
from which
$\displaystyle\begin{align} DE&=c\frac{a^2yz+b^2xz+c^2xy}{AM(y+z)(x+y+z)}\cdot\frac{x+y+z}{(x+z)BN}\\ &=c\frac{a^2yz+b^2xz+c^2xy}{AM\cdot BN(x+z)(y+z)}. \end{align}$
Hence,
$\displaystyle BD\times CE=\frac{ab^2cxz}{AM\cdot(x+z)(y+z)}$ and $\displaystyle BC\times DE=ac\frac{a^2yz+b^2xz+c^2xy}{AM\cdot(x+z)(y+z)}$
and, finally,
$\displaystyle\frac{BD\times CE}{BC\times DE}=\frac{b^2xz}{a^2yz+b^2xz+c^2xy}.$
The other two fractions are found similarly and are immediately seen to add up to $1.$
Acknowledgment
The problem proposed by Van Khea (May 2, 2015) was communicated to me - along with the solution - by Leo Giugiuc.
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73296851
