The Law of Cosines for 60° and 120°
In the spirit of Proof #3 of the Pythagorean theorem, we show that
In a triangle $ABC,\;$ with sides $a=BC,\;$ $b=AC,\;$ $c=AB,\;$ and $\gamma=\angle ACB,\;$ we have
$c^2=a^2+b^2-ab\;$ if $\gamma=60^{\circ}\;$ and
$c^2=a^2+b^2+ab\;$ if $\gamma=120^{\circ}\;$
These are clearly specific cases of the Law of Cosines. Observe, however, that in the proofs below neither the Law of Cosines nor the Pythagorean theorem are actually used.
Lemma
If the area of an isosceles triangle with the sides of length $1\;$ and the apex angle $\gamma\;$ equals $g,\;$ then the area of $\Delta ABC\;$ equals $gab.$
Indeed, by first extending side $BC\;$ we get a triangle with the area of $ga.\;$
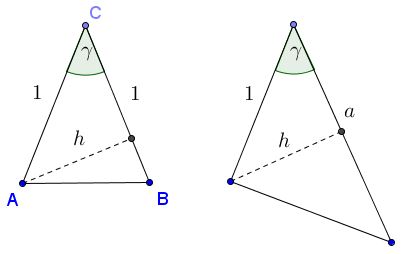
Next, extending side $AC\;$ we get a triangle with the area of $gab.\;$

Proof of Statement for $\gamma =60^{\circ}.$
Arrange three copies of the $60\text{-degree}\;$ triangle with sides $a,b,c$ into an equilateral triangle:
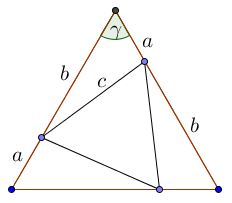
The figure consists of two equilateral triangles - big with side $a+b,\;$ and small with side $c,\;$ and three equal triangles. Taking $\gamma=60^{\circ},\;$ gives
$g(a+b)^2 = gc^2+3gab,$
or, $a^2+b^2-ab=c^2.$
Proof of Statement for $\gamma =120^{\circ}.$
Now, arranging the triangle into a regular hexagon:

we get, as before, $6(a+b)^2=6ab+6c^2,\;$ because a regular hexagon is the union of six equilateral triangles. Simplifying we get $a^2+b^2+ab=c^2.$
Reference
- B. Polster, M. Ross, One-Glance(ish) Proofs of Pythagorasí Theorem for 60-Degree and 120-Degree Triangles, Math Magazine, VOL. 89, NO. 1, FEBRUARY 2016 47-54
![]()
|Contact| |Front page| |Contents| |Geometry|
Copyright © 1996-2018 Alexander Bogomolny73296626
